Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles. Trigonometric functions such as sine, cosine, and tangent are essential tools in solving trigonometric problems. The double angle formulas are an important concept in trigonometry that allows us to express the trigonometric functions of twice an angle in terms of the functions of the angle itself. These formulas play a crucial role in simplifying trigonometric equations and finding solutions in geometry and physics.
What is the Double Angle Formula?
The double angle formulas are trigonometric identities that relate the trigonometric functions of double angles to the functions of the original angles. These formulas are derived from the sum formulas of trigonometry and are used to simplify trigonometric expressions and solve trigonometric equations. The double angle formulas for sine, cosine, and tangent are as follows:
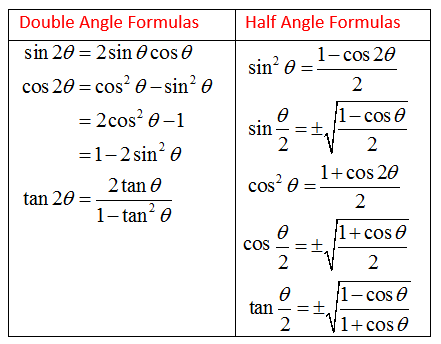
Sine Double Angle Formula: sin(2θ) = 2sin(θ)cos(θ)
Cosine Double Angle Formula: cos(2θ) = cos^2(θ) – sin^2(θ)
Tangent Double Angle Formula: tan(2θ) = 2tan(θ) / (1 – tan^2(θ))
These formulas allow us to express the trigonometric functions of double angles in terms of the functions of the original angles, which can be very useful in various mathematical and engineering applications.
Double Angle Formulas
Double Angle Formulas of Sin
The double angle formula of sine is given by sin(2θ) = 2sin(θ)cos(θ). This formula relates the sine of twice an angle to the product of the sine and cosine of the angle itself. It is derived from the sum-to-product identity for sine, which states that sin(A + B) = sin(A)cos(B) + cos(A)sin(B). By substituting A = B = θ in this identity, we obtain the double angle formula for sine.
Double Angle Formulas of Cos
The double angle formula of cosine can be expressed in multiple ways. The first form is cos(2θ) = cos^2(θ) – sin^2(θ). This formula is derived from the sum-to-product identity for cosine, which states that cos(A + B) = cos(A)cos(B) – sin(A)sin(B). By substituting A = B = θ in this identity, we obtain the double angle formula for cosine.
Another form of the cosine double angle formula is cos(2θ) = 2cos^2(θ) – 1. This can be derived by using the identity cos^2(θ) = 1 – sin^2(θ) and substituting it into the first form of the formula.
The third form of the cosine double angle formula is cos(2θ) = 1 – 2sin^2(θ). This form is derived by using the identity sin^2(θ) = 1 – cos^2(θ) and substituting it into the first form of the formula.
Double Angle Formulas of Tan
The double angle formula of tangent is given by tan(2θ) = 2tan(θ) / (1 – tan^2(θ)). This formula relates the tangent of twice an angle to the product of the tangent of the angle itself and a denominator term. It is derived from the tangent sum of angles formula, which states that tan(A + B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B)). By substituting A = B = θ in this identity, we obtain the double angle formula for tangent.
Double Angle Formulas Derivation
The double angle formulas can be derived from the sum formulas of trigonometry and the Pythagorean identities. Let’s go through the derivation of each double angle formula step by step.
Derivation of Sine Double Angle Formula
The sum formula for the sine function is sin(A + B) = sin(A)cos(B) + cos(A)sin(B). When A = B, the sum formula becomes sin(2θ) = sin(θ)cos(θ) + cos(θ)sin(θ) = 2sin(θ)cos(θ). This is the double angle formula for sine.
Derivation of Cosine Double Angle Formula
The sum formula for the cosine function is cos(A + B) = cos(A)cos(B) – sin(A)sin(B). When A = B, the sum formula becomes cos(2θ) = cos^2(θ) – sin^2(θ). This is one form of the double angle formula for cosine.
To derive the other forms of the cosine double angle formula, we can use the Pythagorean identities sin^2(θ) + cos^2(θ) = 1 and cos^2(θ) = 1 – sin^2(θ) to rewrite the formula.
By substituting 1 – sin^2(θ) for cos^2(θ) in the first form of the formula, we get cos(2θ) = 2cos^2(θ) – 1.
By substituting 1 – cos^2(θ) for sin^2(θ) in the first form of the formula, we get cos(2θ) = 1 – 2sin^2(θ).
Derivation of Tangent Double Angle Formula
The sum formula for the tangent function is tan(A + B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B)). When A = B, the sum formula becomes tan(2θ) = (tan(θ) + tan(θ)) / (1 – tan(θ)tan(θ)) = 2tan(θ) / (1 – tan^2(θ)). This is the double angle formula for tangent.
Double Angle Identities
Double angle identities are trigonometric equations that relate the trigonometric functions of twice an angle to the functions of the original angle. The double angle formulas we derived earlier are examples of double angle identities. These identities are fundamental tools in trigonometry and are used to simplify trigonometric expressions and solve trigonometric equations.
Half Angle Formulas
Half angle formulas are trigonometric equations that express the trigonometric functions of half an angle in terms of the functions of the original angle. These formulas are derived from the double angle formulas and are used to simplify trigonometric expressions and solve trigonometric equations. The half angle formulas for sine, cosine, and tangent are as follows:
Sine Half Angle Formula: sin(θ/2) = ±√[(1 – cos(θ)) / 2]
Cosine Half Angle Formula: cos(θ/2) = ±√[(1 + cos(θ)) / 2]
Tangent Half Angle Formula: tan(θ/2) = ±√[(1 – cos(θ)) / (1 + cos(θ))]
The ± sign indicates that the formulas have two possible solutions, one positive and one negative, depending on the quadrant in which the angle lies.
Solved Examples on Double Angle Formulas
Let’s solve some examples using the double angle formulas to better understand their application.
Example 1: Find the value of sin(2θ) if sin(θ) = 3/5.
Solution: Given sin(θ) = 3/5 Using the double angle formula for sine, sin(2θ) = 2sin(θ)cos(θ), we can substitute sin(θ) = 3/5. sin(2θ) = 2(3/5)cos(θ) sin(2θ) = (6/5)cos(θ)
Example 2: Express cos(2α) in terms of cos(α) if cos(α) = -4/7.
Solution: Given cos(α) = -4/7 Using the double angle formula for cosine, cos(2α) = cos^2(α) – sin^2(α). We can substitute cos(α) = -4/7 and use the Pythagorean identity sin^2(α) = 1 – cos^2(α). cos(2α) = (-4/7)^2 – (1 – (-4/7)^2) cos(2α) = 16/49 – (1 – 16/49) cos(2α) = 16/49 – (49/49 – 16/49) cos(2α) = 16/49 – 33/49 + 16/49 cos(2α) = -1/49
Example 3: Find the value of tan(2θ) if tan(θ) = 125.
Solution: Given tan(θ) = 125 Using the double angle formula for tangent, tan(2θ) = 2tan(θ) / (1 – tan^2(θ)), we can substitute tan(θ) = 125. tan(2θ) = 2(125) / (1 – 125^2) tan(2θ) = 250 / (1 – 15625) tan(2θ) = 250 / (-15624) tan(2θ) ≈ -0.015997
These examples demonstrate how the double angle formulas can be used to find the trigonometric values of double angles given the values of the original angles.
How Kunduz Can Help You Learn Double Angle Formulas?
Our platform provides a user-friendly interface and engaging content that caters to students of all levels, from beginners to advanced learners. Whether you’re studying for a math exam or looking to enhance your problem-solving skills, Kunduz is here to support you every step of the way.
