Exponential growth and decay are fundamental concepts in mathematics that describe the rapid change in the value or form of a physical quantity over time. These concepts find applications across various fields, including biology, finance, physics, and computer science. In this article, we will explore the definition of exponential growth and decay, understand the formulas involved, examine their applications, and solve examples to gain a deeper understanding of these concepts. So, let’s dive in!
An Introduction to Exponential Growth
Exponential growth refers to a pattern of growth where a quantity steadily increases at an ever-accelerating rate. It occurs when the rate of change of a quantity with respect to time is proportional to the quantity itself. In simpler terms, the more the quantity grows, the faster it grows. This leads to a curved graph that shows a steep increase over time.
On the other hand, exponential decay is the opposite of exponential growth. It describes a pattern where a quantity decreases rapidly over time. The rate of decrease is also proportional to the quantity itself, resulting in a graph that shows a steep decline.
What is Exponential Growth?
Exponential growth is characterized by a continuous increase in a quantity over time at an accelerating rate. It can be observed in various real-life scenarios, such as population growth, compound interest, and the spread of viral infections. The growth is represented by an exponential function, where the variable representing time is the exponent.
The general formula for exponential growth is given by:
f(x) = ab^x
Here, ‘a’ represents the initial value of the quantity, ‘b’ is the growth factor, and ‘x’ represents the time. The growth factor ‘b’ is greater than 1, indicating that the quantity is increasing exponentially.
Exponential Growth Formula
There are several formulas used to calculate exponential growth, depending on the specific context. Here are a few commonly used formulas:
- Exponential Growth Formula: f(x) = ab^x
- ‘a’ represents the initial value of the quantity
- ‘b’ is the growth factor, which is greater than 1
- ‘x’ represents the time
- Exponential Growth Formula (Alternate): f(x) = a(1 + r)^x
- ‘a’ represents the initial value of the quantity
- ‘r’ is the rate of growth, expressed as a decimal
- ‘x’ represents the time
- Exponential Growth Formula (Continuous): P = P₀e^(kt)
- ‘P’ represents the final value of the quantity
- ‘P₀’ is the initial value of the quantity
- ‘e’ is the base of the natural logarithm, approximately equal to 2.71828
- ‘k’ is the constant of proportionality
- ‘t’ represents the time
These formulas provide different ways to calculate the growth of a quantity over time, depending on the given information and context.
Decay Function
Exponential decay is the inverse of exponential growth. It describes a pattern where a quantity decreases rapidly over time. The rate of decrease is proportional to the quantity itself. The general formula for exponential decay is:
f(x) = ab^(-x)
Here, ‘a’ represents the initial value of the quantity, ‘b’ is the decay factor, and ‘x’ represents the time. The decay factor ‘b’ is less than 1, indicating that the quantity is decreasing exponentially.
Exponential Growth and Decay
Exponential growth and decay are two sides of the same coin. While exponential growth refers to the rapid increase in a quantity over time, exponential decay describes the rapid decrease in a quantity over time. Both concepts have various applications in different fields. Let’s explore a few of these applications:
Bacterial Growth
Exponential growth plays a crucial role in studying bacterial growth. Bacteria reproduce by dividing into two cells, which then divide into four, eight, sixteen, and so on. This exponential growth pattern allows bacteria populations to increase rapidly under favorable conditions. Understanding bacterial growth is essential in fields such as medicine and microbiology.
Nuclear Chain Reactions
Nuclear chain reactions, such as those occurring in nuclear reactors and nuclear weapons, are examples of exponential growth. In a nuclear chain reaction, each fission event releases multiple neutrons, which can cause neighboring atoms to undergo fission. If the number of neutrons produced in each fission event exceeds the number of neutrons lost or absorbed, the chain reaction can grow exponentially, resulting in a significant release of energy.
Feedback
The concept of feedback often exhibits exponential growth, particularly in the context of customer feedback. Negative feedback, in particular, can spread rapidly through word-of-mouth or online platforms. If one customer has a negative experience and shares it with others, those individuals may also share the negative feedback with their network, leading to an exponential increase in the dissemination of negative feedback.
Processing Power of Computers
The processing and storage power of computers have experienced exponential growth over the years. Technological advancements have led to a significant increase in computing capabilities, with processing speeds and storage capacities growing exponentially. This growth has revolutionized various industries, enabling complex calculations, data analysis, and the development of cutting-edge technologies.
Food Degradation
Food degradation can be viewed as a case of exponential decay. When food is exposed to external factors such as air, heat, and moisture, it undergoes a process of decay. Initially, the decay is slow, but over time, it accelerates rapidly until the food becomes spoiled or inedible. This exponential decay process is the reason for expiration dates on food products.
Aging of Human Beings
The aging process in humans and other living organisms can be described as exponential decay. As individuals age, their physical and cognitive abilities gradually decline. However, the rate of decline increases exponentially in later stages of life. The aging process can be influenced by various factors, such as genetics, lifestyle choices, and underlying health conditions.
Internet Content
With the advent of the internet, the growth of online content has been exponential. People can easily create and share content, leading to an exponential increase in the amount of information available online. The rise of social media platforms and user-generated content has further fueled this exponential growth, allowing information to spread rapidly across networks.
These are just a few examples of how exponential growth and decay play a role in different fields. The concepts of exponential growth and decay provide valuable insights into various natural and man-made phenomena, helping us understand and analyze their behavior.
Exponential Growth Model
The exponential growth model is a mathematical representation of how a quantity grows exponentially over time. It follows a specific pattern, where the growth rate is proportional to the quantity itself. The exponential growth model is expressed using an exponential function of time, with the variable representing time as the exponent.
The general form of the exponential growth model is given by:
f(x) = ab^x
In this equation, ‘a’ represents the initial value of the quantity, ‘b’ is the growth factor, and ‘x’ represents the time. The growth factor ‘b’ is greater than 1, indicating that the quantity is growing exponentially. As ‘x’ increases, the value of ‘f(x)’ increases at an ever-accelerating rate.
The exponential growth model is widely applicable across various fields, including biology, finance, physics, and computer science. It provides a valuable tool for analyzing and predicting the growth of quantities over time.
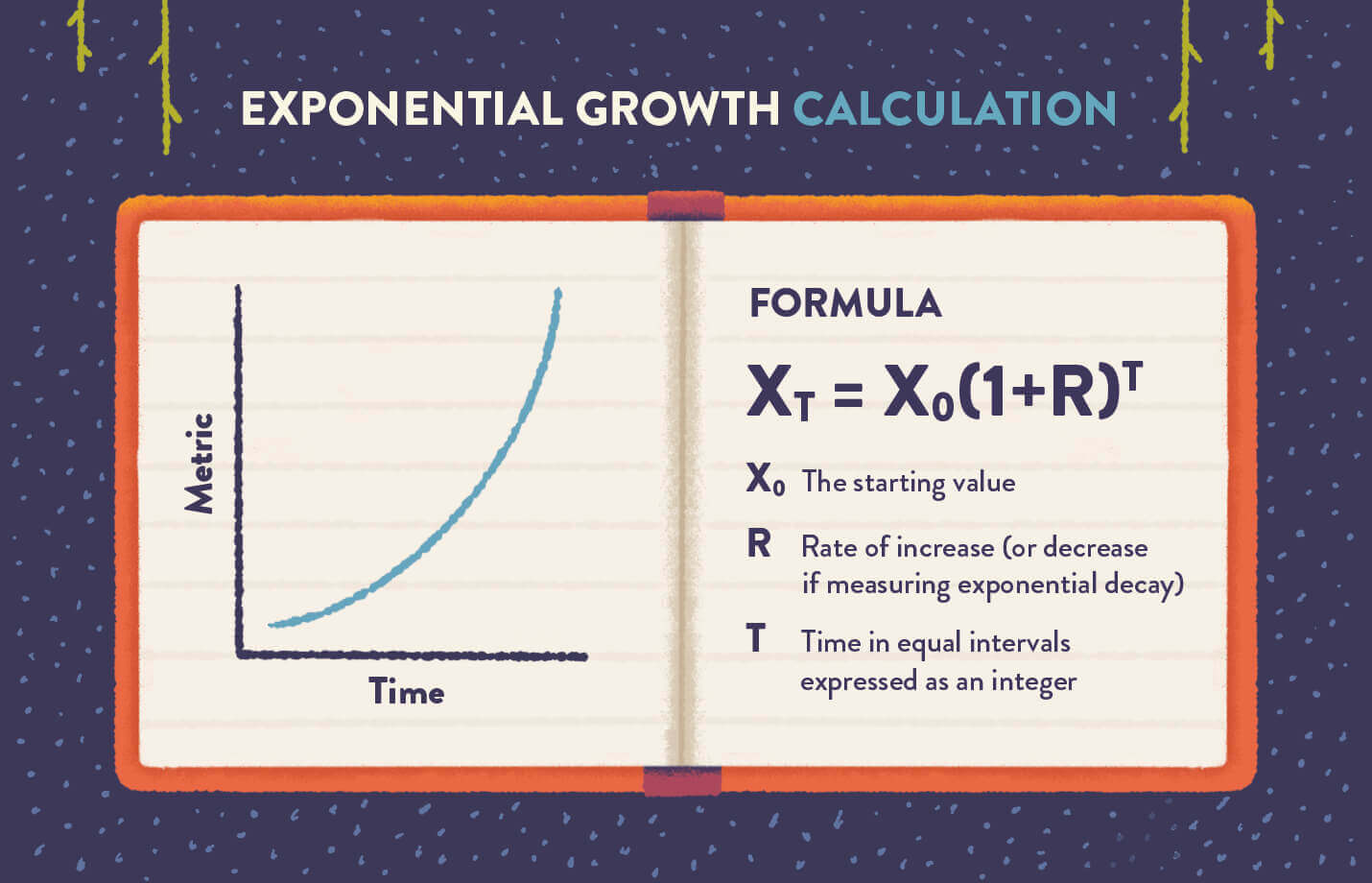
How To Calculate Exponential Growth?
Calculating exponential growth involves applying the appropriate formula and plugging in the given values. The specific formula used depends on the context and the information available. Here’s a step-by-step guide on how to calculate exponential growth:
Step 1: Determine the initial value (a) and the growth factor (b or r) from the given information.
Step 2: Identify the time period (x or t) for which you want to calculate the growth.
Step 3: Choose the appropriate formula based on the context and the available data.
Step 4: Plug in the values of the initial value (a), growth factor (b or r), and time period (x or t) into the formula.
Step 5: Evaluate the equation to calculate the growth of the quantity at the given time period.
Step 6: Round the result to the desired level of precision, if necessary.
By following these steps and using the appropriate formula, you can calculate the exponential growth of a quantity over time.
Understanding the Exponential Growth and Decay Graph
Graphs are a visual representation of mathematical functions and provide a way to understand and analyze the behavior of quantities over time. The graph of exponential growth and decay exhibits distinct characteristics that can help us gain insights into the nature of the quantity being studied.
In the case of exponential growth, the graph starts with a gradual increase and then rapidly becomes steeper. This indicates that the quantity is growing at an ever-accelerating rate. The graph appears as a curve that continually rises, reflecting the exponential nature of the growth.
On the other hand, exponential decay is represented by a graph that starts with a steep decline and then gradually levels off. The rate of decrease slows down over time, leading to a curve that flattens out. This reflects the diminishing rate of decay as the quantity approaches its lower limit.
The shape of the exponential growth and decay graph is influenced by factors such as the initial value, the growth or decay rate, and the time period under consideration. By analyzing the graph, we can observe the trend of the quantity and make predictions about its future behavior.
Frequently Asked Questions on Exponential Growth
What is the decay rate of an exponential function?
The decay rate of an exponential function is determined by the growth factor (b) in the exponential decay formula. If the growth factor is less than 1, the quantity is decreasing, and the decay rate is proportional to the growth factor.
Can exponential growth ever slow down?
Exponential growth can slow down over time due to external factors or limitations imposed by the environment. While exponential growth starts with a rapid increase, it may eventually reach a point where it levels off or transitions into a different growth pattern, such as logistic growth.
Solved Examples on Exponential Growth
Example 1: A population of bacteria doubles every 10 minutes. If there are initially 100 bacteria, how many bacteria will be present after 1 hour?
Solution: In this case, the initial value (a) is 100 and the growth factor (b) is 2. We need to calculate the growth after 1 hour, which is equivalent to 60 minutes.
Using the exponential growth formula f(x) = ab^x, we can calculate the number of bacteria:
f(60) = 100 * 2^(60/10)
= 100 * 2^6
= 100 * 64
= 6400
Therefore, after 1 hour, there will be 6400 bacteria present.
Example 2: The value of a car depreciates by 15% each year. If a car is initially worth $20,000, what will be its value after 5 years?
Solution: In this case, the initial value (a) is $20,000 and the decay rate (r) is 15% or 0.15. We need to calculate the value after 5 years.
Using the exponential decay formula f(x) = a(1 - r)^x, we can calculate the value of the car:
f(5) = 20000 * (1 - 0.15)^5
= 20000 * 0.85^5
≈ 20000 * 0.4437053125
≈ $8874.11
Therefore, after 5 years, the value of the car will be approximately $8874.11.
These examples demonstrate how the exponential growth and decay formulas can be used to calculate the growth or decay of quantities over time.
How Kunduz Can Help You Learn Exponential Growth?
At Kunduz, we understand the importance of mastering mathematical concepts like exponential growth. Our experienced tutors are skilled in breaking down complex topics into easily understandable lessons. Whether you need help with understanding the formulas, solving problems, or gaining a deeper understanding of the underlying principles, our tutors are here to guide you.
