In the world of calculus, there are two fundamental concepts: differentiation and integration. While differentiation involves finding the derivative of a function, integration is the reverse process. It allows us to determine the original function when given its derivative. Integration is a powerful tool that has applications in various areas of mathematics and real-world scenarios.
What is Integral?
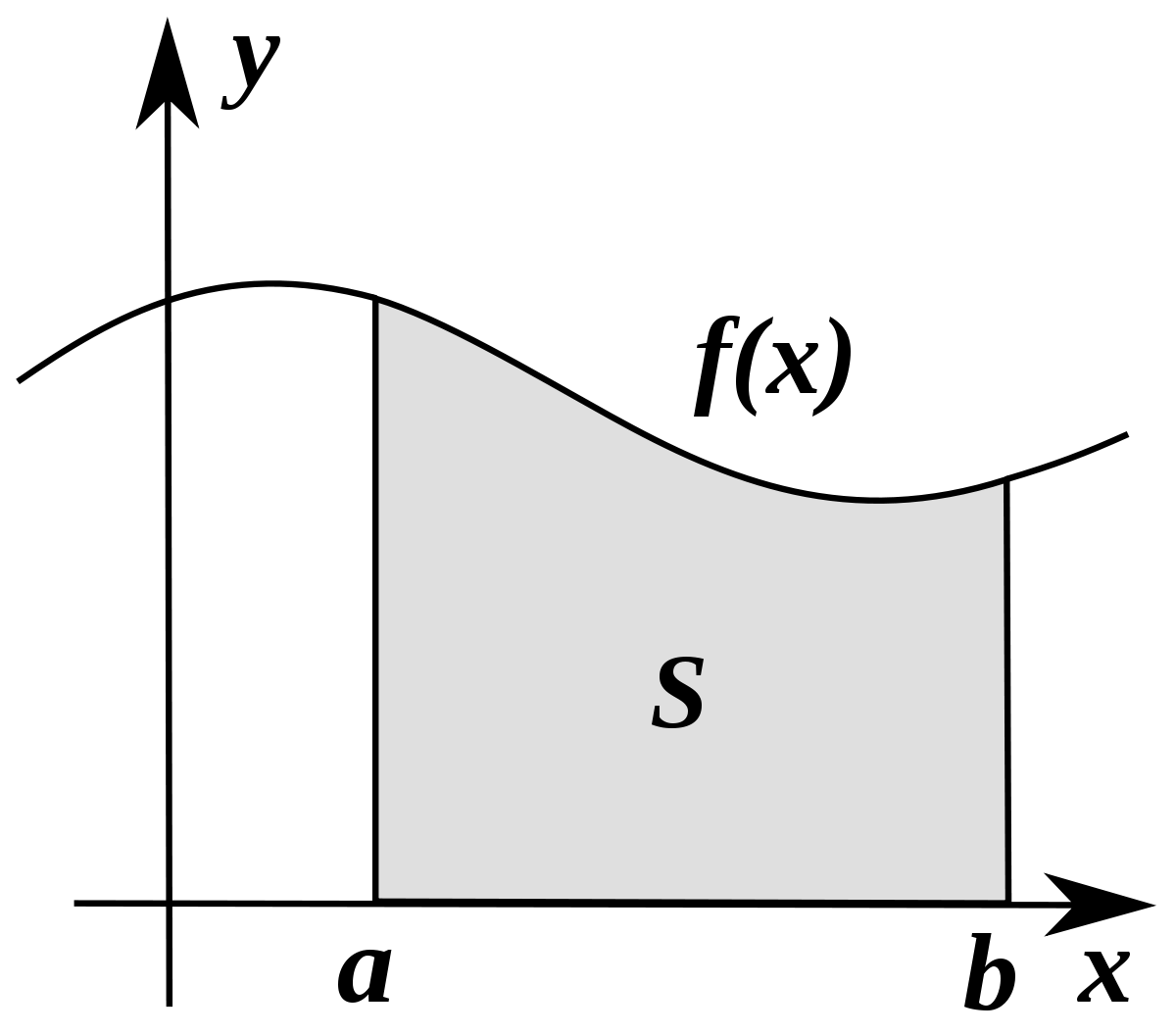
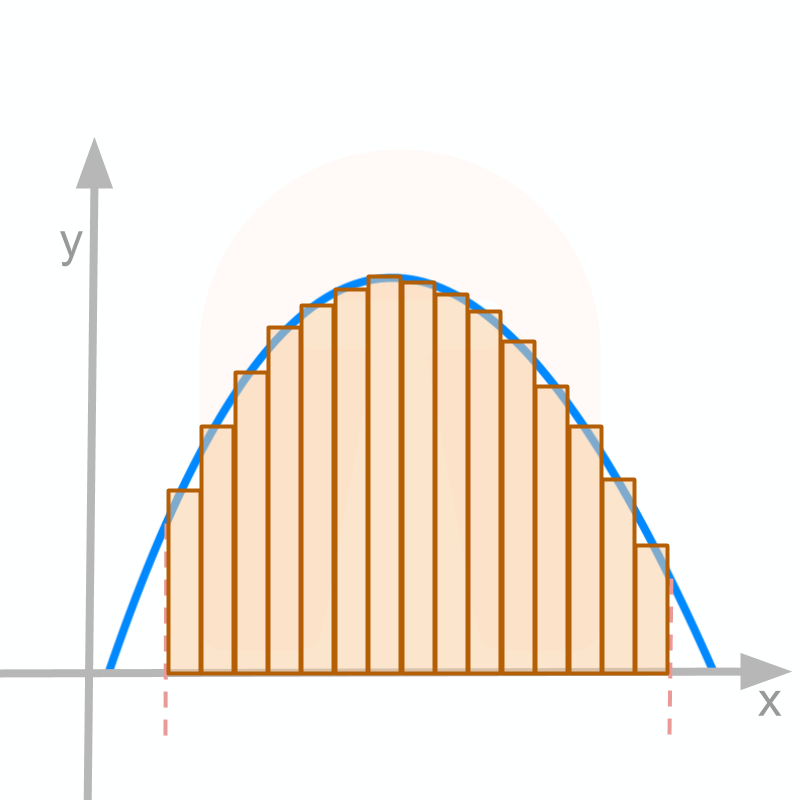
At its core, integration is a mathematical operation that deals with finding the area under a curve. It involves dividing the area into infinitesimally small rectangles and summing them up. The result is a numerical value that represents the accumulated area.
What is Indefinite Integral?
Indefinite integral, also known as antiderivative, is a type of integration that does not have any specific upper or lower limits. It focuses on finding the general solution to the integration problem. The indefinite integral of a function f(x) is denoted by ∫f(x)dx and represents a family of functions that have the same derivative as f(x).
Symbol/Term/Meaning
To better understand the concept of indefinite integral, let’s take a look at some important symbols, terms, and their meanings:
| Symbol/Term/Phrase | Meaning |
|---|---|
| ∫ f(x) dx | Integral of f with respect to x |
| f(x) in ∫ f(x) dx | Integrand |
| x in ∫ f(x) dx | Variable of integration |
| Integral of f | A function F such that F'(x) = f(x) |
| Integration | The process of finding the integral |
| Constant of Integration | Any real number C, considered as a constant function |
Reverse Power Rule
The reverse power rule is a fundamental concept in indefinite integration. It allows us to find the integral of functions with terms of the form xn. The reverse power rule states that:
∫x^n dx = (x^(n+1))/(n+1) + C, where n ≠ -1
This rule is applicable to functions where the exponent of the variable is increased by 1, and the result is divided by the new exponent value.
Indefinite Integral Formulas
There are several important indefinite integral formulas that are commonly used in calculus. These formulas provide a systematic way to evaluate integrals. Here are some of the most frequently used formulas:
- ∫1 dx = x + C
- ∫x^n dx = (x^(n+1))/(n+1) + C, where n ≠ -1
- ∫e^x dx = e^x + C
- ∫sin(x) dx = -cos(x) + C
- ∫cos(x) dx = sin(x) + C
- ∫sec^2(x) dx = tan(x) + C
- ∫cosec^2(x) dx = -cot(x) + C
- ∫sec(x)tan(x) dx = sec(x) + C
- ∫cosec(x)cot(x) dx = -cosec(x) + C
- ∫(1/x) dx = ln|x| + C
These formulas provide a starting point for evaluating indefinite integrals, and they can be combined and modified to handle more complex functions.
Indefinite Integrals of Special Functions
Certain functions have special indefinite integrals that are frequently encountered in calculus. Here are some examples:
- ∫a^x dx = (a^x)/(ln a) + C, where a > 0, a ≠ 1
- ∫log_a(x) dx = (1/ln a) * (1/x) + C
- ∫1/√(1-x^2) dx = arcsin(x) + C
These special functions have unique properties and require specific formulas to evaluate their indefinite integrals.
Indefinite Integral Equation
An indefinite integral equation is an equation that involves an unknown function and its derivative. It can be represented as:
∫f(x) dx = F(x) + C
In this equation, F(x) is the antiderivative of f(x), and C is the constant of integration. The constant of integration arises due to the fact that indefinite integrals are not unique. There are infinitely many functions that have the same derivative, and the constant allows us to account for this ambiguity.
Evaluating Indefinite Integral
To evaluate an indefinite integral, we follow a systematic process that involves applying the appropriate formulas and techniques. Here are the general steps to evaluate an indefinite integral:
- Identify the function and its integrand.
- Apply the relevant integral formulas based on the function’s properties.
- Simplify the integrand and integrate each term separately.
- Add the constant of integration to the result.
By following these steps, we can find the antiderivative and evaluate the indefinite integral of a given function.
Notation of Indefinite Integral
The notation used for indefinite integrals is ∫f(x) dx, where f(x) represents the function being integrated and dx indicates the variable of integration. The integral symbol (∫) represents the integration operation, and the dx represents the infinitesimal change in the variable x.
The notation emphasizes the fact that the indefinite integral is the antiderivative of the function and provides a way to express the relationship between the function and its integral.
Graphical Interpretation of Integrals
The graphical interpretation of integrals provides a visual understanding of the concept. Consider a function f(x) and its integral F(x). The derivative of F(x) is equal to f(x), which means that the slope of the tangent line to the graph of F(x) at any point is equal to the value of f(x) at that point.
Graphically, the integral of a function represents the area under the curve of the function. The definite integral, which has specified upper and lower limits, gives the exact numerical value of the area. In contrast, the indefinite integral represents a family of curves that have the same derivative.
How To Calculate Indefinite Integral?
To calculate the indefinite integral of a function, we follow a step-by-step process. Here’s how to calculate an indefinite integral:
- Identify the function and its integrand.
- Apply the appropriate integral formulas based on the function’s properties.
- Simplify the integrand and integrate each term separately.
- Add the constant of integration to the result.
It’s important to remember that the constant of integration allows for the infinite number of antiderivatives that exist for a given function. By following these steps, we can accurately calculate the indefinite integral of a function.
Properties of Indefinite Integral
Indefinite integrals have several important properties that make them useful in calculus. Here are some of the key properties:
- Linearity: The integral of the sum or difference of two functions is equal to the sum or difference of their integrals.
- Constant Multiple: The integral of a constant times a function is equal to the constant times the integral of the function.
- Additive Constant: The integral of a function plus a constant is equal to the integral of the function plus the same constant.
- Change of Variable: The integral of a function with respect to a variable can be rewritten in terms of a new variable, resulting in a different integral.
These properties allow us to manipulate and simplify indefinite integrals, making them easier to evaluate.
Indefinite Integral by Parts
Sometimes, evaluating an indefinite integral requires the use of integration by parts. Integration by parts is a technique that allows us to rewrite a complicated integral as a product of two functions, which can be easier to integrate.
The formula for integration by parts is:
∫u dv = uv – ∫v du
Here, u and v are functions of x, and du and dv are their respective differentials. By choosing appropriate u and dv, we can simplify the integral and find its antiderivative.
Indefinite vs. Definite Integral
While indefinite and definite integrals are both types of integration, there are some key differences between them. The main difference lies in the presence of limits.
An indefinite integral does not have any specific upper or lower limits, as it represents a family of functions with the same derivative. On the other hand, a definite integral has specified limits, which determine the range over which the integral is evaluated.
The definite integral gives a specific numerical value, which represents the area under the curve of a function between the given limits. In contrast, the indefinite integral provides the general solution to the integration problem, allowing for a range of possible functions.
Applications of Indefinite Integral
Indefinite integrals have numerous applications in mathematics and science. Some of the key applications include:
- Area and Volume Calculation: Indefinite integrals can be used to calculate the area between curves and the volume of solids.
- Physics: Indefinite integrals are used to analyze motion, calculate work done, and determine the center of mass of objects.
- Engineering: Indefinite integrals are used in engineering applications such as calculating electrical circuits, fluid mechanics, and structural analysis.
- Economics: Indefinite integrals are used in economics to model economic growth, calculate consumer surplus, and analyze production functions.
These are just a few examples of how indefinite integrals are applied in various fields. The versatility of indefinite integrals makes them a valuable tool in solving real-world problems.
Differential Equations and Constants of Integration
Differential equations are equations that involve derivatives of unknown functions. The solution to a differential equation often involves finding the indefinite integral of a function. When solving a differential equation, we obtain a family of functions, each of which represents a solution to the equation.
The constant of integration plays a crucial role in solving differential equations. It arises due to the fact that the derivative of a constant is zero. The constant allows for the inclusion of all possible solutions to the differential equation, accounting for the ambiguity introduced by the integration process.
Solved Examples on Indefinite Integral
Let’s take a look at some solved examples to further illustrate the concept of indefinite integrals:
Example 1: Evaluate the indefinite integral ∫(3x^2 – 6x + 2cosx) dx.
Solution: We can solve this integral by applying the properties of indefinite integrals. Using the linearity property, we can split the integral into three separate integrals:
∫3x^2 dx – ∫6x dx + ∫2cosx dx
Applying the power rule, we find:
(3/3)x^3 – (6/2)x^2 + 2sinx + C
Simplifying further:
x^3 – 3x^2 + 2sinx + C
Example 2: Evaluate the indefinite integral ∫(2x + 1)/(x^2 + x + 1) dx.
Solution: This integral can be solved using the technique of partial fractions. By decomposing the integrand into partial fractions, we can rewrite the integral as:
∫(2x + 1)/(x^2 + x + 1) dx = ∫(A/(x + α) + B/(x + β)) dx
By solving for the constants A and B, we find:
A = 1/3 and B = 2/3
Substituting these values back into the integral, we have:
∫(1/3)/(x + α) + (2/3)/(x + β) dx
= (1/3)ln|x + α| + (2/3)ln|x + β| + C
where α and β are the roots of the quadratic denominator.
Example 3: Evaluate ∫ (3x² – 6x + 2cosx) dx.
Solution: Split and solve the integral: ∫ 3x² dx – ∫ 6x dx + ∫ 2cosx dx = (3/3)x³ – (6/2)x² + 2sinx + C = x³ – 3x² + 2sinx + C.
How Kunduz Can Help You Learn Indefinite Integral?
At Kunduz, we understand the importance of mastering concepts like indefinite integrals in mathematics. That’s why we offer comprehensive resources and tools to help you learn and practice indefinite integrals. Our online platform provides step-by-step explanations, interactive examples, and a robust indefinite integral calculator.
With our intuitive interface, you can easily input your function and evaluate the indefinite integral with just a few clicks. Our calculator not only provides the result but also shows the steps involved in the integration process, allowing you to learn and understand the concept better.
Whether you’re a student looking to improve your calculus skills or a professional in need of quick and accurate integration solutions, Kunduz has you covered. Our platform is designed to make learning and solving indefinite integrals accessible, efficient, and affordable.
