When we think of a polygon, we often imagine shapes such as squares, triangles, or hexagons. But what exactly is a polygon? In simple terms, a polygon is a two-dimensional shape that is formed by connecting straight line segments. These line segments, also known as sides or edges, come together to create a closed shape with no curves. The points where the sides meet are called vertices or corners, and the angles formed at these points are known as interior angles.
Polygons are widely seen in our everyday lives. From the tiles on the floor to the signs on the road, many objects are polygonal in shape. For example, a stop sign is an octagon, and a starfish has a pentagonal shape. But polygons are not limited to specific forms or numbers of sides. They can vary in size, number of sides, and angles, making them a diverse category of shapes.
What is a Polygon?
A polygon is a two-dimensional figure that consists of a finite number of straight line segments connected end to end. These line segments form the sides of the polygon. The sides do not intersect, and the shape is closed, meaning that all the sides connect to each other to form a complete shape. A polygon can have any number of sides, but it must have at least three sides to be considered a polygon. Anything less than three sides would result in an open shape, which is not a polygon.
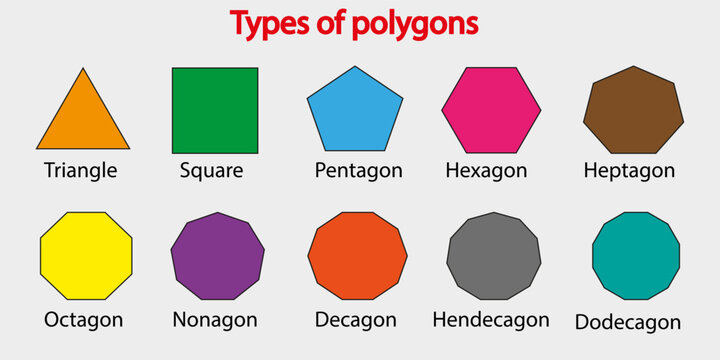
The name of a polygon is often determined by the number of sides it has. For example, a polygon with three sides is called a triangle, while a polygon with four sides is called a quadrilateral. A polygon with five sides is called a pentagon, and a polygon with six sides is called a hexagon. This naming convention continues as the number of sides increases, with names such as heptagon (7 sides), octagon (8 sides), nonagon (9 sides), and decagon (10 sides).
| Number of Sides | Polygon Shape |
|---|---|
| 3 | Triangle |
| 4 | Quadrilateral |
| 5 | Pentagon |
| 6 | Hexagon |
| 7 | Heptagon |
| 8 | Octagon |
| 9 | Nonagon |
| 10 | Decagon |
| 11 | Hendecagon |
| 12 | Dodecagon |
| 13 | Triskaidecagon |
| 14 | Tetrakaidecagon |
| 15 | Pentadecagon |
| … |
Polygon Chart
Here is a visual representation of some common polygons:

Polygon Formulas
Area of Polygons
The formula for finding the area of a polygon depends on the type of polygon. Here are some formulas for finding the area of common polygons:
| Name of Polygon | Area | Perimeter |
|---|---|---|
| Triangle | ½ x base x height | a+b+c |
| Square | side^2 | 4 x side |
| Rectangle | Length x Breadth | 2(length+breadth) |
| Parallelogram | Base x Height | 2(Sum of pair of adjacent sides) |
| Trapezoid | ½ (sum of parallel side) x height | Sum of all sides |
| Rhombus | ½ (Product of diagonals) | 4 x side |
| Pentagon | ¼√5(5+2√5) x side^2 | Sum of all five sides |
| Hexagon | 3√3/2 x (side)^2 | Sum of all six sides |
Perimeter of Polygons
The perimeter of a polygon is the total length of all its sides. Here are some formulas for finding the perimeter of common polygons:
| Polygon Shape | Formula for Perimeter |
|---|---|
| Triangle | Perimeter = side1 + side2 + side3 |
| Quadrilateral | Perimeter = side1 + side2 + side3 + side4 |
| Pentagon | Perimeter = side1 + side2 + side3 + side4 + side5 |
| Hexagon | Perimeter = side1 + side2 + side3 + side4 + side5 + side6 |
| … | … |
Polygon shapes
Triangle
A triangle is a polygon with three sides and three angles. It is one of the simplest and most common polygons. The sum of the interior angles of a triangle is always 180 degrees. Triangles can be further classified based on the lengths of their sides and the measures of their angles. Some common types of triangles include equilateral triangles, isosceles triangles, and scalene triangles.

Equilateral Triangle
An equilateral triangle is a triangle in which all three sides are equal in length. This means that all three angles of an equilateral triangle are also equal, measuring 60 degrees each.
Isosceles Triangle
In an isosceles triangle, two sides are equal in length, and the remaining side is different. The angles opposite the equal sides are also equal. The formula for finding the area of an isosceles triangle is:
Area = (1/2) * base * height
Scalene Triangle
A scalene triangle is a triangle in which all three sides have different lengths. The angles of a scalene triangle are also different. The formula for finding the area of a scalene triangle is:
Area = (1/2) * base * height
Quadrilateral
A quadrilateral is a polygon with four sides and four angles. The sum of the interior angles of a quadrilateral is always 360 degrees. Quadrilaterals can have various shapes and properties depending on the lengths of their sides and the measures of their angles. Some common types of quadrilaterals include squares, rectangles, parallelograms, and trapezoids.
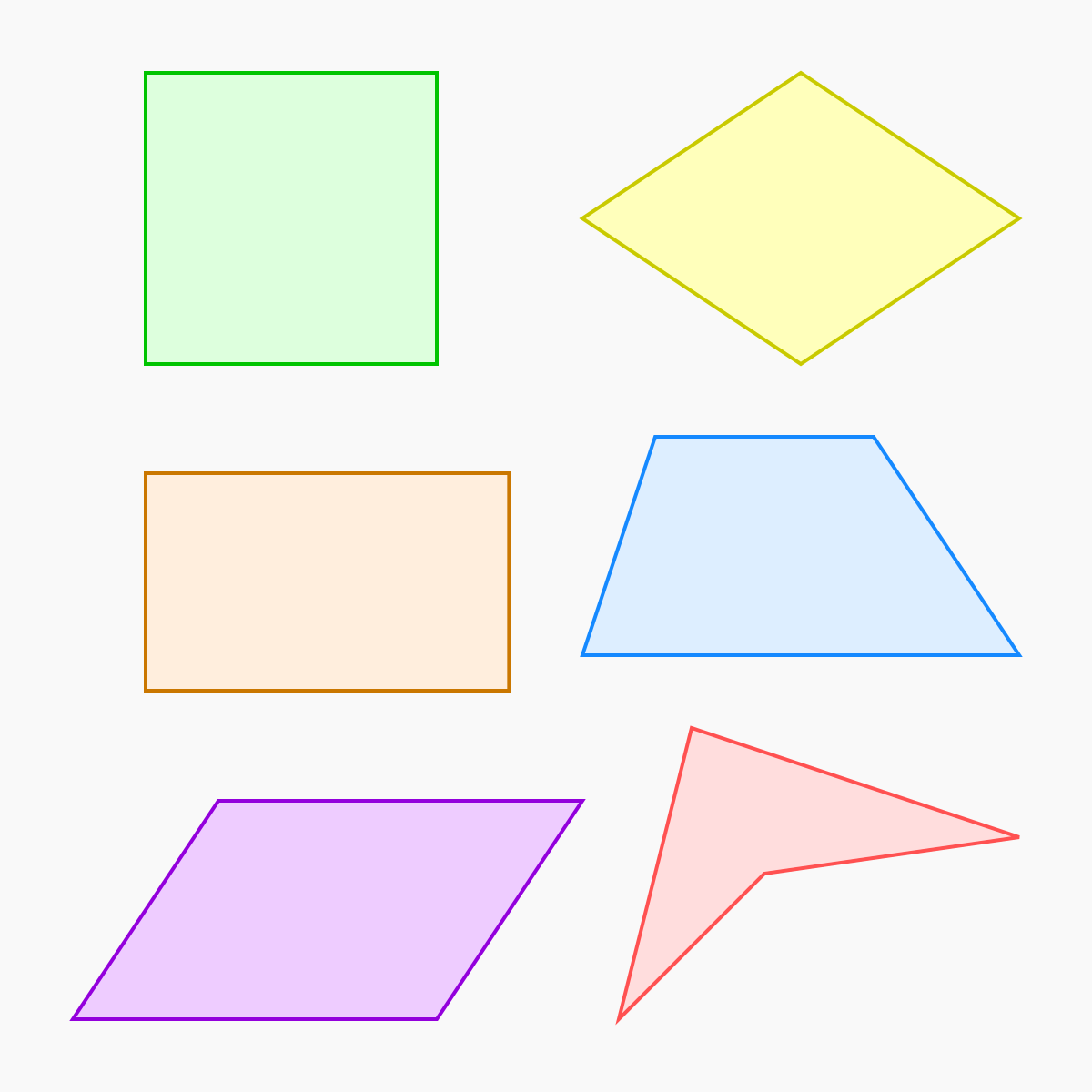
Square
A square is a quadrilateral in which all four sides are equal in length, and all four angles are right angles (90 degrees). The formula for finding the area of a square is:
Area = side^2

Rectangle
A rectangle is a quadrilateral in which opposite sides are equal in length, and all four angles are right angles (90 degrees). The formula for finding the area of a rectangle is:
Area = length * width

Parallelogram
A parallelogram is a quadrilateral in which opposite sides are parallel and equal in length. The formula for finding the area of a parallelogram is:
Area = base * height

Trapezoid
A trapezoid is a quadrilateral with one pair of parallel sides. The formula for finding the area of a trapezoid is:
Area = (1/2) * (sum of the lengths of the parallel sides) * height
Pentagon
A pentagon is a polygon with five sides and five angles. The sum of the interior angles of a pentagon is always 540 degrees. A regular pentagon has all sides and angles equal in measure. The formula for finding the area of a regular pentagon is:
Area = (1/4) * √(5(5 + 2√5)) * side^2
Hexagon
A hexagon is a polygon with six sides and six angles. The sum of the interior angles of a hexagon is always 720 degrees. A regular hexagon has all sides and angles equal in measure. The formula for finding the area of a regular hexagon is:
Area = (3√3/2) * side^2
Angles of Polygon
A polygon has both interior and exterior angles. The interior angles are the angles formed inside the polygon at each vertex, while the exterior angles are the angles formed outside the polygon at each vertex.
Interior Angle Property
The sum of the interior angles of a polygon with n sides can be calculated using the formula:
Sum of interior angles = (n – 2) * 180 degrees
Exterior angle property
The sum of the interior and exterior angles at each vertex of any polygon is always 180 degrees. In other words, the exterior angle at a vertex is equal to the sum of the interior angle and the exterior angle at that vertex.
Types of Polygon
Polygons can be classified into different types based on their sides and angles. The main types of polygons are:
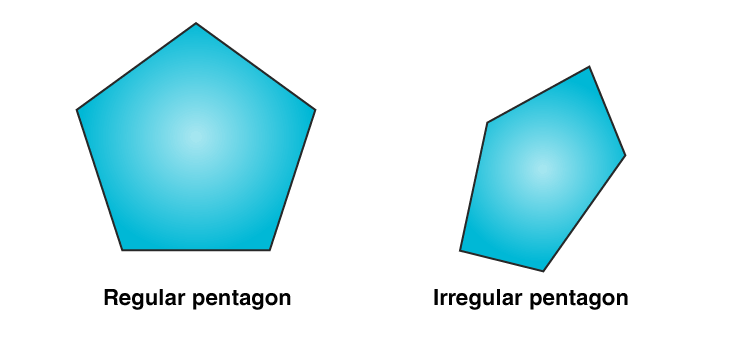
Regular Polygon
A regular polygon is a polygon in which all sides and angles are equal in measure. Some examples of regular polygons include equilateral triangles, squares, and regular hexagons.
Irregular Polygon
An irregular polygon is a polygon in which the sides and/or angles are not equal in measure. Examples of irregular polygons include scalene triangles, rectangles, and trapezoids.
Convex Polygon
A convex polygon is a polygon in which all interior angles are less than 180 degrees. In a convex polygon, all diagonals lie within the interior of the polygon.
Concave Polygon
A concave polygon is a polygon in which at least one interior angle is greater than 180 degrees. In a concave polygon, some diagonals may lie outside the polygon.
Properties of Polygons
Polygons have several properties that can be used to identify and classify them. Some important properties of polygons include:
- The sum of the interior angles of a polygon with n sides is given by the formula (n – 2) * 180 degrees.
- The sum of the exterior angles of any polygon is always 360 degrees.
- The number of diagonals in a polygon with n sides is given by the formula n(n – 3)/2.
- The measure of each interior angle of a regular polygon with n sides is given by [(n – 2) * 180 degrees]/n.
- The measure of each exterior angle of a regular polygon with n sides is given by 360 degrees/n.
- The perimeter of a polygon is the sum of the lengths of its sides.
- The area of a polygon can be found using various formulas based on the type of polygon.
Solved Examples on Polygon
Let’s look at some solved examples to better understand polygons:
Example 1: Consider a regular hexagon. It has six sides, so n equals 6. The polygon’s sum of exterior angles equals 360°. Therefore, each exterior angle equals 360°/n, which simplifies to 360°/6, or 60°.
Example 2: Consider a polygon with an interior angle sum of 1260°. To find the number of sides it has, we use the formula for the sum of the interior angles of a polygon, (n – 2) x 180°. Solving 1260° equals (n – 2) x 180° gives n equals 9. So, the given polygon has nine sides.
Example 3: Find the sum of the interior angles of a hexagon.
Solution: The formula for the sum of the interior angles of a polygon with n sides is (n – 2) * 180 degrees. Substituting n = 6, we have:
Sum of interior angles = (6 – 2) * 180 degrees = 4 * 180 degrees = 720 degrees
Therefore, the sum of the interior angles of a hexagon is 720 degrees.
How Kunduz Can Help You Learn Polygon?
At Kunduz, we understand that learning about polygons and their properties can be challenging. That’s why we offer a variety of resources and tools to help you master this topic. Whether you’re a student looking for clear explanations or a teacher seeking engaging lesson plans, Kunduz has you covered.
Our online platform provides interactive tutorials, practice exercises, and educational videos to enhance your understanding of polygons. With step-by-step guidance and real-world examples, you’ll gain the confidence to solve polygon-related problems and excel in your geometry studies.