Pythagorean triples are sets of three positive integers that satisfy the Pythagorean theorem. This theorem states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. Pythagorean triples are represented as (a, b, c), where ‘a’ and ‘b’ are the legs of the triangle, and ‘c’ is the hypotenuse.
The concept of Pythagorean triples originates from the ancient Greek mathematician Pythagoras, who discovered the famous Pythagorean theorem. This theorem is fundamental in geometry and has numerous applications in various fields, including architecture, engineering, and physics.
What is a Pythagorean Triple?
In simple terms, a Pythagorean triple consists of three positive integers a, b, and c, such that their relationship satisfies the equation a² + b² = c². This equation is derived from Pythagoras’ Theorem – a fundamental principle in the realm of Geometry. In the context of a right-angled triangle, ‘a’ and ‘b’ are the lengths of the two shorter sides (perpendicular and base), while ‘c’ represents the length of the longest side (hypotenuse).
What is the Pythagorean Theorem?
The Pythagorean theorem is a fundamental principle in Euclidean geometry. It states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be written as: a² + b² = c². The theorem is named after the ancient Greek mathematician Pythagoras, who by tradition is credited with its discovery.
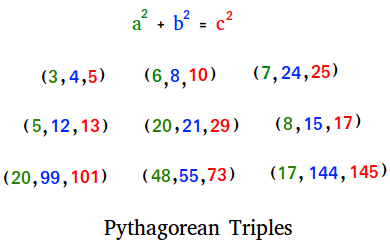
List of Pythagorean Triples
Pythagorean triples are infinite in number, but there are some common triples that are frequently encountered. Here is a list of Pythagorean triples:
- (3, 4, 5)
- (5, 12, 13)
- (8, 15, 17)
- (7, 24, 25)
- (20, 21, 29)
- (12, 35, 37)
- (9, 40, 41)
- (28, 45, 53)
- (11, 60, 61)
- (16, 63, 65)
- (33, 56, 65)
- (48, 55, 73)
- (13, 84, 85)
- (36, 77, 85)
- (39, 80, 89)
- (65, 72, 97)
This is just a small selection of the many Pythagorean triples that exist. The list goes on indefinitely as you can multiply each number in a Pythagorean triple by any positive integer to generate another valid triple.
Formula for Generating Pythagorean Triples
The formula for generating Pythagorean triples is derived from the Pythagorean theorem itself. If we have two integers ‘m’ and ‘n’ such that m>n>0, the Pythagorean triples (a, b, c) can be generated as follows:
- a = m² – n²
- b = 2mn
- c = m² + n²
This formula, known as Euclid’s formula, is an efficient way to generate Pythagorean triples.
Pythagorean Triples Proof
The Pythagorean theorem can be proved using geometric and algebraic methods. One geometric proof involves constructing squares on each side of a right-angled triangle and comparing their areas.
By constructing squares on the sides of a right-angled triangle, we can visualize the relationship between the areas of the squares and the lengths of the sides. The sum of the areas of the smaller squares (a² and b²) is equal to the area of the larger square (c²).
This geometric proof confirms the validity of the Pythagorean theorem and provides a visual representation of why it holds true for all right-angled triangles.
How to Generate Pythagorean Triples?
The process of generating Pythagorean triples is quite straightforward. Below are the step-by-step procedures:
Step 1: Choose two positive integers ‘m’ and ‘n’ such that m > n.
Step 2: Substitute the values of ‘m’ and ‘n’ into Euclid’s formula: a = m² – n², b = 2mn, c = m² + n².
Step 3: The resulting values of ‘a’, ‘b’, and ‘c’ are the required Pythagorean triple.
By following these steps, one can generate any number of Pythagorean triples.
Types of Pythagorean Triples
Pythagorean triples can be categorized into three types: common, primitive, and non-primitive.
Common Pythagorean Triples
Common Pythagorean triples are the most frequently encountered triples. They are often used as examples to illustrate the Pythagorean theorem. Some common Pythagorean triples include (3, 4, 5), (5, 12, 13), and (8, 15, 17).

Primitive Pythagorean Triples
A primitive Pythagorean triple is one in which the three numbers have no common factors other than 1. In other words, the lengths of the sides of the triangle are relatively prime. For example, (3, 4, 5) is a primitive Pythagorean triple because the numbers 3, 4, and 5 have no common factors other than 1.

Non-Primitive Pythagorean Triples
Non-primitive Pythagorean triples are multiples of primitive triples. By multiplying each number in a primitive triple by the same positive integer, you can generate a non-primitive triple. For example, multiplying the numbers in the primitive triple (3, 4, 5) by 2 gives the non-primitive triple (6, 8, 10).
How to Find Pythagorean Triples?
The process of finding Pythagorean triples involves identifying three integers that satisfy the Pythagorean theorem. This can be done either by using Euclid’s formula or by checking whether a given set of three numbers satisfies the theorem. In the latter approach, if the equation a² + b² = c² holds true for the three numbers, they are considered a Pythagorean triple.
Properties of Pythagorean Triples
Pythagorean triples have several interesting properties that can be explored. Some of these properties include:
- The sum of the squares of the two smaller sides is equal to the square of the largest side.
- Pythagorean triples can be generated by multiplying each number in a primitive triple by the same positive integer.
- The difference between the squares of the two smaller sides is always an odd number.
- There are infinitely many Pythagorean triples, as they can be generated using various formulas and methods.
- Pythagorean triples have applications in various fields, including geometry, trigonometry, and number theory.
By studying the properties of Pythagorean triples, you can gain a deeper understanding of their characteristics and explore their mathematical significance.
Triangular Numbers
Triangular numbers are a sequence of numbers that can be represented as the sum of consecutive positive integers. They are called triangular numbers because they can be arranged in the shape of an equilateral triangle. For example, the numbers 1, 3, 6, 10, 15, and so on are triangular numbers.
Interestingly, every perfect square can be expressed as the sum of two consecutive triangular numbers. This property is closely related to the Pythagorean theorem and the concept of Pythagorean triples.
Solved Examples on Pythagorean Triples
Let’s look at some examples to understand how to find Pythagorean triples:
Example 1: Verify whether (7, 24, 25) is a Pythagorean triple.
Solution: To verify, we check whether the numbers satisfy the Pythagorean theorem a² + b² = c².
Substituting the values, we get: 7² + 24² = 25²
Simplifying, we find that 49 + 576 = 625, which holds true. Hence, (7, 24, 25) is a Pythagorean triple.
Example 2: Find the Pythagorean triple where one of the numbers is 12.
Solution: Since 12 is an even number, we use the formula for even numbers to find the other two numbers: a = n, b = (n/2)² – 1, c = (n/2)² + 1.
Substituting n = 12 into the formulas, we get: a = 12, b = (12/2)² – 1 = 35, c = (12/2)² + 1 = 37.
Hence, the Pythagorean triple is (12, 35, 37).
How Kunduz Can Help You Learn Pythagorean Triples?
Our experienced instructors provide step-by-step explanations to ensure that you grasp the fundamentals of Pythagorean triples and related topics. Whether you’re a beginner or looking to deepen your understanding, Kunduz has the resources to support your learning journey.
Start exploring our online courses and resources today to enhance your understanding of Pythagorean triples and unlock your full mathematical potential.