In the world of geometry, angles play a fundamental role in understanding the relationships between shapes and lines. One important concept to grasp is that of supplementary angles. Supplementary angles are a pair of angles that add up to 180 degrees. They can be adjacent, meaning they share a common vertex and side, or non-adjacent, where there is no shared side or vertex.
Understanding supplementary angles is crucial not only for solving geometric problems but also for real-world applications. From architecture to engineering, supplementary angles find their place in various fields. In this comprehensive guide, we will explore the definition, properties, and methods for finding supplementary angles. So, let’s dive in and unravel the mysteries of supplementary angles!
What are Supplementary Angles?
Supplementary angles are two angles that, when combined, add up to 180 degrees. This means that the sum of the measures of the two angles is equal to 180 degrees. The angles can be adjacent or non-adjacent, depending on whether they share a common side and vertex.
When two adjacent angles are supplementary, they form a straight line. This is because a straight line measures 180 degrees, and when the two adjacent angles are added together, they complete the straight line. On the other hand, non-adjacent supplementary angles do not form a straight line but still have a combined measure of 180 degrees.
Properties of Supplementary Angles
Supplementary angles possess several intriguing properties that make them unique and useful in geometry. Here are some key properties of supplementary angles:
- Sum of Measures: The sum of the measures of supplementary angles is always equal to 180 degrees. This property is the defining characteristic of supplementary angles.
- Adjacent Supplementary Angles: Adjacent supplementary angles share a common vertex and side. When combined, they form a straight angle or a straight line measuring 180 degrees.
- Non-Adjacent Supplementary Angles: Non-adjacent supplementary angles do not share a common side or vertex. Despite this, their combined measures still equal 180 degrees.
- Complement of Supplementary Angles: If two angles are supplementary to the same angle, they are congruent. In other words, if angle A and angle B are both supplementary to angle C, then angle A is congruent to angle B.
- Vertical Angles: Vertical angles, also known as opposite angles, are formed when two lines intersect. Vertical angles are always congruent, and if one pair of vertical angles is supplementary, then all pairs of vertical angles are supplementary.
How To Find Supplementary Angles
Finding supplementary angles involves identifying two angles whose measures add up to 180 degrees. This process requires understanding the properties of supplementary angles and applying them to solve for unknown angle measures. Here’s a step-by-step guide to finding supplementary angles:
- Identify the Given Angle Measures: Determine the measures of the known angles in the problem. This information will help you find the measure of the unknown angle.
- Set Up the Equation: Use the fact that the sum of the measures of supplementary angles is 180 degrees to set up an equation. If the measures of the angles are represented by variables, assign one variable to each angle.
- Solve the Equation: Solve the equation for the unknown variable. This will give you the measure of the unknown angle.
- State the Answer: Clearly state the measure of the unknown angle, ensuring it is in the appropriate units (usually degrees).
By following these steps, you can successfully find the measure of supplementary angles and solve related problems in geometry.
How To Find the Measure of Supplementary Angles
To find the measure of a supplementary angle, you need to know the measure of the other angle. By subtracting the known angle measure from 180 degrees, you can determine the measure of the supplementary angle. Here’s a step-by-step process for finding the measure of a supplementary angle:
- Identify the Known Angle Measure: Determine the measure of the known angle.
- Subtract from 180: Subtract the known angle measure from 180 degrees. The result is the measure of the supplementary angle.
- State the Answer: Clearly state the measure of the supplementary angle, ensuring it is in the appropriate units (usually degrees).
By following these steps, you can find the measure of a supplementary angle when given the measure of its counterpart.
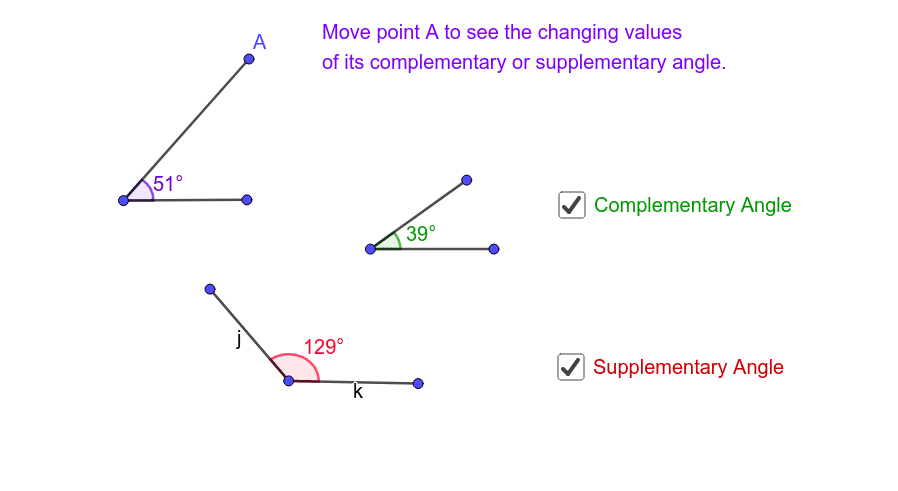
Complementary vs Supplementary Angles
While supplementary angles add up to 180 degrees, complementary angles add up to 90 degrees. Complementary angles are another important concept in geometry, and it’s crucial to understand the difference between the two.
The main distinction lies in the sum of the angle measures. Complementary angles add up to 90 degrees, while supplementary angles add up to 180 degrees.
Complementary angles are often associated with right angles, as the sum of a right angle and its complement is always 90 degrees. On the other hand, supplementary angles are associated with straight lines, as their sum equals 180 degrees, forming a straight angle.
Complementary vs Supplementary Angles Table
To further illustrate the difference between complementary and supplementary angles, here’s a table summarizing their key characteristics:
| Complementary Angles | Supplementary Angles | |
|---|---|---|
| Sum | 90 degrees | 180 degrees |
| Example | 30 degrees + 60 degrees = 90 degrees | 120 degrees + 60 degrees = 180 degrees |
| Relationship | Complements each other | Supplements each other |
| Form | Right angle | Straight angle |
As you can see, complementary angles form a right angle when combined, while supplementary angles form a straight angle. Understanding these distinctions will help you solve problems involving angles more effectively.
What Are Adjacent and Non-adjacent Supplementary Angles?
Supplementary angles can be either adjacent or non-adjacent. Adjacent supplementary angles share a common vertex and a common side, forming a straight line. Non-adjacent supplementary angles, on the other hand, do not share a common vertex or a common side, but their measures still add up to 180 degrees.
Adjacent Supplementary Angles
Adjacent supplementary angles share a common vertex and a common side. When placed adjacent to each other, these angles form a straight line or a ‘straight angle,’ measuring 180 degrees.
Non-Adjacent Supplementary Angles
Non-adjacent supplementary angles do not share a common vertex or a common side. Despite this, they are still considered supplementary as long as their measures add up to 180 degrees.
Supplementary Angles Theorem and Proof
The Supplementary Angles Theorem states that if two angles are supplementary to the same angle, then they are congruent. In other words, if Angle A and Angle B are both supplementary to Angle C, then Angle A is congruent to Angle B.
To prove this theorem, we can use a simple proof by contradiction. Let’s assume that Angle A and Angle B are supplementary to Angle C, but Angle A is not congruent to Angle B.
- Assume that Angle A and Angle B are supplementary to Angle C, but Angle A is not congruent to Angle B.
- Since Angle A and Angle B are supplementary, their sum is equal to 180 degrees: Angle A + Angle B = 180 degrees.
- Let’s suppose that Angle A is greater than Angle B: Angle A > Angle B.
- Since Angle A is greater than Angle B, we can subtract Angle B from both sides of the equation: Angle A – Angle B > Angle B – Angle B.
- This simplifies to: Angle A – Angle B > 0.
- But since Angle A and Angle B are supplementary, their difference should be equal to 0: Angle A – Angle B = 0.
- This contradiction proves that our assumption was incorrect. Therefore, Angle A must be congruent to Angle B.
The proof demonstrates that if two angles are supplementary to the same angle, they must be congruent. This property of supplementary angles is useful in various geometric proofs and problem-solving scenarios.
Facts About Supplementary Angles
Here are some interesting facts about supplementary angles that can deepen your understanding of this geometric concept:
- Straight Angle: Supplementary angles can form a straight angle when combined. A straight angle measures 180 degrees and is formed when two adjacent supplementary angles are put together.
- Complement of Supplementary Angles: If two angles are supplementary to the same angle, they are congruent. This means that if Angle A and Angle B are both supplementary to Angle C, then Angle A is congruent to Angle B.
- Vertical Angles: Vertical angles are formed when two lines intersect. Vertical angles are always congruent, and if one pair of vertical angles is supplementary, then all pairs of vertical angles are supplementary.
- Decomposing Angles: Supplementary angles can be used to decompose angles into smaller parts. By breaking down an angle into two supplementary angles, you can find the measurement of an unknown angle part.
These facts highlight the significance of supplementary angles in geometry and their applications in various problem-solving scenarios.
Frequently Asked Questions About Supplementary Angles
Can 2 Acute Angles be Supplementary Angles?
No, two acute angles cannot be supplementary angles. Acute angles are angles that measure less than 90 degrees. If two angles are acute, their sum will always be less than 180 degrees, which is the requirement for being supplementary angles.
Can Three Angles be Supplementary?
No, three angles cannot be supplementary even if their sum is 180 degrees. Supplementary angles always occur in pairs, with two angles adding up to 180 degrees. The definition of supplementary angles holds true only for two angles.
Can 2 Obtuse Angles be Supplementary Angles?
No, two obtuse angles cannot be supplementary angles. Obtuse angles are angles that measure more than 90 degrees. If two angles are obtuse, their sum will always be greater than 180 degrees, which does not satisfy the requirement for being supplementary angles.
Can 2 Right Angles be Supplementary Angles?
Yes, two right angles are always supplementary because a right angle measures exactly 90 degrees. If two right angles are added together, their sum will be equal to 180 degrees, which satisfies the requirement for being supplementary angles.
Are supplementary and complementary angles the same?
No, supplementary angles and complementary angles are not the same. Supplementary angles add up to 180 degrees, while complementary angles add up to 90 degrees.
What angle is formed if we put the supplementary angles together?
When supplementary angles are combined, they form a straight angle or a straight line measuring 180 degrees. This means that the angles are placed next to each other to create a straight line.
What do Supplementary Angles Add up to?
Supplementary angles add up to 180 degrees. This means that the sum of the measures of two supplementary angles is equal to 180 degrees.
Can Vertical Angles Be Supplementary?
Yes, vertical angles can be supplementary. Vertical angles are formed when two lines intersect, and they are always congruent. If one pair of vertical angles is supplementary, then all pairs of vertical angles are supplementary.
When are supplementary angles congruent?
Supplementary angles are congruent when they are both supplementary to the same angle. In other words, if Angle A and Angle B are both supplementary to Angle C, then Angle A is congruent to Angle B.
These frequently asked questions provide further clarity on the properties and characteristics of supplementary angles, helping to deepen your understanding of this important concept in geometry.
Solved Examples on How to Use the Supplementary Angles
Let’s consider some solved examples to understand the concept of supplementary angles better.
Example 1: Find the supplement of an angle measuring 75 degrees.
Solution: Supplement = 180 – known angle Supplement = 180 – 75 = 105 degrees
Example 2: If angle A is 58 degrees, what is its supplement?
Solution: Supplement of angle A = 180 – Angle A Supplement of angle A = 180 – 58 = 122 degrees
Example 3: Two angles are supplementary. If one angle measures 95 degrees, what is the measure of the other angle?
Solution: The other angle = 180 – given angle The other angle = 180 – 95 = 85 degrees
How Kunduz Can Help You Learn Supplementary Angles
Kunduz is your go-to resource for learning and mastering supplementary angles. With our comprehensive lessons, interactive activities, and practice problems, you’ll develop a deep understanding of this geometric concept in no time.
Our platform offers engaging and interactive lessons that break down complex topics into digestible explanations. You’ll find visual aids, step-by-step examples, and real-world applications to help you grasp the concept of supplementary angles.
In addition to lessons, Kunduz provides interactive activities and practice problems to reinforce your understanding. You’ll have the opportunity to apply your knowledge, solve problems, and gain confidence in your geometry skills.
Whether you’re a student looking to improve your grades or a teacher seeking resources to enhance your lessons, Kunduz has you covered. Our user-friendly platform and comprehensive content make learning supplementary angles enjoyable and accessible.
So, why wait? Start exploring Kunduz today and unlock your full potential in geometry and beyond!
