Viscosity is a fundamental property of fluids that measures their resistance to flow. In the case of water, viscosity plays a crucial role in determining how easily it flows and interacts with other substances. In this comprehensive guide, we will explore the concept of viscosity, delve into the formulas used to calculate it, and examine the different types of viscosity. So, let’s dive in!
An Introduction to Viscosity of Water
When we observe the flow of different liquids, such as water and honey, it becomes evident that they behave differently. Water flows much faster compared to honey, which is more viscous. Viscosity is the property of a liquid that measures its resistance to flow. It is determined by the internal friction between different layers of the liquid when there is relative motion between them.
Viscosity can be understood by imagining a fluid moving parallel to a horizontal plane. Layers of the fluid have different velocities, with the topmost layer having the maximum velocity. As the distance from the horizontal plane increases, the velocity of the layers decreases. The difference in velocity between adjacent layers gives rise to the viscous force, which opposes the flow and determines the viscosity of the liquid.
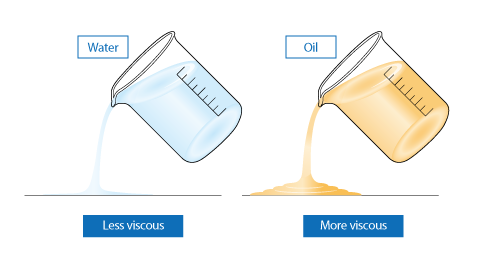
What is Viscosity of Water?
The viscosity of water refers to the measure of its resistance to flow. It is influenced by various factors such as temperature, pressure, and the strength of forces between water molecules. At room temperature, water has a relatively low viscosity compared to other liquids. As the temperature decreases, the viscosity of water increases, and vice versa.
Understanding the viscosity of water is essential in various fields, including physics, chemistry, and engineering. It helps in designing efficient fluid systems, calculating flow rates, and predicting the behavior of liquids under different conditions.
To get a better understanding of the viscosity of water, let’s take a look at the dynamic and kinematic viscosity of water at different temperatures:
| Temperature (°C) | Dynamic viscosity (mPa⋅s) | Kinematic viscosity (mm2/s) | Fluid density (g/cm3) |
|---|---|---|---|
| 5 | 1.518 | 1.53 | 1.000 |
| 10 | 1.306 | 1.31 | 0.999 |
| 15 | 1.138 | 1.14 | 0.999 |
| 20 | 1.002 | 1.00 | 0.998 |
| 25 | 0.894 | 0.89 | 0.997 |
| 30 | 0.797 | 0.80 | 0.995 |
| 35 | 0.719 | 0.72 | 0.994 |
| 40 | 0.653 | 0.65 | 0.992 |
What is Viscosity?
Viscosity is a property of fluids that measures their resistance to deformation at a given rate. It is the internal friction or the tangential force that arises between adjacent layers of the fluid when there is relative motion between them. Viscosity is often described as the thickness or stickiness of a fluid.
The reciprocal of viscosity is called fluidity, which is a measure of how easily a fluid flows. For many fluids, the tangential or shearing stress that causes the flow is directly proportional to the rate of shear strain. This relationship is known as Newton’s law of viscosity.

Viscosity of Water Formula
The viscosity of a fluid is calculated using the formula F=ηA(u/y) where:
Fis the forceηis the viscosity of the fluidAis the area of each plateu/yis the rate of shear deformation
This formula helps in determining the viscosity of a fluid, including water, under different conditions.
Coefficient of Viscosity of Water
The coefficient of viscosity of water is a measure of the internal friction or the resistance that occurs when layers of the fluid rub against each other.
The formula for the coefficient of viscosity of water is η = F.dx/A.dv where:
ηis the coefficient of viscositydv/dxis the velocity gradient between two layers of the liquidFis the viscous forceAis the surface area
The SI unit of the coefficient of viscosity is Newton-second per square meter (Ns.m⁻²).
Types of Viscosity
There are two main types of viscosity: dynamic viscosity and kinematic viscosity.
Dynamic Viscosity
Dynamic or absolute viscosity is the measure of a fluid’s internal friction. It is defined as the resistance to flow when an external force acts on it.
The formula for dynamic viscosity can be written as η = T/γ where η is the dynamic viscosity of water, T is shearing stress, and γ is the shear rate of change.
The SI unit for dynamic viscosity is Pascal-second (Pa.s).
Kinematic Viscosity
Kinematic viscosity, on the other hand, is defined as the ratio of dynamic viscosity to its density. It is a useful measure in many industrial applications, for instance, in the design of pipeline systems where the properties of the water flow need to be considered.
The kinematic viscosity of water can be calculated using the formula v=η/ρ where v is the kinematic viscosity of water, η is the dynamic viscosity of water, and ρ is the density of water.
The SI unit for kinematic viscosity is square meter per second (m²/s).
How to Calculate the Viscosity of Water?
The viscosity of water can be calculated using the formula η = F.dx/A.dv. Here, η is the coefficient of viscosity, dv/dx is the velocity gradient between two layers of the liquid, F is the viscous force, and A is the surface area. By substituting the known values into this formula, one can calculate the viscosity of water under different conditions.
How to Calculate the Kinematic Viscosity of Water?
The calculation of kinematic viscosity involves the ratio of dynamic viscosity to the density of the liquid. The formula used is v=η/ρ. Here, v represents the kinematic viscosity of water, η stands for the dynamic viscosity of water, and ρ indicates the density of water. By plugging in the known values, one can calculate the kinematic viscosity of water.
Solved Examples on Viscosity of Water
Example 1:
Imagine that a layer of glycerin, measuring 3mm in thickness, is placed between a flat plate and a large plate. The viscosity coefficient of glycerin is given as 2 N s/m² and the area of the plane plate is 48 cm². What would be the force required to move the plate at a speed of 6 cm/s?
Given:
- Thickness of the layer, dx = 3 mm = 3 × 10⁻³ m
- Coefficient of viscosity, η = 2 N s/m²
- Change in speed, dv = 6 cm/s = 6 × 10⁻² m/s
- Area of the plate, A = 48 cm² = 48 × 10⁻⁴ m²
Using the formula F = ηA(u/y), the force required to move the plate would be calculated as follows:
F = 2 N s/m² × 48 × 10⁻⁴ m² × (6 × 10⁻² m/s / 3 × 10⁻³ m) = 192 × 10⁻³ N = 0.192 N
Example 2:
Consider a pipe with a diameter of 2 cm. What would be the maximum average speed of water for a level flow, considering that the viscosity coefficient for water is 0.001 N-s/m²?
Given:
- Diameter of the pipe, D = 2 cm = 0.02 m
- Viscosity coefficient, η = 0.001 N-s/m²
- Density of water, ρ = 1000 kg/m³
Given that the maximum value of K for level flow is 2000, the maximum speed of water can be calculated using the formula v = Kη / ρD as follows:
v = 2000 × 0.001 N-s/m² / 1000 kg/m³ × 0.02 m = 0.1 m/s
These examples provide a practical understanding of the concept of viscosity and how it can be calculated in different scenarios.
How Kunduz Can Help You Learn Viscosity of Water?
At Kunduz, we understand the importance of mastering concepts like viscosity and its various properties. We provide comprehensive learning resources and expert guidance to help you excel in mathematics, physics, and other subjects. With our step-by-step explanations, solved examples, and interactive learning tools, you can develop a strong understanding of complex topics.
Our platform offers a wide range of educational materials, including video lessons, practice exercises, and quizzes. Whether you’re a student preparing for exams or an enthusiast looking to expand your knowledge, Kunduz can help you achieve your academic goals.
Don’t let the complexities of viscosity hold you back. Join Kunduz today and embark on a journey of learning and discovery!
