1 2 3 PL K 111 LLL Evaluate the following integrals using

Last updated: 5/10/2023

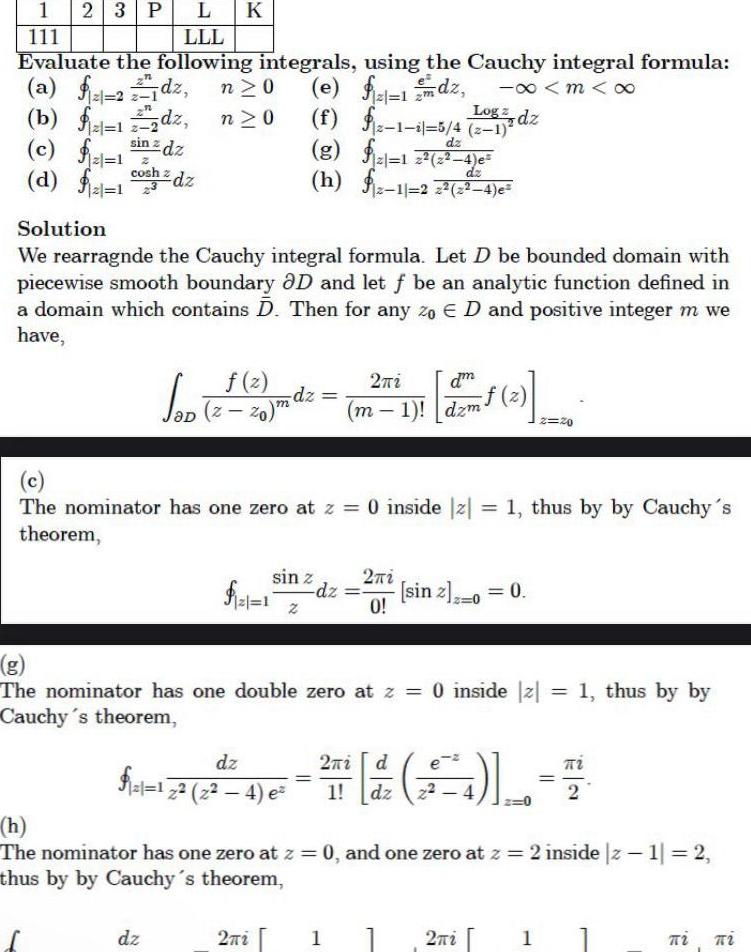
1 2 3 PL K 111 LLL Evaluate the following integrals using the Cauchy integral formula a 2 dz m n 0 e z 1 mdz f f 2 1 1 5 4 2 1 Log dz b dz n20 g h sin z dz z cosh z c d 21 123 z 1 dz Solution We rearragnde the Cauchy integral formula Let D be bounded domain with piecewise smooth boundary D and let f be an analytic function defined in a domain which contains D Then for any zo E D and positive integer m we have D f z z dz dz m c The nominator has one zero at z 0 inside z 1 thus by by Cauchy s theorem sin z 1 1 z dz z 1 z 2 4 e 2 1 222 22 4 e dz dz 1 1 z z 4 e 2 i 2 i dm m1 dzm f 2 i dz sin 2 0 0 g The nominator has one double zero at z 0 inside z 1 thus by by Cauchy s theorem 2 20 h The nominator has one zero at z 0 and one zero at z 2 inside z 1 2 thus by by Cauchy s theorem 2 i d 1 2 2 1 dz 1 1 2 i 1 1 i i