1 cos x 2 cos x sin x u 8 1 u 2 3 cos x 15 15 3 cos x 13 13 1 cos cos x sin x cos 5 2 3 cos 2 15 13 At least one of the answers

Question

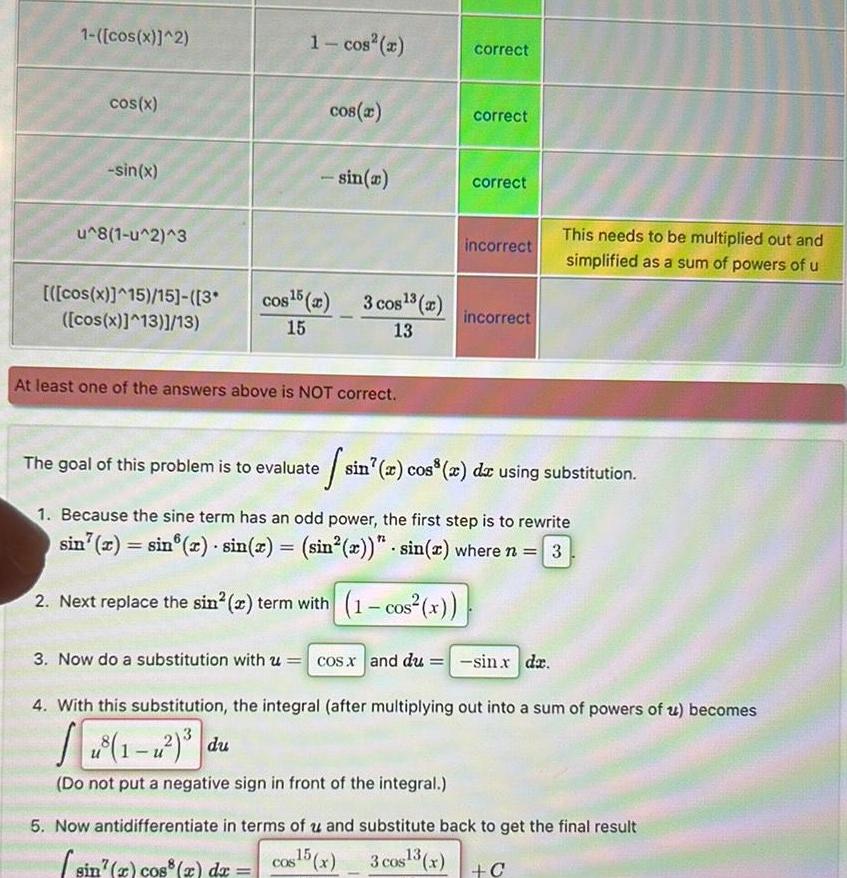
1 cos x 2 cos x sin x u 8 1 u 2 3 cos x 15 15 3 cos x 13 13 1 cos cos x sin x cos 5 2 3 cos 2 15 13 At least one of the answers above is NOT correct correct correct correct incorrect incorrect The goal of this problem is to evaluate sin x cos x dx using substitution 1 Because the sine term has an odd power the first step is to rewrite sin x sin x sin x sin x sin x where n 3 2 Next replace the sin 2 term with 1 cos x This needs to be multiplied out and simplified as a sum of powers of u 3 Now do a substitution with u cos x and du sin x dx 4 With this substitution the integral after multiplying out into a sum of powers of u becomes Do not put a negative sign in front of the integral 5 Now antidifferentiate in terms of u and substitute back to get the final result cos15 x 3 cos 3 x sin x cos x dx C

Kunduz · Accepted Answer

Click to see the answer