8 1 1 The magnetic field of a single current loop A single current loop is shown in Figure 8 1 below This figure was taken from

Question

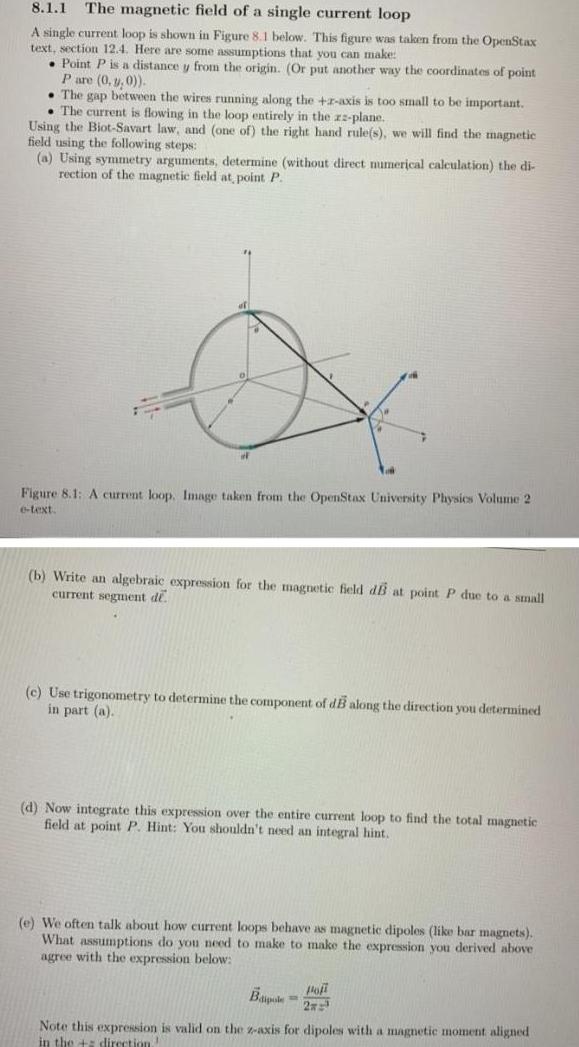
8 1 1 The magnetic field of a single current loop A single current loop is shown in Figure 8 1 below This figure was taken from the OpenStax text section 12 4 Here are some assumptions that you can make Point P is a distance y from the origin Or put another way the coordinates of point Pare 0 3 0 The gap between the wires running along the z axis is too small to be important The current is flowing in the loop entirely in the zz plane Using the Biot Savart law and one of the right hand rule s we will find the magnetic field using the following steps a Using symmetry arguments determine without direct mumerical calculation the di rection of the magnetic field at point P Figure 8 1 A current loop Image taken from the OpenStax University Physics Volume 2 e text b Write an algebraic expression for the magnetic field dB at point P due to a small current segment de c Use trigonometry to determine the component of dB along the direction you determined in part a d Now integrate this expression over the entire current loop to find the total magnetic field at point P Hint You shouldn t need an integral hint e We often talk about how current loops behave as magnetic dipoles like bar magnets What assumptions do you need to make to make the expression you derived above agree with the expression below Bipole Note this expression is valid on the z axis for dipoles with a magnetic moment aligned in the direction Poft 2

Kunduz · Accepted Answer

Click to see the answer