function defined by wherever the limit exists is defined to

Last updated: 10/10/2023

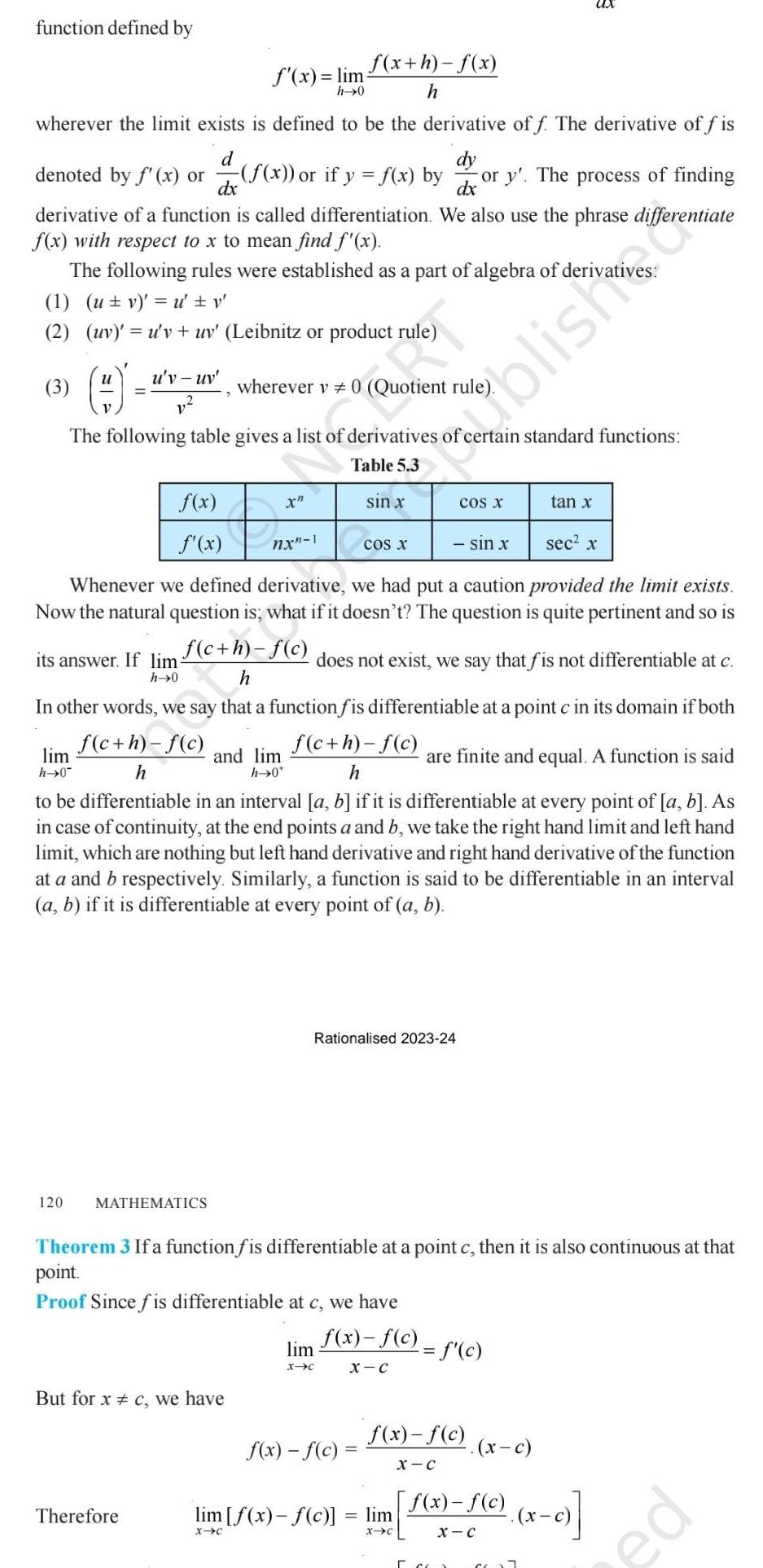
function defined by wherever the limit exists is defined to be the derivative off The derivative of fis d dy denoted by f x or f x or if y f x by or y The process of finding dx dx derivative of a function is called differentiation We also use the phrase differentiate f x with respect to x to mean find f x 3 The following rules were established as a part of algebra of deriva 1 ut v u v 2 uv u v uv Leibnitz or product rule lim h 0 120 u v uv wherever v 0 Quotient rule The following table gives a list of derivatives of rtain standard functions Table 5 3 its answer If lim h 0 f x 2 I f x f c h f c h f x lim h 0 Therefore MATHEMATICS sec x Whenever we defined derivative we had put a caution provided the limit exists Now the natural question is what if it doesn t The question is quite pertinent and so is f c h f c does not exist we say that f is not differentiable at c h In other words we say that a function f is differentiable at a point c in its domain if both are finite and equal A function is said to be differentiable in an interval a b if it is differentiable at every point of a b As in case of continuity at the end points a and b we take the right hand limit and left hand limit which are nothing but left hand derivative and right hand derivative of the function at a and b respectively Similarly a function is said to be differentiable in an interval a b if it is differentiable at every point of a b f x h f x h and lim h 0 But for x c we have sin x f c h f c h lim X C COS X Rationalised 2023 24 f x f c lim f x f c X C f x f c X C Theorem 3 If a function f is differentiable at a point c then it is also continuous at that point Proof Since f is differentiable at c we have blish C COS X lim X C sin x f x f c X C f c 560 3 x c f x f c X C tan x x c COT ed