Hence from 1 the d c s of the line are a b C a b c a b c a b

Last updated: 9/17/2023

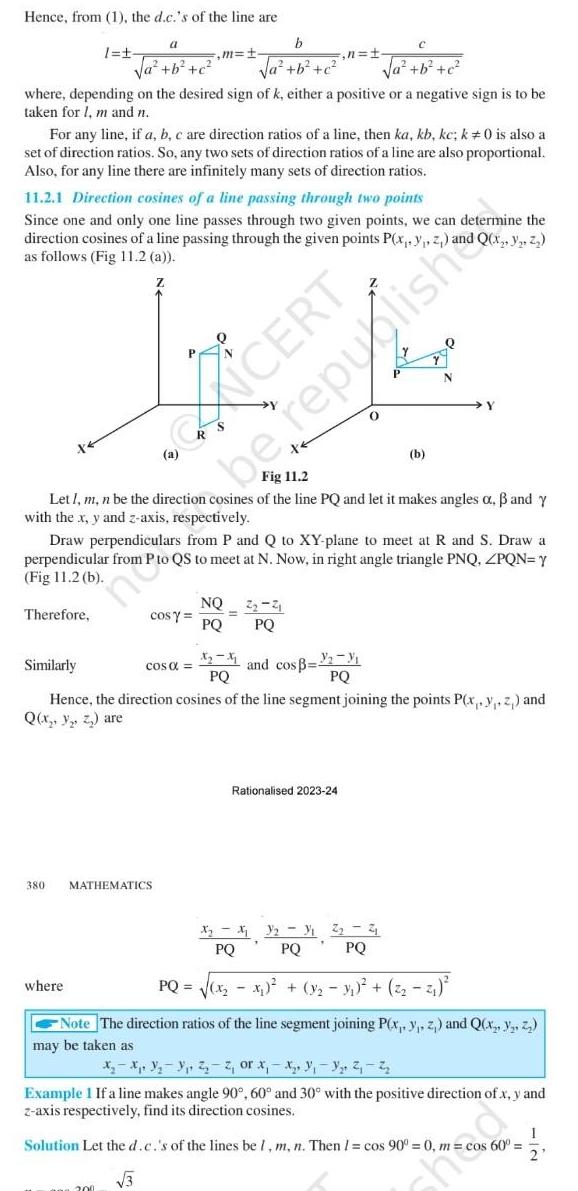
Hence from 1 the d c s of the line are a b C a b c a b c a b c where depending on the desired sign of k either a positive or a negative sign is to be taken for 1 m and n For any line if a b c are direction ratios of a line then ka kb kc k 0 is also a set of direction ratios So any two sets of direction ratios of a line are also proportional Also for any line there are infinitely many sets of direction ratios 1 t 11 2 1 Direction cosines of a line passing through two points Since one and only one line passes through two given points we can determine the direction cosines of a line passing through the given points P x y z and Q x Z as follows Fig 11 2 a J Therefore Let 1 m n be the direction cosines with the x y and z axis respectively 380 where m t cos y Draw perpendiculars from P and Q to XY plane to meet at R and S Draw a perpendicular from P to QS to meet at N Now in right angle triangle PNQ ZPQN Y Fig 11 2 b cos MATHEMATICS 200 CERT NO PQ n t 1 17 PQ PQ Similarly and cos Hence the direction cosines of the line segment joining the points P x y z and Q x y z are b of the line PQ and let it makes angles a and y N X PQ be repy fish Rationalised 2023 24 Q X X 3 3 2 2 PQ PQ PQ PQ x x 1 3 Note The direction ratios of the line segment joining P x y z and Q x may be taken as X X Y Y 2 z or x x 2 2 Example 1 If a line makes angle 90 60 and 30 with the positive direction of x y and z axis respectively find its direction cosines I Solution Let the d c s of the lines be 1 m n Then cos 90 0 m cos 60 2 3