i The lens is thin so that the distances measured from the poles of its two surfaces can be taken as equal to the distances from

Question

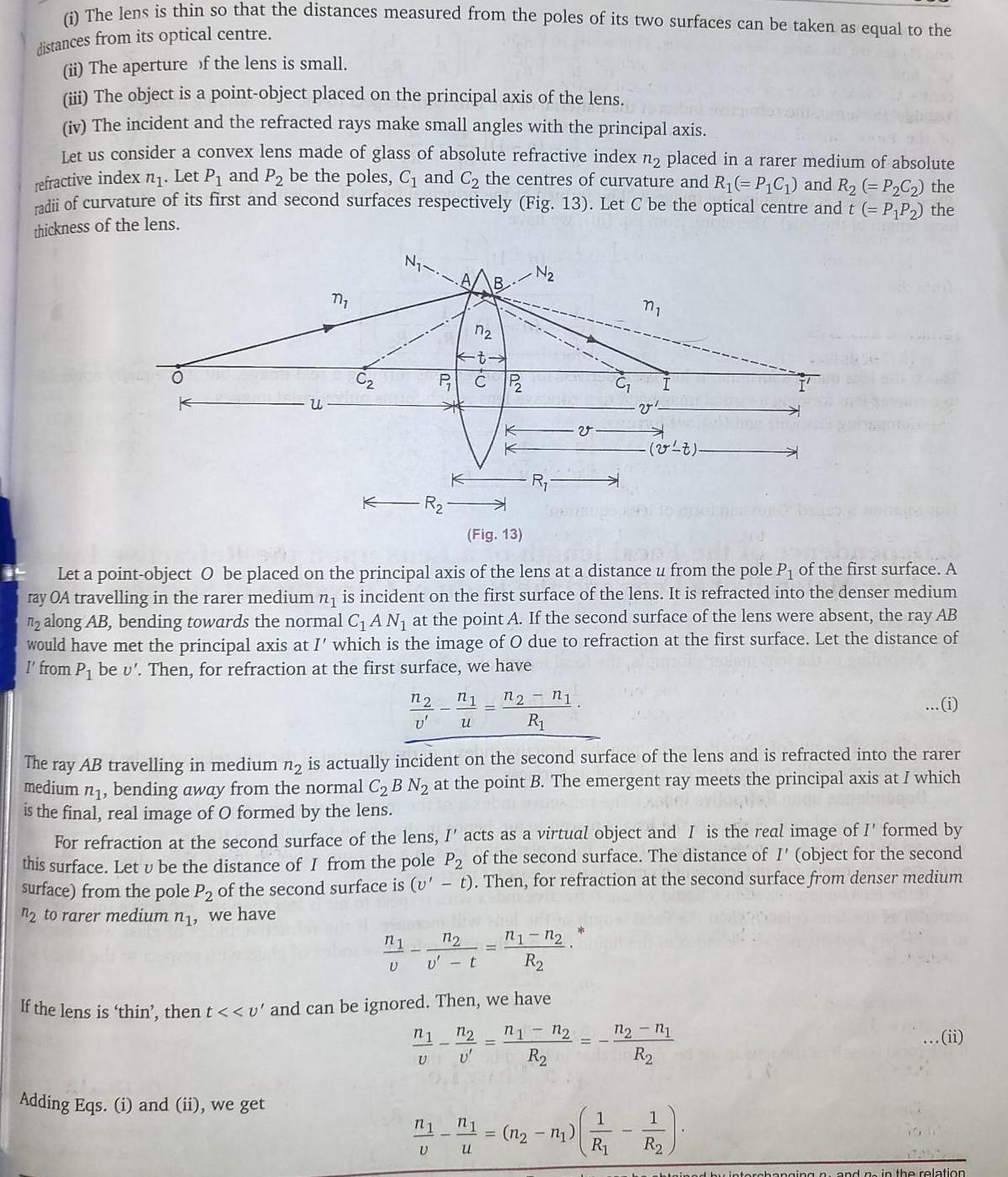
i The lens is thin so that the distances measured from the poles of its two surfaces can be taken as equal to the distances from its optical centre ii The aperture of the lens is small iii The object is a point object placed on the principal axis of the lens iv The incident and the refracted rays make small angles with the principal axis Let us consider a convex lens made of glass of absolute refractive index n2 placed in a rarer medium of absolute refractive index 1 Let P and P be the poles C and C the centres of curvature and R P C and R P C the radii of curvature of its first and second surfaces respectively Fig 13 Let C be the optical centre and t P P the thickness of the lens u m C K Adding Eqs i and ii we get R 1 n2 n1 V u NU Fig 13 Let a point object O be placed on the principal axis of the lens at a distance u from the pole P of the first surface A ray OA travelling in the rarer medium n is incident on the first surface of the lens It is refracted into the denser medium n along AB bending towards the normal C A N at the point A If the second surface of the lens were absent the ray AB would have met the principal axis at I which is the image of O due to refraction at the first surface Let the distance of I from P be u Then for refraction at the first surface we have 12 11 R n1 772 v t U i The ray AB travelling in medium n is actually incident on the second surface of the lens and is refracted into the rarer medium n bending away from the normal C B N at the point B The emergent ray meets the principal axis at I which is the final real image of O formed by the lens If the lens is thin then t u and can be ignored Then we have n1 122 n1 n U U R For refraction at the second surface of the lens I acts as a virtual object and I is the real image of I formed by this surface Let u be the distance of I from the pole P2 of the second surface The distance of I object for the second surface from the pole P2 of the second surface is v t Then for refraction at the second surface from denser medium 12 to rarer medium n we have 11 12 R v 11 n1 1 U u m v n v Z n 11 R2 11 12 R R ii btained by interchanging and no in the relation

Kunduz · Accepted Answer

Click to see the answer