VII 1 2 Calculate the residue at each singularity in the

Last updated: 6/4/2023

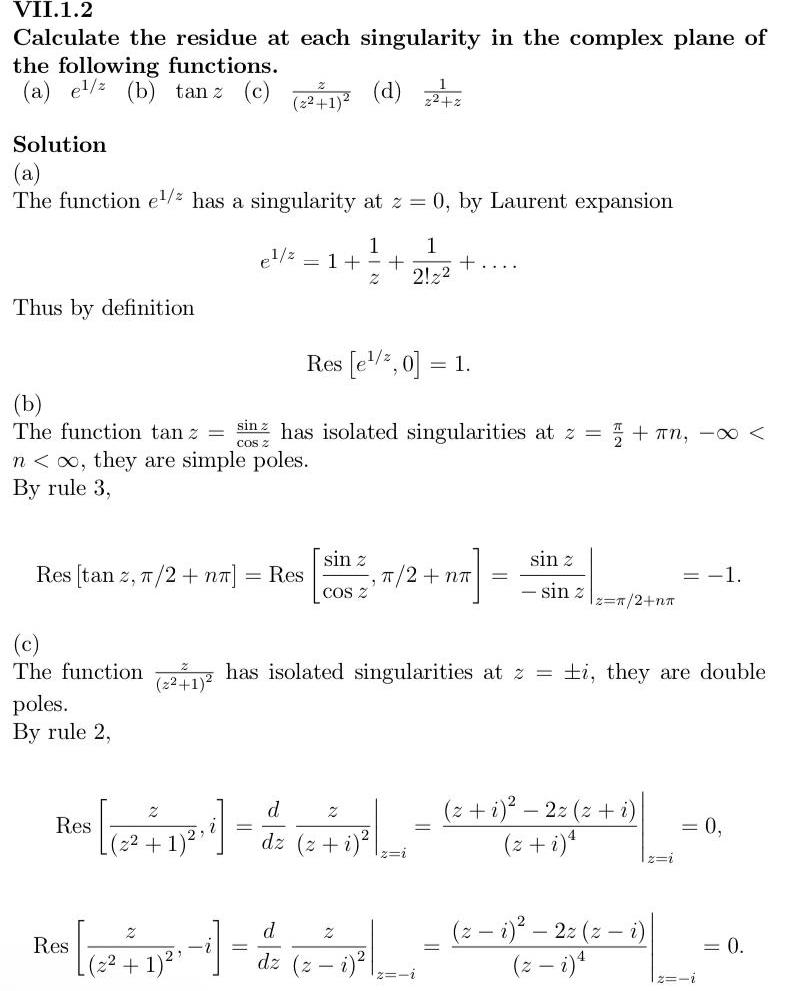
VII 1 2 Calculate the residue at each singularity in the complex plane of the following functions a e 2 b tanz c Thus by definition Solution a The function e 1 has a singularity at z 0 by Laurent expansion Res tan z 2 n c The function poles By rule 2 Res e 2 0 1 b sin has isolated singularities at z The function tan z n they are simple poles COS 2 By rule 3 22 1 Res d z 1 1 el z 1 2 D 1 Res 1 Z 2122 d sin z COS 2 z z 2 nT n L dz z i 2 1 has isolated singularities at z ti they are double d Z Res 2 1 2 1 2 0 i dz z i sin z sinz n z 2 nT z i 2z z i z i z i 2z z i z i 4 1 z i 0 2 1 0