When studying calculus, one of the fundamental concepts is finding the derivative of a function. The derivative measures how a function changes as its input variable changes. In this article, we will focus on the derivative of the natural logarithm function, ln x. The derivative of ln x is a key result in calculus and has many applications in various fields of science and mathematics.
What is ln?
Before diving into the derivative of ln x, let’s first understand what ln represents. Ln stands for the natural logarithm, which is a specific type of logarithm. The natural logarithm is defined as the logarithm with base e, where e is Euler’s number, approximately equal to 2.71828. The natural logarithm function is denoted by ln(x), where x is the input value.
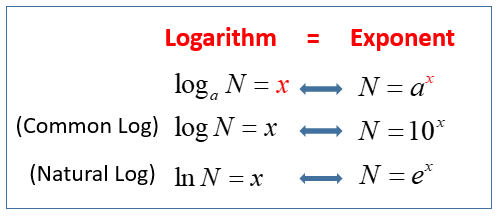
What is a Natural Logarithm?
A natural logarithm is a mathematical function that describes the time needed to reach a certain level of exponential growth. It is the inverse of the exponential function, where the base of the exponential function is equal to the value of Euler’s number, e. The natural logarithm function is widely used in various applications, such as exponential growth and decay, compound interest, and solving exponential equations.

What is ln x?
The natural logarithm function, ln x, is defined as the logarithm of x with base e. In other words, ln x represents the power to which e must be raised to obtain x. For example, ln(2) is the power to which e must be raised to obtain 2, which is approximately 0.69315.
What is the Derivative of ln x?
The derivative of ln x is a fundamental result in calculus. It represents the rate of change of the natural logarithm function with respect to its input variable, x. The derivative of ln x is equal to 1/x. In other words, if y = ln x, then dy/dx = 1/x.
The Derivative of the Natural Logarithmic Function
The derivative of the natural logarithmic function, ln x, can be derived using the first principle (definition of derivative) or the chain rule. The first principle approach involves taking the limit of the difference quotient as it approaches zero, while the chain rule approach involves differentiating the function using the chain rule.
To derive the derivative of ln x using the first principle, let’s assume that f(x) = ln x. By the definition of the derivative, the derivative of f(x), denoted as f'(x), is given by the limit:
f'(x) = lim(h→0) [f(x + h) – f(x)] / h
Substituting f(x) = ln x, we have f(x + h) = ln(x + h). Substituting these values into the definition of the derivative, we get:
f'(x) = lim(h→0) [ln(x + h) – ln x] / h
Using the property of logarithms, ln m – ln n = ln(m/n), we can simplify the above expression as:
f'(x) = lim(h→0) [ln((x + h) / x) ] / h
By letting h/x = t, we can rewrite the expression as:
f'(x) = lim(t→0) [ln(1 + t)] / (xt)
Using the property of logarithms again, ln am = m ln a, we can further simplify the expression as:
f'(x) = lim(t→0) (1/x) ln [(1 + t)1/t]
By applying one of the formulas of limits, lim(t→0) [(1 + t)1/t] = e, we finally obtain:
f'(x) = (1/x) ln e = (1/x)(1) = 1/x
Hence, we have proved that the derivative of ln x is 1/x using the definition of the derivative.
Using Properties of Logarithms in a Derivative
Properties of logarithms can be used to simplify the derivative of ln x before taking the derivative. These properties include:
- ln (ab) = ln a + ln b
- ln (a/b) = ln a – ln b
- ln (an) = n ln a
By applying these properties, we can expand an expression before finding the derivative, which can make the differentiation process easier. Let’s look at some examples:
Example 1: Find the derivative of f(x) = ln(x³ + 3x – 4)
Solution: Before applying the derivative, we can expand the expression using the properties of logarithms. We can write:
f(x) = ln(x³ + 3x – 4) = ln(x³) + ln(3x) – ln(4) = 3ln(x) + ln(3x) – ln(4)
Now, we can take the derivative of the expanded form of the function:
f'(x) = 3(1/x) + (1/(3x))(3) – 0 = 3/x + 1/x = 4/x
Hence, the derivative of ln(x³ + 3x – 4) is 4/x.
Example 2: Find the derivative of f(x) = ln(5x^4)
Solution: Before taking the derivative, we can expand this expression using the property of logarithms. Since the exponent is only on x, we can break this up as a product:
f(x) = ln(5x^4) = ln(5) + ln(x^4) = ln(5) + 4ln(x)
Now, we can take the derivative of the expanded form of the function:
f'(x) = 0 + 4(1/x) = 4/x
Therefore, the derivative of ln(5x^4) is 4/x.
Applying Derivative Formulas
In addition to using the properties of logarithms, we can also apply derivative formulas to find the derivative of ln x. Let’s look at some examples:
Example 1: Find the derivative of f(x) = ln(3x² + 5)
Solution: Since the argument of the natural logarithm is not just x, but instead is 3x² + 5, we cannot directly apply the basic rule for the derivative of the natural logarithm. Instead, we need to use the chain rule. The chain rule states that if y = f(g(x)), then the derivative of y with respect to x is given by:
dy/dx = f'(g(x)) * g'(x)
In this case, f(u) = ln u and g(x) = 3x² + 5. Taking the derivatives, we have:
f'(u) = 1/u g'(x) = 6x
Now, we can apply the chain rule to find the derivative of f(x):
f'(x) = f'(g(x)) * g'(x) = (1/(3x² + 5)) * 6x = 6x/(3x² + 5)
Therefore, the derivative of ln(3x² + 5) is 6x/(3x² + 5).
Example 2: Find the derivative of f(x) = ln((x² * sin x)/(2x + 1))
Solution: Before taking the derivative, we can simplify the expression using the properties of logarithms. We have:
f(x) = ln((x² * sin x)/(2x + 1)) = ln(x² * sin x) – ln(2x + 1) = 2ln(x) + ln(sin x) – ln(2x + 1)
Now, we can take the derivative of the expanded form of the function:
f'(x) = 2(1/x) + (1/sin x) * (cos x) – (1/(2x + 1))
Simplifying the expression further, we have:
f'(x) = 2/x + cos x/sin x – 1/(2x + 1)
Therefore, the derivative of ln((x² * sin x)/(2x + 1)) is 2/x + cos x/sin x – 1/(2x + 1).
Derive an Equation for the Derivative of ln x
To derive an equation for the derivative of ln x, we can use the definition of the derivative and the properties of logarithms. Let’s go through the derivation step by step:
Step 1: Start with the definition of the derivative:
f'(x) = lim(h→0) [f(x + h) – f(x)] / h
Step 2: Substitute f(x) = ln x:
f'(x) = lim(h→0) [ln(x + h) – ln x] / h
Step 3: Use the property of logarithms, ln m – ln n = ln(m/n):
f'(x) = lim(h→0) [ln((x + h) / x)] / h
Step 4: Simplify the expression:
f'(x) = lim(h→0) [ln(1 + h/x)] / h
Step 5: Substitute t = h/x:
f'(x) = lim(t→0) [ln(1 + t)] / (xt)
Step 6: Use the property of logarithms, ln am = m ln a:
f'(x) = lim(t→0) (1/x) ln [(1 + t)1/t]
Step 7: Use the limit formula, lim(t→0) [(1 + t)1/t] = e:
f'(x) = (1/x) ln e = (1/x)(1) = 1/x
Hence, we have derived the equation for the derivative of ln x, which is 1/x.
How to Calculate the Derivative of ln x
To calculate the derivative of ln x, you can use the derivative formula for the natural logarithm function. The derivative of ln x is 1/x. This means that if you have a function y = ln x, then the derivative dy/dx is equal to 1/x.
To calculate the derivative of ln x, follow these steps:
- Identify the function you want to differentiate. In this case, the function is y = ln x.
- Apply the derivative formula for ln x, which is dy/dx = 1/x.
- Simplify the expression, if needed.
For example, let’s say you want to find the derivative of the function y = ln(2x). Applying the derivative formula, we have dy/dx = 1/(2x). Simplifying, we get dy/dx = 1/(2x).
Therefore, the derivative of ln(2x) is 1/(2x).
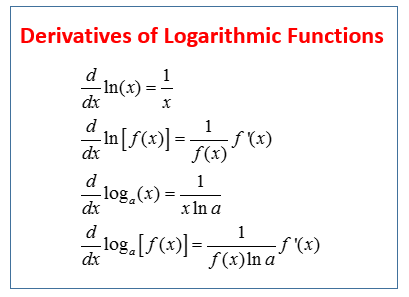
ln Derivative Rules
The derivative of ln x follows a simple rule: the derivative of ln x is 1/x. This means that if you have a function y = ln x, then the derivative dy/dx is equal to 1/x.
However, it is important to note that the derivative of ln x is different from the derivative of log x. The derivative of log x is 1/(x ln 10), while the derivative of ln x is 1/x.
For readers exploring the intricacies of the derivative of ln(x), our differentiation of trigonometric functions page serves as an invaluable resource. It provides essential insights into the calculus of trigonometric expressions, offering a comprehensive understanding that enhances the exploration of logarithmic derivatives and their applications in mathematical analysis.
Derivative of ln x Proof by First Principle Rule
The derivative of ln x can be proven using the first principle, which is the definition of the derivative. The first principle states that the derivative of a function f(x) at a point x is given by the limit:
f'(x) = lim(h→0) [f(x + h) – f(x)] / h
To prove the derivative of ln x using the first principle, let’s assume that f(x) = ln x.
By the definition of the derivative, we have:
f'(x) = lim(h→0) [ln(x + h) – ln x] / h
Using the property of logarithms, ln m – ln n = ln(m/n), we can simplify the expression as:
f'(x) = lim(h→0) [ln((x + h) / x) ] / h
By letting h/x = t, we can rewrite the expression as:
f'(x) = lim(t→0) [ln(1 + t)] / (xt)
Using another property of logarithms, ln am = m ln a, we can further simplify the expression as:
f'(x) = lim(t→0) ln [(1 + t)1/t]
Since the limit of (1 + t)1/t as t approaches 0 is equal to e, we can substitute e for the limit:
f'(x) = ln e = 1
Therefore, the derivative of ln x is equal to 1.
Derivative of ln x Proof by Chain Rule
The derivative of ln x can also be proven using the chain rule. The chain rule states that if y = f(g(x)), then the derivative of y with respect to x is given by:
dy/dx = f'(g(x)) * g'(x)
To prove the derivative of ln x using the chain rule, let’s consider the function y = ln x.
We can rewrite this function as y = ln(g(x)), where g(x) = x.
Now, we can apply the chain rule to find the derivative of y with respect to x:
dy/dx = f'(g(x)) * g'(x)
In this case, f(u) = ln u and g(x) = x. Taking the derivatives, we have:
f'(u) = 1/u g'(x) = 1
Substituting these into the chain rule, we get:
dy/dx = (1/x) * 1 = 1/x
Therefore, the derivative of ln x is equal to 1/x.
Graphical Deduction of the Formula For the Derivative of ln(x)
To deduce the formula for the derivative of ln(x) graphically, we can observe the slope of the tangent line to the graph of ln(x) at different points.
The graph of ln(x) is increasing on the interval (0, +∞). This means that as x increases, the value of ln(x) also increases.
By observing the slope of the tangent line at different points on the graph, we can deduce that the slope of the tangent line at any point x is equal to 1/x.
For example, at x = 1, the slope of the tangent line is 1. At x = 2, the slope of the tangent line is 1/2. At x = 3, the slope of the tangent line is 1/3, and so on.
Therefore, we can deduce that the derivative of ln(x) is equal to 1/x.
What is the Domain of the Derivative of ln(x)?
The derivative of ln(x) is defined for all values of x in its domain. The natural logarithm function, ln(x), is defined only for positive values of x. Therefore, the domain of the derivative of ln(x) is also restricted to positive values of x. In other words, the derivative of ln(x) is defined for x > 0.
Differentiation of ln x by Implicit Differentiation
Another method to find the derivative of ln x is by using implicit differentiation. Implicit differentiation allows us to differentiate a function that is defined implicitly, where the dependent and independent variables are not explicitly expressed.
To differentiate ln x using implicit differentiation, let’s consider the equation y = ln x.
We can rewrite this equation as e^y = x, where e is Euler’s number.
Now, we can differentiate both sides of the equation with respect to x:
d/dx (e^y) = d/dx (x)
Using the chain rule on the left side, we get:
(e^y)(dy/dx) = 1
Simplifying the equation, we have:
dy/dx = 1/(e^y)
Since y = ln x, we can substitute ln x for y:
dy/dx = 1/(e^(ln x))
Using the property e^(ln x) = x, we can further simplify the equation:
dy/dx = 1/x
Therefore, the derivative of ln x, obtained through implicit differentiation, is 1/x.
nth Derivative of ln x
To find the nth derivative of ln x, we can apply the power rule for derivatives. The power rule states that if y = x^n, then the derivative of y with respect to x is given by:
d^n y/dx^n = n(n-1)(n-2)…(n-k+1)x^(n-k)
For ln x, the exponent is equal to 1. Therefore, the nth derivative of ln x is given by:
d^n ln x/dx^n = (n-1)!
where ! denotes the factorial function.
For example, the first derivative of ln x is 1/x, the second derivative is -1/x², the third derivative is 2/x³, and so on.
Therefore, the nth derivative of ln x is given by d^n ln x/dx^n = (n-1)!/x^n.
Solved Examples on Derivative of ln(x)
Let’s solve some examples to further illustrate the derivative of ln(x):
Example 1: Find the derivative of f(x) = ln(2x).
Solution: Applying the derivative formula, we have f'(x) = 1/(2x).
Example 2: Find the derivative of f(x) = ln(x²).
Solution: Applying the derivative formula, we have f'(x) = 2/x.
Example 3: Find the second derivative of f(x) = ln(x³).
Solution: The first derivative of f(x) is f'(x) = 3/x. Applying the derivative formula again, we have f”(x) = -3/x².
These examples demonstrate the application of the derivative formula for ln(x) in finding the first and second derivatives.
How Kunduz Can Help You Learn Derivatives of ln(x)?
If you want to learn more about derivatives of ln(x) and other calculus concepts, Kunduz is here to help. Kunduz is an online learning platform that offers comprehensive and interactive courses on mathematics and physics. Our courses are designed to help students of all levels understand complex concepts and improve their problem-solving skills.
With Kunduz, you can access video lessons, practice problems, and interactive quizzes to enhance your understanding of derivatives of ln(x) and other calculus topics. Our experienced instructors will guide you through each step, providing clear explanations and real-world examples to make the learning process engaging and enjoyable.
Whether you are a high school student preparing for exams or a college student studying advanced calculus, Kunduz has the resources you need to succeed. Join Kunduz today and unlock your full potential in mathematics and physics.
