The derivative of a function measures its rate of change with respect to the independent variable. In calculus, the derivative of the sine function, sin x, is a fundamental concept. It represents the rate of change of sin x with respect to the angle x. The derivative of sin x is denoted as d/dx (sin x) or sin'(x) and is equal to cos x.
Understanding the derivative of sin x is crucial in various fields, including mathematics, physics, engineering, and economics. It allows us to analyze the behavior of sinusoidal functions, solve differential equations, and optimize functions involving trigonometry.
In this article, we will delve into the concept of the derivative of sin x, explore its various formulas such as the quotient rule, chain rule, and first principle method, provide proofs for these formulas, and present solved examples to solidify our understanding.
What is Derivative of Sin X?
The derivative of sin x represents the rate of change of the sine function with respect to the angle x. It provides information about how quickly the value of sin x is changing as the angle x varies. The derivative of sin x is equal to cos x, which means that the rate of change of sin x at any given angle x is given by the cosine of that angle.
Mathematically, the derivative of sin x can be represented as d/dx (sin x) = cos x or (sin x)’ = cos x. This formula allows us to calculate the derivative of sin x at any point on the sine curve.
What is Sine?
Before we dive into the derivative of sin x, let’s briefly discuss the sine function itself. The sine function, sin x, is a trigonometric function that relates the ratio of the length of the side opposite an angle in a right triangle to the length of the hypotenuse. In other words, it represents the vertical displacement of a point on the unit circle as the angle x increases.
The sine function is periodic, with a period of 2π. It oscillates between the values of -1 and 1 as the angle x varies. The graph of sin x is a smooth, periodic wave that repeats itself every 2π units along the x-axis. The graph exhibits symmetry about the origin, as sin (-x) = -sin x.

Derivative of Sin X Formula
The derivative of sin x can be determined using various methods, including the first principle method, chain rule, and quotient rule. Each of these methods provides a different approach to finding the derivative, but they all yield the same result: cos x.
Derivative of Sin X using the First Principle Method
The first principle method, also known as the limit definition of the derivative, allows us to find the derivative of a function by evaluating the limit of the difference quotient as the interval approaches zero. To find the derivative of sin x using the first principle method, we start by applying the limit definition:
lim(h → 0) [f(x + h) - f(x)] / h
Let’s apply this definition to the function f(x) = sin x:
lim(h → 0) [sin(x + h) - sin x] / h
Using the sum-to-product formula for sine, sin(A + B) = sin A cos B + cos A sin B, we can rewrite the numerator:
lim(h → 0) [sin x cos h + cos x sin h - sin x] / h
Next, we can separate the terms and simplify the expression:
lim(h → 0) [sin x (cos h - 1) + cos x sin h] / h
By factoring out sin x and applying the limit properties, we can further simplify:
sin x (lim(h → 0) (cos h - 1) / h) + cos x (lim(h → 0) sin h / h)
Using the limit properties, we find that lim(h → 0) (cos h – 1) / h = 0 and lim(h → 0) sin h / h = 1. Therefore, the derivative of sin x using the first principle method is:
sin x (0) + cos x (1) = cos x
Hence, we have derived the formula for the derivative of sin x as cos x using the first principle method.
Derivative of Sin X Proof by Chain Rule
The chain rule is a powerful rule in calculus that allows us to find the derivative of composite functions. To find the derivative of sin x using the chain rule, we need to express sin x as a composition of functions. We can rewrite sin x as cos(π/2 – x) using a trigonometric identity. Applying the chain rule, we find:
d/dx (sin x) = d/dx (cos(π/2 - x))
By differentiating the outer function and the inner function, we get:
d/dx (sin x) = -sin(π/2 - x) * d/dx (π/2 - x)
Simplifying further, we find:
d/dx (sin x) = -sin(π/2 - x)
Using the trigonometric identity sin(π/2 – x) = cos x, we obtain:
d/dx (sin x) = cos x
Thus, we have proved the derivative of sin x using the chain rule to be cos x.
Derivative of Sin X Proof by Quotient Rule
The quotient rule provides a method for finding the derivative of a function that is a quotient of two functions. To find the derivative of sin x using the quotient rule, we can rewrite sin x as 1/csc x. Applying the quotient rule, we have:
d/dx (sin x) = [d/dx (1)] * csc x - 1 * [d/dx (csc x)] / (csc x)^2
Simplifying further, we get:
d/dx (sin x) = -csc x * cot x
Using the trigonometric identities csc x = 1/sin x and cot x = cos x/sin x, we can rewrite the derivative as:
d/dx (sin x) = -cos x
Therefore, we have proved the derivative of sin x using the quotient rule to be -cos x.
LIATE Rule
The LIATE rule is a helpful mnemonic device to determine which function to differentiate first when applying the chain rule. It stands for Logarithmic, Inverse trigonometric, Algebraic, Trigonometric, and Exponential functions. When differentiating a composite function, the LIATE rule suggests differentiating the functions in the order of the letters in LIATE.
When applying the LIATE rule to the function sin^2(x), we can differentiate the outer function (squared function) first and then differentiate the inner function (sine function). This approach yields the derivative of sin^2(x) as:
d/dx (sin^2(x)) = 2sin(x)cos(x)
Hence, the derivative of sin^2(x) is 2sin(x)cos(x) using the LIATE rule.
Proof of Derivative of Sin X
The derivative of sin x can be proven using various methods, such as the first principle method, chain rule, and quotient rule. Each of these methods provides a different perspective on the derivative of sin x, but they all arrive at the same result: cos x.
Using the first principle method, we can evaluate the limit of the difference quotient as the interval approaches zero. By applying the sum-to-product formula for sine, we can simplify the expression and find that the derivative of sin x is cos x.
Alternatively, using the chain rule, we can express sin x as a composite function and differentiate the outer and inner functions separately. By simplifying the expression, we arrive at the same result: the derivative of sin x is cos x.
Similarly, applying the quotient rule allows us to rewrite sin x as a quotient and differentiate the numerator and denominator separately. By simplifying the expression, we once again find that the derivative of sin x is cos x.
These different proofs provide multiple perspectives on the derivative of sin x and demonstrate the consistency of the result: the derivative of sin x is cos x.
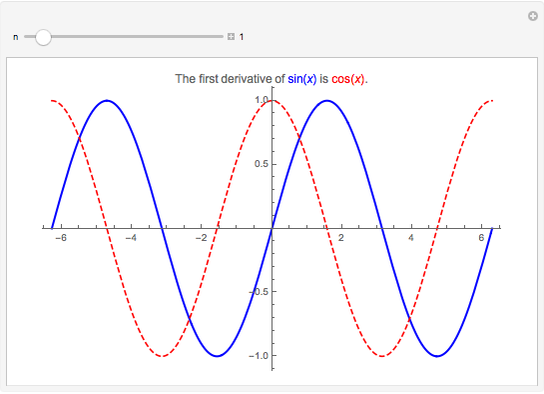
Graph of Sin X and Derivative of Sin X
The graph of sin x is a smooth, periodic wave that oscillates between the values of -1 and 1 as the angle x varies. It repeats itself every 2π units along the x-axis and exhibits symmetry about the origin.
The derivative of sin x, cos x, represents the rate of change of the sine function at any given point. The graph of the derivative, cos x, is a periodic function that oscillates between the values of -1 and 1 as x varies. It is also symmetric about the origin, similar to the graph of sin x.
The graph of sin x and its derivative, cos x, are closely related. The peaks and valleys of the sine curve correspond to the zeros of the cosine curve, and vice versa. As the angle x increases, the value of sin x oscillates between -1 and 1, while the value of cos x oscillates between 1 and -1.
By analyzing the graph of sin x and its derivative, we gain insights into the behavior of the sine function and its rate of change. We can see how the slope of the sine curve varies as x changes, and how it relates to the values of cos x.
Derivative of the Composite Function Sin(u(x))
The derivative of a composite function, such as sin(u(x)), can be found using the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function multiplied by the derivative of the inner function.
To find the derivative of sin(u(x)), we differentiate the outer function (sine function) and the inner function separately, and then multiply the results. Let’s denote the derivative of u(x) as u'(x) and the derivative of sin x as cos x. Then, the derivative of sin(u(x)) is given by:
d/dx (sin(u(x))) = cos(u(x)) * u'(x)
This formula allows us to find the derivative of any composite function involving the sine function. By substituting the appropriate expressions for u(x) and u'(x), we can evaluate the derivative of sin(u(x)).
Nth derivative of Sin X
The nth derivative of sin x can be found by repeatedly applying the derivative operator to the sine function. Each time we differentiate sin x, the result is cos x. Therefore, the first derivative of sin x is cos x.
Applying the derivative operator again to cos x, we find that the second derivative of sin x is equal to the derivative of cos x, which is -sin x. Continuing this process, we can find the third derivative, fourth derivative, and so on.
The pattern of the derivatives of sin x is as follows:
- First derivative: cos x
- Second derivative: -sin x
- Third derivative: -cos x
- Fourth derivative: sin x
- Fifth derivative: cos x
- Sixth derivative: -sin x
- And so on
We can see that the derivatives of sin x repeat in a cyclical manner, with the cosine and sine functions alternating. This pattern reflects the periodic nature of the sine function itself.
Anti-Derivative of Sin X
The anti-derivative, or integral, of sin x can be found by reversing the process of differentiation. The integral of sin x is denoted as ∫ sin x dx and represents the set of functions whose derivative is sin x.
The anti-derivative of sin x is equal to -cos x plus a constant of integration. In mathematical notation, we can express this as:
∫ sin x dx = -cos x + C
where C is the constant of integration. This formula allows us to find the integral of sin x for any given interval.
The integral of sin x is closely related to the anti-derivative of cos x, which is equal to sin x plus a constant of integration. These two formulas are fundamental in calculus and are used to solve various problems involving trigonometric functions.
Solved Examples on Derivative of Sin X
Example 1:
Find the derivative of sin^2 x.
Solution:
Let y = sin^2 x
By using the power rule of differentiation, we get:
y’ = 2sinx (cosx) = 2sinxcosx
This is the derivative of sin^2 x.
Example 2:
Find the derivative of the function sin(3x^2).
Solution:
Let y = sin(3x^2)
By using the chain rule of differentiation, we get:
y’ = cos(3x^2) * 6x = 6xcos(3x^2)
This is the derivative of sin(3x^2).
How Kunduz Can Help You Learn Derivatives of Sin X?
Kunduz is a comprehensive online learning platform that offers a wide range of resources to help you learn and understand the derivative of sin x. With Kunduz, you can access interactive lessons, video tutorials, practice problems, and personalized feedback to enhance your understanding of calculus concepts.
Whether you’re a student looking to improve your grades or a professional seeking to refresh your knowledge, Kunduz provides a user-friendly interface and engaging learning materials to support your learning journey. With our step-by-step explanations and real-world examples, you can master the concepts of differentiation and apply them to various problem-solving scenarios.
Kunduz also offers a supportive community of learners and experienced instructors who are dedicated to helping you succeed. You can interact with fellow students, ask questions, and receive guidance from experts in the field.
