(a) Show that the vectors v1 = (1,2,1,0), v2 = (2,6,0,2) and

Last updated: 7/25/2022

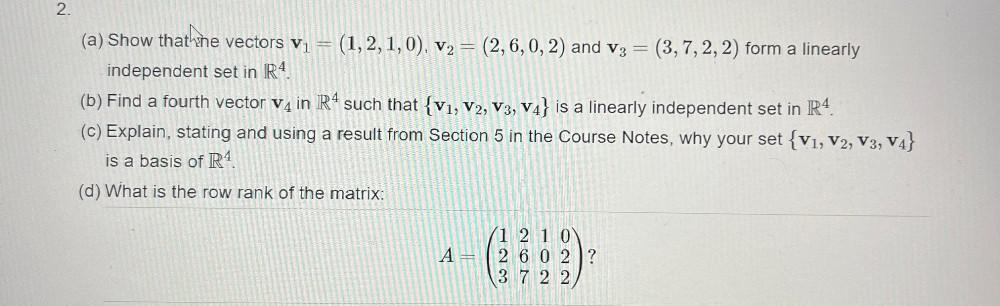
(a) Show that the vectors v1 = (1,2,1,0), v2 = (2,6,0,2) and v3 = (3,7,2,2) form a linearly independent set in R^4. (b) Find a fourth vector v4 in R^4 such that {V1, V2, V3, V4} is a linearly independent set in R^4. (c) Explain, stating and using a result from Section 5 in the Course Notes, why your set {v1, v2, v3, v4} is a basis of R4. (d) What is the row rank of the matrix: A= 1 2 1 0 2 6 0 2 3 7 2 2