Find the point of inflection and discuss the concavity of

Last updated: 7/17/2022

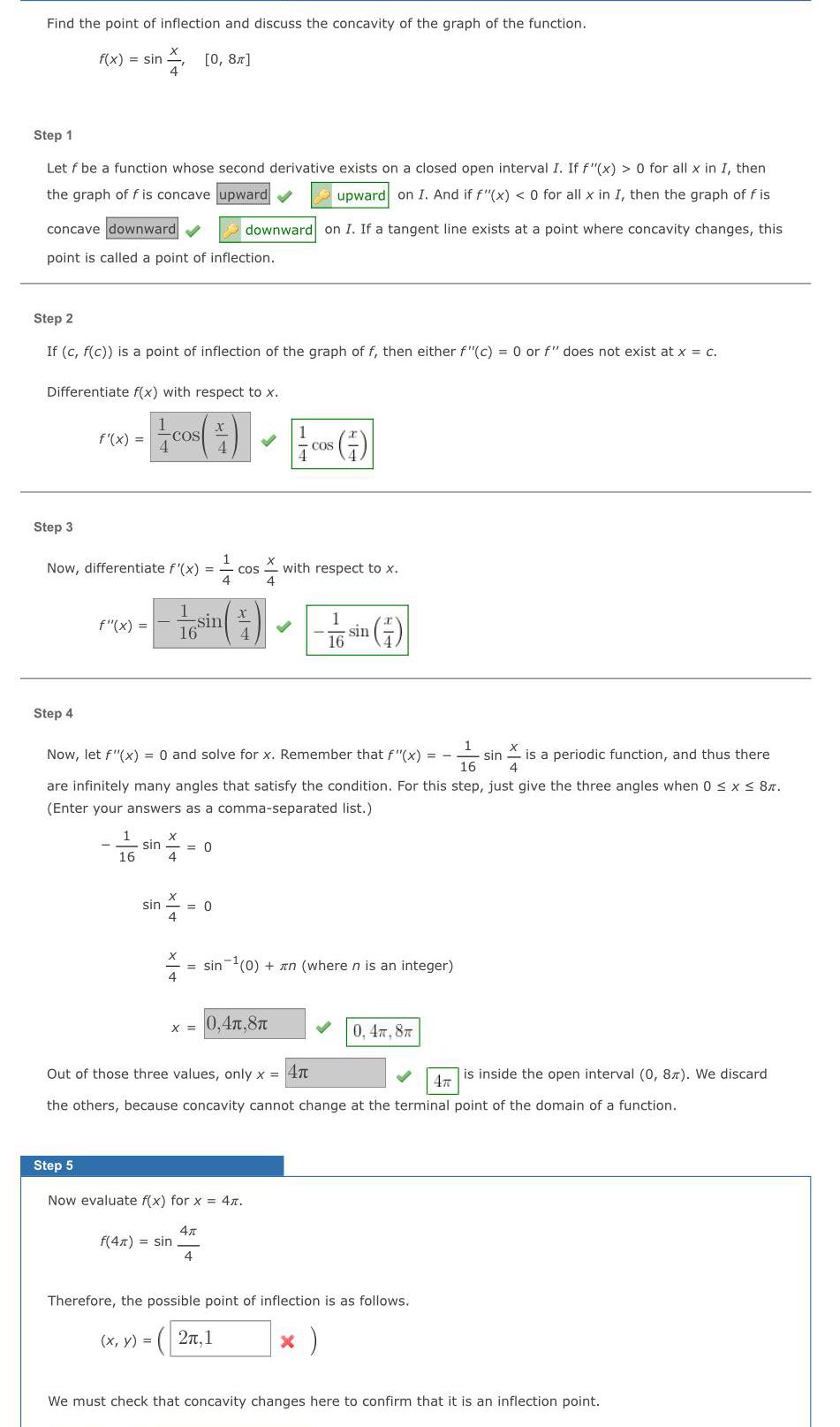
Find the point of inflection and discuss the concavity of the graph of the function. f(x) = sin [0, 8π] Let f be a function whose second derivative exists on a closed open interval I. If f"(x) > 0 for all x in I, then the graph of f is concave upward upward on I. And if f"(x) < 0 for all x in I, then the graph of fis downward on I. If a tangent line exists at a point where concavity changes, this concave downward point is called a point of inflection. If (c, f(c)) is a point of inflection of the graph of f, then either f"(c) = 0 or f" does not exist at x = c. Differentiate f(x) with respect to x.