The cubic polynomial equation x³ + bx² + cx + d = 0 has three roots X₁, X2, and X3. By expanding the product (x-x₁)(x - x₂)(x -

Question

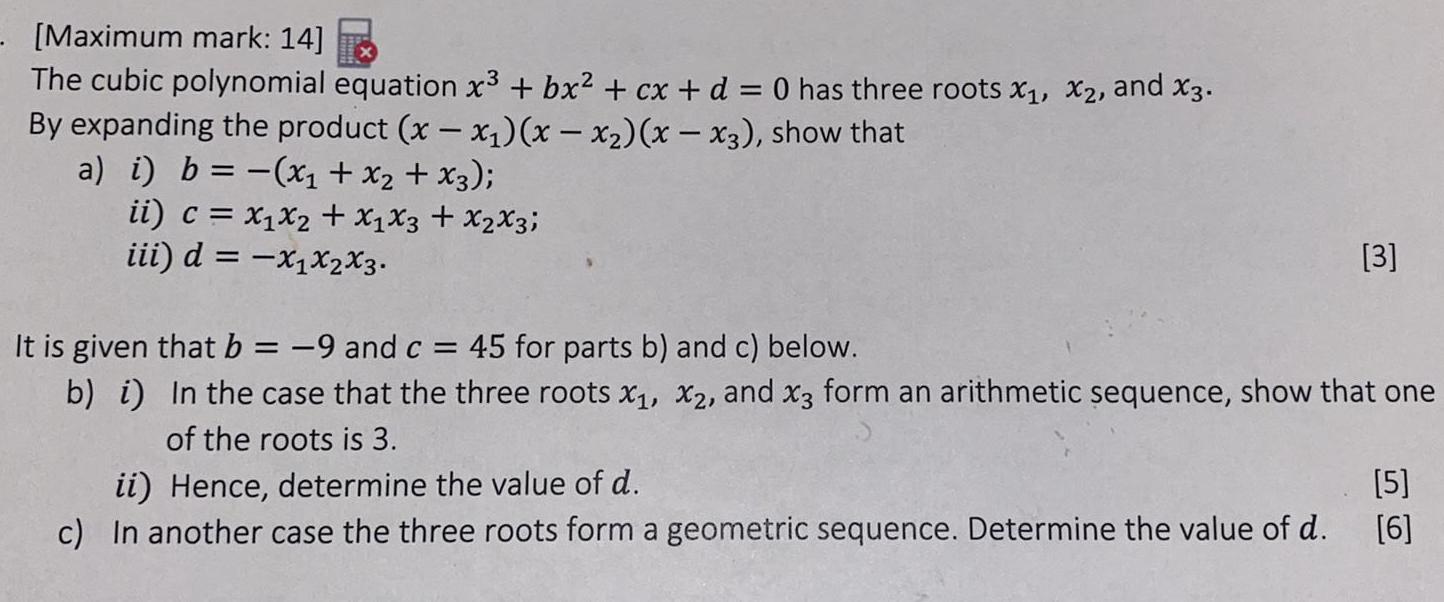
The cubic polynomial equation x³ + bx² + cx + d = 0 has three roots X₁, X2, and X3.
By expanding the product (x-x₁)(x - x₂)(x - x3), show that
a) i) b = (x₁ + x₂ + x3);
ii) c = x₁x₂ + x₁ x3 + x2X3;
iii) d = -X₁X2X3.
It is given that b = -9 and c = 45 for parts b) and c) below.
b) i) In the case that the three roots x₁, x2, and x3 form an arithmetic sequence, show that one
of the roots is 3.
ii) Hence, determine the value of d.
c) In another case the three roots form a geometric sequence. Determine the value of d.

Kunduz · Accepted Answer

Click to see the answer