Polynomial long division is an essential method in algebra for dividing one polynomial by another. It allows us to simplify complex expressions, find factors, and solve equations involving polynomials. In this article, we will explore the definition, methods, and steps involved in polynomial long division. We will also discuss long division with monomials and binomials, as well as provide solved examples to illustrate the process. So, let’s dive into the world of polynomial long division!
An Introduction to Polynomial Long Division
Polynomials are algebraic expressions that consist of variables and coefficients combined using addition, subtraction, multiplication, and exponentiation. A polynomial can have one or more terms, and each term may contain multiple variables raised to different powers. Polynomial long division is a method used to divide a polynomial by another polynomial of lower degree.
What is a Polynomial?
Before we delve into polynomial long division, let’s first understand what a polynomial is. A polynomial is an algebraic expression that includes real numbers, variables, and coefficients. It is composed of various terms, and each term consists of a coefficient multiplied by one or more variables raised to specific powers.
There are different types of polynomials based on the number of terms they have. A monomial is a polynomial with only one term, a binomial has two terms, and a trinomial has three terms.
What is Polynomial Long Division?
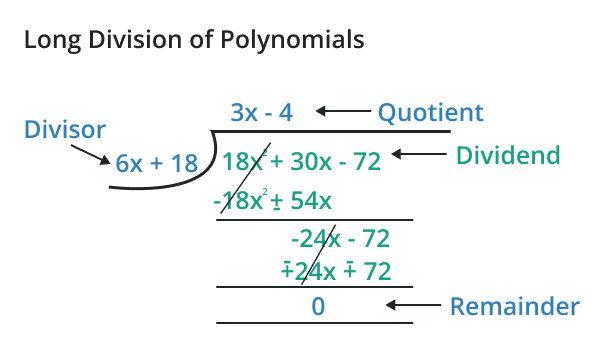
Polynomial long division is an algorithm used to divide one polynomial by another polynomial. It involves the division of the polynomial into smaller parts, resulting in a quotient and a remainder. The process is similar to long division of numbers, where we divide, multiply, subtract, and bring down terms until we reach a remainder of lower degree than the divisor.
The long division process is based on the fact that every polynomial can be expressed as the product of the divisor and the quotient added to the remainder. By dividing polynomials, we can simplify expressions, find factors, and solve equations.
Polynomial Long Division Methods
There are different methods for performing polynomial long division, depending on the specific scenario. Let’s explore some of the common methods:
Splitting the Terms Method
In the splitting the terms method, we divide the polynomial by grouping and splitting the terms. This method is useful when the dividend and divisor have similar terms. We divide each term individually and simplify the expression to find the quotient and remainder.
Factorization Method
The factorization method involves factoring the polynomial to simplify the division process. By factoring the dividend and divisor, we can cancel out common factors and simplify the expression. This method is particularly useful when the divisor is a factor of the dividend.
Long Division of Polynomial by Missing Terms
In some cases, there may be missing terms in the polynomial expression. When performing long division in such scenarios, we can either leave a gap while dividing or consider the missing terms as having a coefficient of zero. This allows us to divide the polynomial accurately and obtain the quotient and remainder.
Long Division of Polynomials by Monomials
When dividing a polynomial by a monomial, we divide each term of the polynomial individually by the monomial. This method involves dividing the coefficients and subtracting the exponents of the variables. By simplifying the division process term by term, we can find the quotient and remainder.
Long Division of Polynomials by Binomials
Long division of polynomials by binomials is similar to long division of numbers. When there are no common factors between the dividend and divisor, or if we cannot identify the factors, we use this method. By following the long division algorithm step by step, we can divide the polynomial by the binomial and obtain the quotient and remainder.
Long Division of Polynomials with Remainders
In polynomial long division, it is possible to have a remainder. The remainder is the term left over after dividing the dividend by the divisor. If the remainder is zero, it means that the divisor divides evenly into the dividend. If the remainder is nonzero, it indicates that the divisor is not a factor of the dividend.
Steps For Long Division of Polynomials
The long division of polynomials follows a systematic step-by-step process. Let’s outline the general steps involved:
- Arrange the terms of the dividend and divisor in descending order of their degrees.
- Divide the first term of the dividend by the first term of the divisor to obtain the first term of the quotient.
- Multiply the divisor by the quotient term to obtain the product.
- Subtract the product from the dividend, and bring down the next term if any.
- Repeat steps 2-4 until you reach a remainder of lower degree than the divisor.
- Express the remainder as a fraction using the divisor as the denominator, if necessary.
By following these steps, we can perform polynomial long division and obtain the quotient and remainder.
Long Division Algorithm of Polynomials
The long division algorithm of polynomials provides a systematic approach to dividing polynomials. It ensures that the division is performed correctly and yields accurate results. The algorithm involves arranging the terms, dividing, multiplying, subtracting, and bringing down terms until the division process is complete.
The long division algorithm is based on the concept of dividing the polynomial into smaller parts and simplifying the expression step by step. It allows us to find the quotient and remainder, which is useful for various mathematical computations involving polynomials.
Types of Polynomials
Polynomials can be classified into different types based on the number of terms they have. Let’s explore some common types of polynomials:
Monomial
A monomial is a polynomial with only one term. It consists of a coefficient multiplied by one or more variables raised to specific powers. Monomials are the simplest form of polynomials and can be constants or products of constants and variables.
Binomials
A binomial is a polynomial with two terms. It consists of two monomials separated by an addition or subtraction operator. Binomials can be expressed in the form of “ax + b” or “a – bx,” where “a” and “b” are coefficients and “x” is a variable.
Trinomials
A trinomial is a polynomial with three terms. It consists of three monomials separated by addition or subtraction operators. Trinomials can be expressed in the form of “ax² + bx + c,” where “a,” “b,” and “c” are coefficients and “x” is a variable.
Understanding the types of polynomials helps us identify their characteristics and perform operations such as addition, subtraction, multiplication, and division more effectively.
Solved Examples on Polynomial Long Division
To illustrate the process of polynomial long division, let’s work through some solved examples:
Example 1: Divide the polynomial 3x^3 – 5x^2 + 2x + 1 by the polynomial x – 1.
Solution: We start by dividing the highest degree term 3x^3 by x to obtain 3x^2. Then, we multiply (x – 1) by 3x^2 to get 3x^3 – 3x^2, which is subtracted from the original polynomial. We continue this process, dividing the highest degree term of the new dividend by the highest degree term of the divisor at each step. Finally, we obtain the quotient 3x^2 + 2x + 3 and the remainder 4.
Example 2: Divide the polynomial 4x^3 – 10x^2 + 5x by the monomial 2x.
Solution: In this example, we divide each term of the polynomial individually by 2x. Dividing 4x^3 by 2x gives us 2x^2. Dividing -10x^2 by 2x gives us -5x. Dividing 5x by 2x gives us 2.5. So, the quotient is 2x^2 – 5x + 2.5.
These examples demonstrate how polynomial long division is performed step by step to obtain the quotient and remainder.
How Kunduz Can Help You Learn Polynomial Long Division?
At Kunduz, we understand the importance of mastering polynomial long division. It is a fundamental concept in algebra and serves as a foundation for various mathematical calculations. We offer comprehensive courses and resources designed to help you understand and apply polynomial long division effectively.
So, whether you’re a student looking to improve your math skills or a teacher seeking additional resources, Kunduz is here to assist you. Join us today and unlock your potential in polynomial long division and other mathematical concepts.
