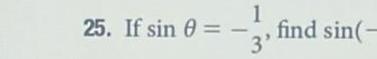Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

College Math - Others
Functions2 Use an Euler diagram to determine whether the argument is valid or invalid b No rocker would do the mariachi All baseball fans do the mariachi No rocker is a baseball fan
College Math - Others
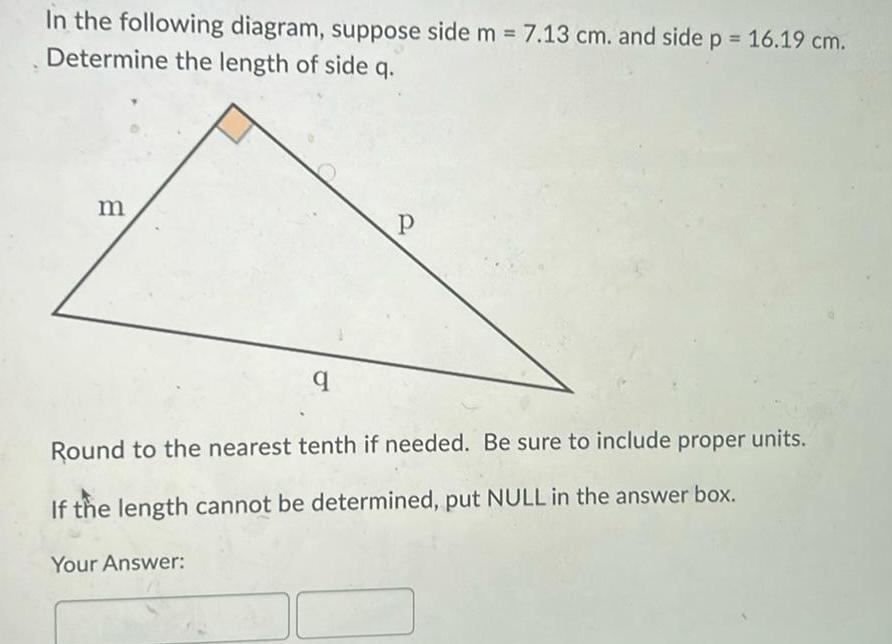
Basic MathIn the following diagram suppose side m 7 13 cm and side p 16 19 cm Determine the length of side q m q Your Answer P Round to the nearest tenth if needed Be sure to include proper units If the length cannot be determined put NULL in the answer box
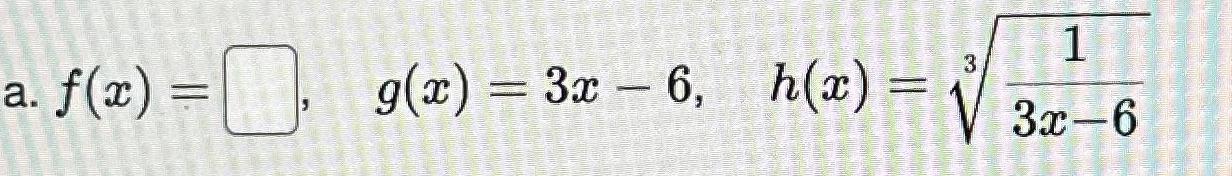
Anatomy and Physiology
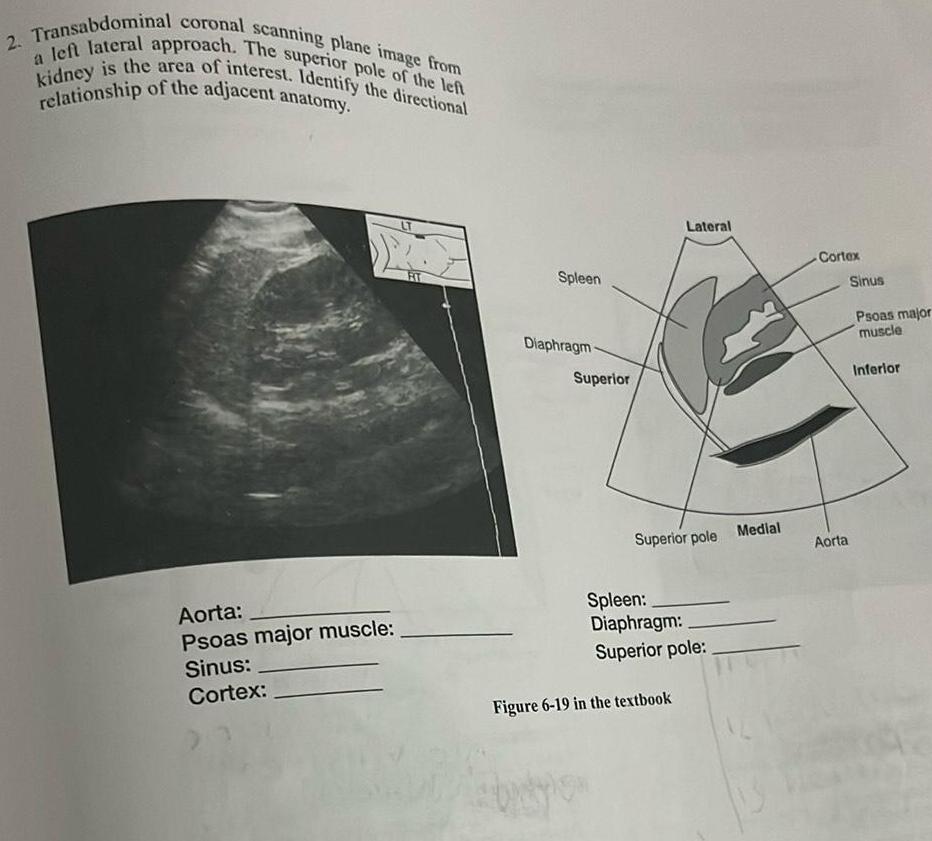
Infex2 Transabdominal coronal scanning plane image from a left lateral approach The superior pole of the left kidney is the area of interest Identify the directional relationship of the adjacent anatomy Aorta Psoas major muscle Sinus Cortex Spleen Diaphragm Superior Lateral Superior pole Spleen Diaphragm Superior pole Figure 6 19 in the textbook Medial Cortex Aorta Sinus Psoas major muscle Inferior
College Calculus
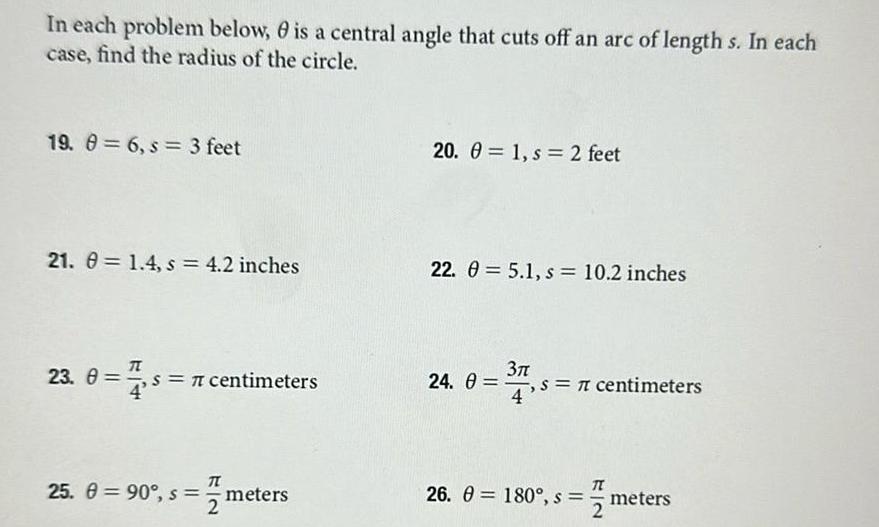
Vector CalculusIn each problem below is a central angle that cuts off an arc of length s In each case find the radius of the circle 19 8 6 s 3 feet 21 1 4 s 4 2 inches 23 0 7 5 7 centimeters 4 25 90 s meters s me 20 1 s 2 feet 22 0 5 1 s 10 2 inches 3 24 0 s 7 centimeters 12 26 180 s meters

College Calculus
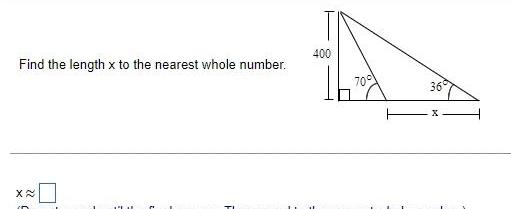
Application of derivatives27 Altitude of a Plane From the ground a 747 Jumbo Jet flying overhead subtends an angle of 0 42 If the length of the jet is 230 feet find its altitude to the nearest thousand feet

College Calculus
Definite IntegralsIn each problem below 0 is a central angle that cuts off an arc of length s In each case find the radius of the circle 19 6 s 3 feet 20 1 s 2 feet


College Calculus
Definite Integrals17 Diameter of the Moon From the earth the moon subtends an angle of approximately 0 5 If the distance to the moon is approximately 240 000 miles find an approximation for the diameter of the moon accurate to the nearest hundred miles See Example 4 and the discussion that precedes it

College Calculus
Limits & Continuity15 Arc Length The pendulum on a grandfather clock swings from side to side once every second If the length of the pendulum is 4 feet and the angle through which it swings is 20 how far does the tip of the pendulum travel in one second

College Calculus
Application of derivatives13 Arc Length A space shuttle 200 miles above the earth is orbiting the earth once every 6 hours How far does the shuttle travel in 1 hour Assume the radius of the earth is 4 000 miles Give both the exact value and a three significant digit approximation for your answer

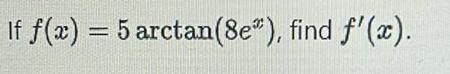
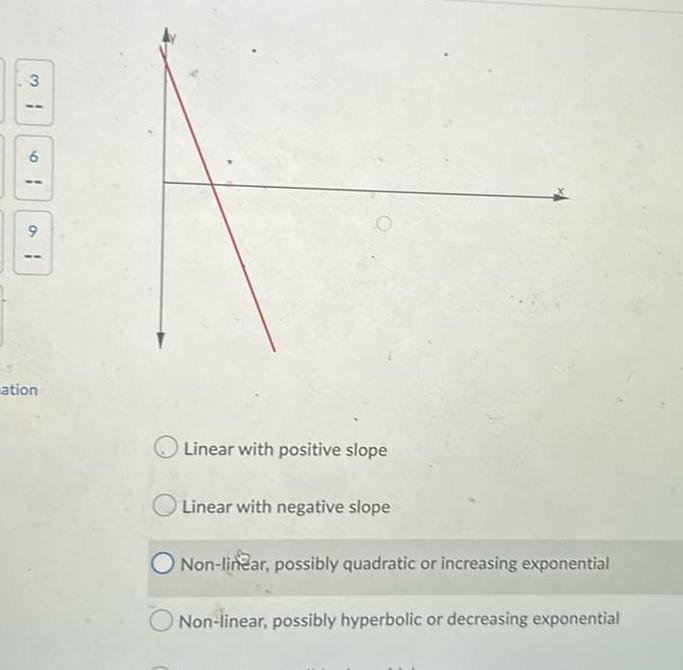
College Math - Others
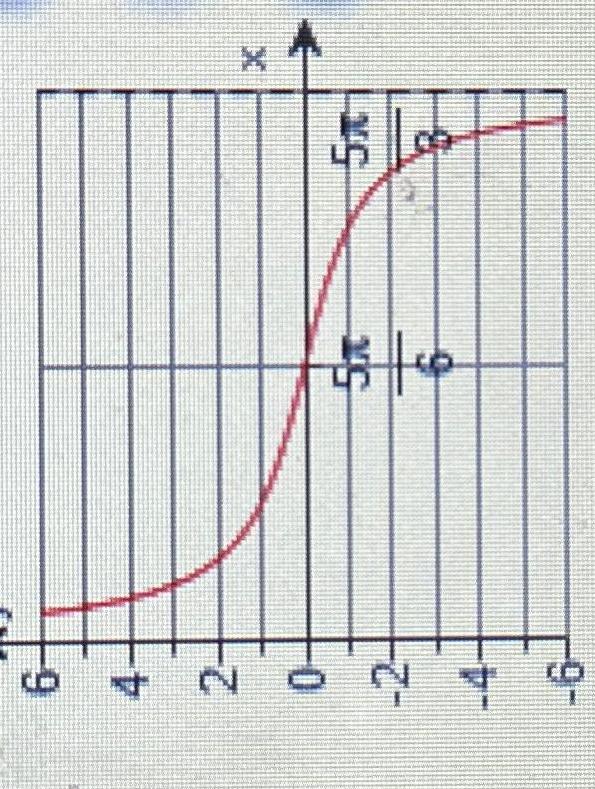
Basic Math3 9 1 9 1 ation Linear with positive slope Linear with negative slope O Non linear possibly quadratic or increasing exponential Non linear possibly hyperbolic or decreasing exponential


College Algebra
Sequences & SeriesSuppose each edge of the cube shown in the figure is 3 00 inches long Find the measure of the angle formed by diagonals DE and DG Round your answer to the nearest tenth of a degree EDG X E A B 111 D F H

Calculus
Indefinite IntegrationFind the slope of the graph of the function at the given point Use the derivative feature of a graphing utility to confirm your results Function Point 0 0 f 0 8 sin 0 0 f 0

College Math - Others
Basic MathThe current gasoline price is 4 24 per gallon and it is projected to increase next year by 5 8 the following year a 10 the third year What is the average inflation rate for the projected gasoline price for the next three years The average inflation rate is Round to two decimal places

College Calculus
Vector CalculusUnless otherwise stated all answers in this problem set that need to be rounded should be rounded to three significant digits For each problem below 0 is a central angle in a circle of radius r In each case find the length of arc s cut off by 0 1 0 2 r 3 inches 3 0 1 5 r 1 5 feet 2 0 3 r 2 inches 4 0 2 4 r 1 8 feet

College Algebra
Quadratic equationsto the due date Given the function f evaluate f 1 0 2 and f 4 f 1 f 0 f 2 f 4 Number Number Number Number f x x 2 4 x 7 if x 2 if x 2

College Calculus
Application of derivatives3 At time t 0 a diver jumps from a platform diving board that is 32 feet above the water Because the initial velocity of the diver is 16 feet per second the position of the diver is s t 16t2 16t 32 where s is measured in feet and t is measured in seconds a 6 points When does the diver hit the water b 6 points What is the diver s velocity at impact c 4 points What is the diver s speed on impact d 4 points What is the acceleration at impact
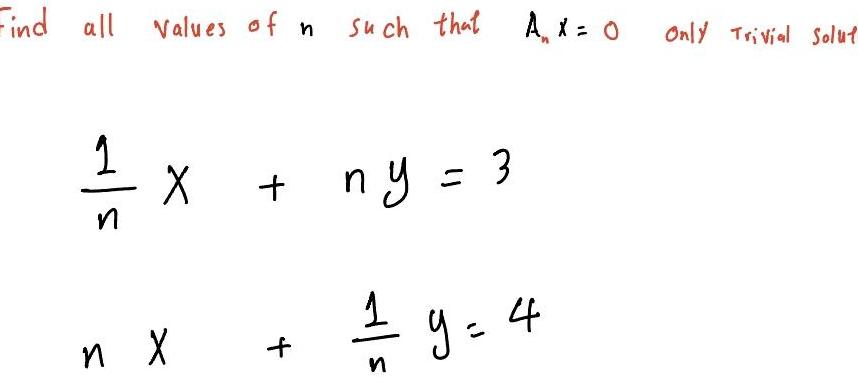
College Algebra
Quadratic equationsFind all values of n such that 3 10 X ny 3 n X A x 0 19 4 y n Only Trivial Solut

High School Calculus
DifferentiationA 2010 estimate of the population of Mexico is 102 million people with a projected growth rate of 0 92 per year At this rate the population P t in millions can be approximated by P9t 102 1 0092 t where t is the time in years since 2010 Find the population in the 2 017 Round your answer to the nearest million Your Answer

College Statistics
ProbabilityIdentify the sampling techniques used and discuss potential sources of bias if any Explain In 1965 researchers used random digit dialing to call 1400 people and ask what obstacles kept them from exercising between phone numbers What potential sources of bias were present if any Select all that apply A No one talks honestly about their exercising habits with people they do not know because it is too personal a subject This may have led to untrue responses to the survey question B Individuals may have refused to participate in the sample This may have made the sample less representative of the population C Individuals may have not been available when the researchers were calling Those individuals that were available may have not been representative of the population D Telephone sampling only includes people who had telephones People who owned telephones may have been older or wealthier on average and may not have been representative of the entire population E There were no potential sources of bias

College Statistics
StatisticsUse the given minimum and maximum data entries and the number of classes to find the class width the lower class limits and the upper class limits minimum 7 maximum 92 6 classes A 22 36 52 67 82 96 OB 7 21 37 51 66 82 OC 21 36 52 66 81 96 OD 7 22 37 52 67 82 Choose the correct upper class limits below OA 22 37 51 66 82 96 OB 21 36 52 67 81 96 OC 21 36 51 66 81 96 OD 22 37 52 67 82 96


College Calculus
DifferentiationMake a diagram of the unit circle with an angle in quadrant I and its complement 180 in quadrant II Label the point on the terminal side of and the unit circle with x y and the point on the terminal side of 180 0 and the unit circle with x y Use this diagram to show that 1 35 sin 180 0 sin 0 37 Show that tangent is an odd function 36 cos 180 0 cos

Anatomy and Physiology
Introduction to PhysiologyThe following appear on a physician s intake form Identify the level of measurement of the data a Time since last visit c Year of birth Interval Ordinal O Ratio O Nominal b Allergies d Pain level scale of 0 to 10 C What is the level of measurement for Year of birth O Nominal O Ordinal d What is the level of measurement for Pain level scale of 0 to 10
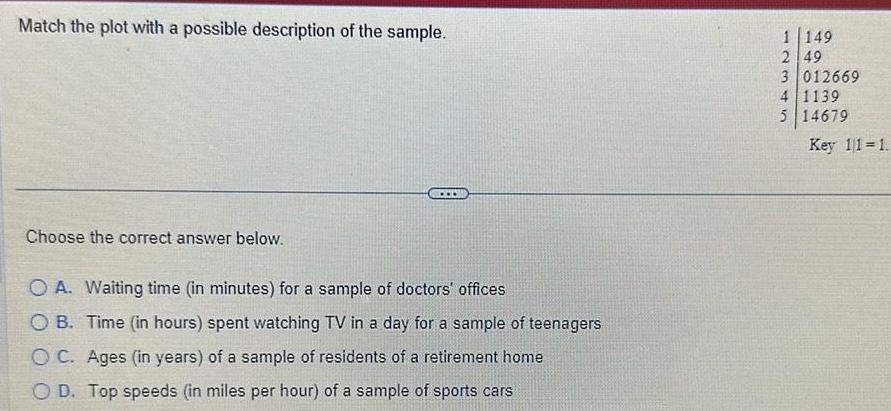
College Statistics
StatisticsMatch the plot with a possible description of the sample Choose the correct answer below O A Waiting time in minutes for a sample of doctors offices OB Time in hours spent watching TV in a day for a sample of teenagers OC Ages in years of a sample of residents of a retirement home OD Top speeds in miles per hour of a sample of sports cars 1 149 2 49 3 012669 4 1139 5 14679 Key 11 1
Biology
Reproduction in OrganismsWhich sense is well developed at birth Ovision O touch O taste hearing
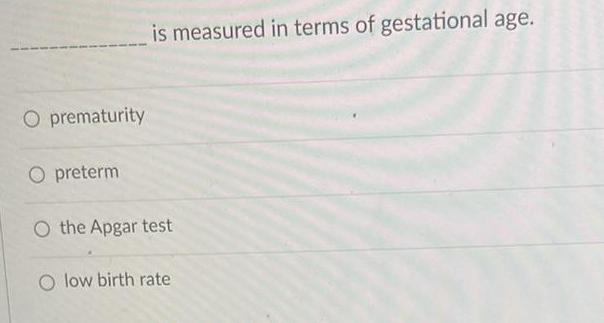
Anatomy and Physiology
Introduction to PhysiologyO prematurity O preterm is measured in terms of gestational age O the Apgar test low birth rate

College Statistics
StatisticsDetermine whether the approximate shape of the distribution in the histogram shown is symmetric uniform skewed left skewed right or none of these Justify your answer Choose the correct answer below OA The shape of the distribution is approximately skewed left because the bars have a tail to the left OB The shape of the distribution is approximately symmetric because the bars have a tail to the left and to the right OC The shape of the distribution is approximately uniform because the bars are approximately the same height OD The shape of the distribution is approximately skewed right because the bars have a tail to the right OE The shape of the distribution is none of these because the bars do not show any of these general trends 18 15 12 9 so 6 34 0 2 4 6 8 10 12 W

College Statistics
StatisticsFind the mean median and mode of the data if possible If any of these measures cannot be found or a measure does not represent the center of the data explain why A sample of seven admission test scores for a professional school are listed below 11 2 11 5 11 5 11 1 10 3 10 1 9 7 CIEE What is the mode of the scores Select the correct choice below and fill in any answer box to complete your choice OA The mode s of the scores is are Use a comma to separate answers as needed OB There is no mode Does Do the mode s represent the center of the data OA The mode s represent s the center OB The mode s can t represent the center because it they is are not a data value OC The mode s does do not represent the center because it one is the smallest data value OD The mode s does do not represent the center because it one is the largest data value
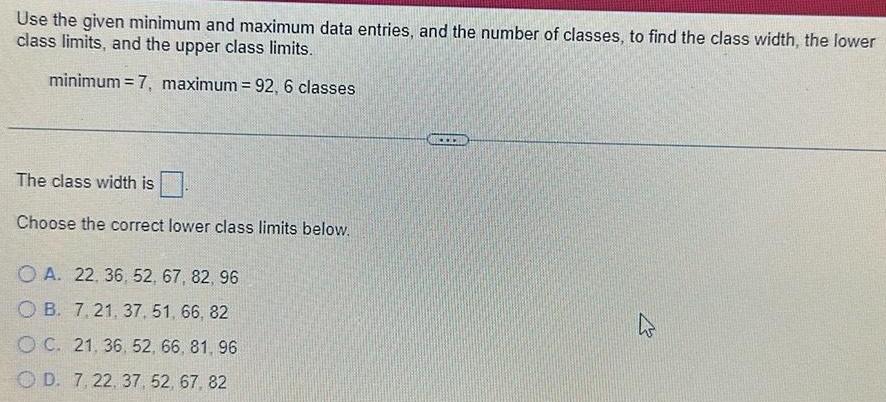
College Statistics
StatisticsUse the given minimum and maximum data entries and the number of classes to find the class width the lower class limits and the upper class limits minimum 7 maximum 92 6 classes The class width is Choose the correct lower class limits below OA 22 36 52 67 82 96 OB 7 21 37 51 66 82 OC 21 36 52 66 81 96 OD 7 22 37 52 67 82 www K

Anatomy and Physiology
Introduction to PhysiologyThe most common drug used by pregnant women is O alcohol O tobacco O marijuana O caffeine
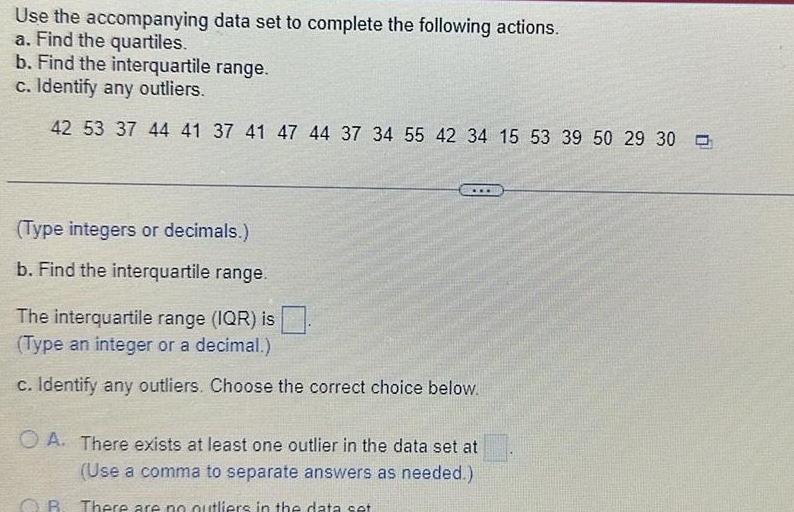
College Statistics
StatisticsUse the accompanying data set to complete the following actions a Find the quartiles b Find the interquartile range c Identify any outliers 42 53 37 44 41 37 41 47 44 37 34 55 42 34 15 53 39 50 29 30 Type integers or decimals b Find the interquartile range The interquartile range IQR is Type an integer or a decimal c Identify any outliers Choose the correct choice below OA There exists at least one outlier in the data set at Use a comma to separate answers as needed B There are no outliers in the data set

College Statistics
StatisticsUse the box and whisker plot to determine if the shape of the distribution represented is symmetric skewed left skewed right or none of these Choose the correct answer below O skewed left O symmetric Onone of these O skewed right SOCCE 0 40 80 120 160 200
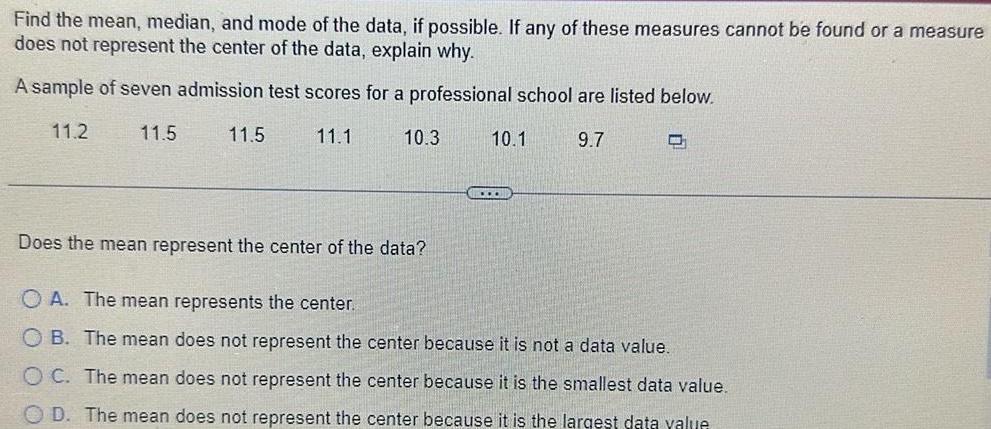
College Statistics
StatisticsFind the mean median and mode of the data if possible If any of these measures cannot be found or a measure does not represent the center of the data explain why A sample of seven admission test scores for a professional school are listed below 11 2 11 5 11 1 9 7 11 5 10 3 10 1 O Does the mean represent the center of the data OA The mean represents the center OB The mean does not represent the center because it is not a data value OC The mean does not represent the center because it is the smallest data value OD The mean does not represent the center because it is the largest data value
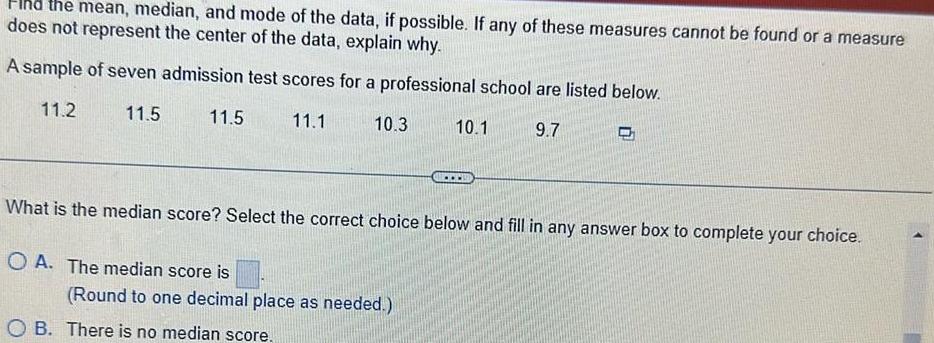
College Statistics
Statisticsthe mean median and mode of the data if possible If any of these measures cannot be found or a measure does not represent the center of the data explain why A sample of seven admission test scores for a professional school are listed below 11 2 11 5 11 1 9 7 11 5 10 3 10 1 What is the median score Select the correct choice below and fill in any answer box to complete your choice OA The median score is Round to one decimal place as needed OB There is no median score


College Statistics
StatisticsThe following appear on a physician s intake form Identify the level of measurement of the data a Time since last visit c Year of birth O b Allergies d Pain level scale of 0 to 10 a What is the level of measurement for Time since last visit Ordinal Interval Ratio Nominal

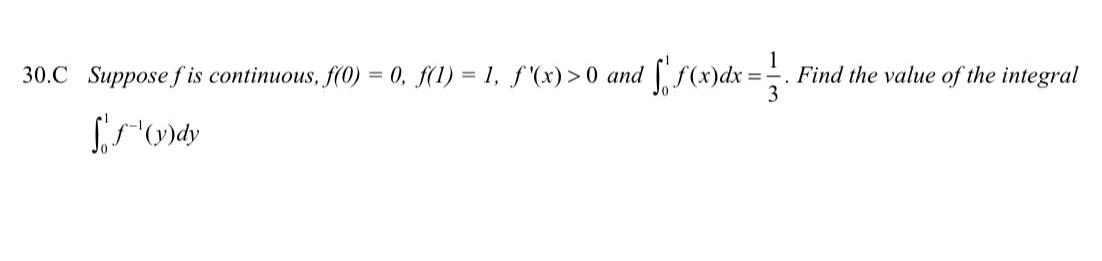
College Calculus
Application of derivatives1 f x dx 1 Find the value of the integral 3 30 C Suppose f is continuous f 0 0 f 1 1 f x 0 and y dy

College Calculus
DifferentiationUse the unit circle to find all values of 0 between 0 and 27 for which 17 sin 0 1 2 19 cos 0 V3 2 21 tan 0 3 18 sin 123 20 cos 80 22 cot 0 3

Biology
Principles of Inheritance & Variation (Genetics)1 61 GTCGACTGGG CACCAGCCGG ATGCGTCCGT CAACCCCGAC TGTTCCGCCA AGAACTCCG CCTTCTGTGG CGGGATGACG TTGAGCACAT CGGCACAGAA AGTGTCGAAC TCGGTTTCG 121 CGGTTCGCCC GGCGGCATCG ATCCGCTCCA GCCGTCCCTC TTTTTCCGAG GAAACCCAT 181 CCACCATGTC TCCGTAGAGT TCGCGCCAGG CCTGCTCGAA TGCCGGCTGC TTGGAAAACC 241 GGGTCTTGGC ATCGAGTATC AGGATCTTGG AGCGGGGTTT GTGCTTTTTC ATGTAATAGE 301 CGATGAGCGA GGCGCGTTCG TAGGGGCCAG GCGGACAACG ATAGGGAGAA GGTGGTGCGG 361 TGATCAGAAC CAGGCCGCCG TCGGGCATCG CGCGGATCTG CCGGGCGAGC AAAGCGGTCT 421 GGGGGCCGGC CTTCCAGGCA TGGGGAACGA ACCGGCTCGC CGCCTCGTCA TAGCCCATTA 481 TCGCCTCCCA GCGAAAGTCG ATGCCGGGGG AGAGGACTAG CCTGTCGTAG GTGACCTCGG 541 CACCATCGTT CAGTATCACA CGCCGTCGCT GCCGATCCAG GCGGGCGACC CGGGCGGTTA 601 CCTTTCCTAT ATCCAGCTCC CGCCGCAACC AGTCATAAGA CCGCGCGAGA GTCCCCATGT 661 CCCCGAGGCC GGCGACTGCT TCGTTCGATC CGGGGCAGGA AAGATAAGTC TCCTGCGGTT 721 CGATCAATGT GATCGTCAGA CCGGGATTCA TCTGCTTCAG ATAGCGGGCG GCCGTGGCAC 781 CGCCATAACC GCCACCGACG ACCACGACCG GCCGCCTGAG CGAAGACCTT GCCGGAAGCG 841 CAGCCACCCA GTCCCAGCCC ATGCCGCAGA CTGCCAGCAG GCGCAGGAAC CGCCGCCGGC 901 GGATCATGGC CTCTTTCCCA GAAAGCCGGC GATCGCTTCA ATGTCCTGGT TCGTGAGTCC 961 GGCAGCGATC CGGTTCATGA CCGTGCCCGA TCTTTTTCCT TCACGGTACT CCCGCAACAG 1021 AGATGCCATC TCCTTCGCAT CGAAGCGGCG TAACGATGCC GGTTCGGGAA TCTGCTCCTC 1081 CTCGTCGGCA TGGCAGCCGA GGCAACCGAG CGCAGCCAAA ACCATGTCCG GTTTTTCGGC 1141 CCGGGCCGGG AAACAGAGAG CGACAGCGAT CGTACCGATC TGGACGCGTC TCACAAACGA 1201 CAAAACGTCA CGATGGGTGT TCGGTAGCTG AGTCACGGGG ATTTGTAGAA GTATAGGACC 1261 GACGGATTTT ATGCAAGCAT GTCGCTTTGA CCAAGCCGGG ATTCCATGGA AGGGATGTCA 1321 TCGGGAGAGT TATTTATGTC GTTGATTTAT AAGAAACTAC CCCTGCGTCA AAATGTCGCA 1381 GATTTTTCTT GACAGTTTGG GGGAGGGTGA TAGATCCTCC ACCGATGGAC CGGTACCGCC 1441 TCTGTTGCGG GGTCCATGAA ATGCCCGTTA GAGGCAGAAC CGATAGGGAA TTAGAGAAGC 1501 GGGCGTCGGC GCCGAATGCC GGCCCCTGTC AACCATCACT TTAGGAGGAA CAAACAATGG 1561 CAGCAACAAC CATTGGTGGT GCAGCTGCGG CGTAAGCGCC GCTGCTGGAC AAGAAGTGGC 1621 TCACGTTCGC ACTGGCGATT TACACCGTGT TCTACCTGTG GGTGCGGTGG TACGAAGGTG 1681 TCTATGGCTG GTCCGCCGGA CTGGACTCGT TCGCGCCGGA GTTCGAGACC TACTGGATGA 1741 ATTTCCTGTA CACCGAGATC GTCCTGGAGA TCGTGACGGC TTCGATCCTG TGGGGCTATC 1801 TCTGGAAGAC CCGCGACCGC AACCTGGCCG CGCTGACCCC GCGTGAAGAG CTGCGCCGCA 1861 ACTTCACCCA CCTGGTGTGG CTGGTGGCCT ACGCCTGGGC CATCTACTGG GGCGCATCCT 1921 ACTTCACCGA GCAGGACGGC ACCTGGCATC AGACGATCGT GCGCGACACC GACTTCACGC 1981 CGTCGCACAT CATCGAGTTC TATCTGAGCT ACCCGATCTA CATCATCACC GGTTTTGCGG 2041 CGTTCATCTA CGCCAAGACG CGTCTGCCGT TCTTCGCGAA GGGCATCTCG CTGCCGTACC 2101 TGGTGCTGGT GGTGGGTCCG TTCATGATTC TGCCGAACGT GGGTCTGAAC GAATGGGGCC

Biology
Principles of Inheritance & Variation (Genetics)1 GTCGACTGGG CACCAGCCGG ATGCGTCCGT CAACCCCGAC TGTTCCGCCA AGAACTCCGO 61 CCTTCTGTGG CGGGATGACG TTGAGCACAT CGGCACAGAA AGTGTCGAAC TCGGTTTCGA 121 CGGTTCGCCC GGCGGCATCG ATCCGCTCCA GCCGTCCCTC TTTTTCCGAG GAAACCCATT 181 CCACCATGTC TCCGTAGAGT TCGCGCCAGG CCTGCTCGAA TGCCGGCTGC TTGGAAAACC 241 GGGTCTTGGC ATCGAGTATC AGGATCTTGG AGCGGGGTTT GTGCTTTTTC ATGTAATAGG 301 CGATGAGCGA GGCGCGTTCG TAGGGGCCAG GCGGACAACG ATAGGGAGAA GGTGGTGCGG 361 TGATCAGAAC CAGGCCGCCG TCGGGCATCG CGCGGATCTG CCGGGCGAGC AAAGCGGTCT 421 GGGGGCCGGC CTTCCAGGCA TGGGGAACGA ACCGGCTCGC CGCCTCGTCA TAGCCCATTA 481 TCGCCTCCCA GCGAAAGTCG ATGCCGGGGG AGAGGACTAG CCTGTCGTAG GTGACCTCGG 541 CACCATCGTT CAGTATCACA CGCCGTCGCT GCCGATCCAG GCGGGCGACC CGGGCGGTTA 601 CCTTTCCTAT ATCCAGCTCC CGCCGCAACC AGTCATAAGA CCGCGCGAGA GTCCCCATGT 661 CCCCGAGGCC GGCGACTGCT TCGTTCGATC CGGGGCAGGA AAGATAAGTC TCCTGCGGTT 721 CGATCAATGT GATCGTCAGA CCGGGATTCA TCTGCTTCAG ATAGCGGGCG GCCGTGGCAC 781 CGCCATAACC GCCACCGACG ACCACGACCG GCCGCCTGAG CGAAGACCTT GCCGGAAGCG 841 CAGCCACCCA GTCCCAGCCC ATGCCGCAGA CTGCCAGCAG GCGCAGGAAC CGCCGCCGGC 901 GGATCATGGC CTCTTTCCCA GAAAGCCGGC GATCGCTTCA ATGTCCTGGT TCGTGAGTCC 961 GGCAGCGATC CGGTTCATGA CCGTGCCCGA TCTTTTTCCT TCACGGTACT CCCGCAACAG 1021 AGATGCCATC TCCTTCGCAT CGAAGCGGCG TAACGATGCC GGTTCGGGAA TCTGCTCCTC 1081 CTCGTCGGCA TGGCAGCCGA GGCAACCGAG CGCAGCCAAA ACCATGTCCG GTTTTTCGGC 1141 CCGGGCCGGG AAACAGAGAG CGACAGCGAT CGTACCGATC TGGACGCGTC TCACAAACGA 1201 CAAAACGTCA CGATGGGTGT TCGGTAGCTG AGTCACGGGG ATTTGTAGAA GTATAGGACC 1261 GACGGATTTT ATGCAAGCAT GTCGCTTTGA CCAAGCCGGG ATTCCATGGA AGGGATGTCA 1321 TCGGGAGAGT TATTTATGTC GTTGATTTAT AAGAAACTAC CCCTGCGTCA AAATGTCGCA 1381 GATTTTTCTT GACAGTTTGG GGGAGGGTGA TAGATCCTCC ACCGATGGAC CGGTACCGCC 1441 TCTGTTGCGG GGTCCATGAA ATGCCCGTTA GAGGCAGAAC CGATAGGGAA TTAGAGAAGO 1501 GGGCGTCGGC GCCGAATGCC GGCCCCTGTC AACCATCACT TTAGGAGGAA CAAACAATGG 1561 CAGCAACAAC CATTGGTGGT GCAGCTGCGG CGTAAGCGCC GCTGCTGGAC AAGAAGTGGC 1621 TCACGTTCGC ACTGGCGATT TACACCGTGT TCTACCTGTG GGTGCGGTGG TACGAAGGTG 1681 TCTATGGCTG GTCCGCCGGA CTGGACTCGT TCGCGCCGGA GTTCGAGACC TACTGGATGA 1741 ATTTCCTGTA CACCGAGATC GTCCTGGAGA TCGTGACGGC TTCGATCCTG TGGGGCTATC 1801 TCTGGAAGAC CCGCGACCGC AACCTGGCCG CGCTGACCCC GCGTGAAGAG CTGCGCCGCA 1861 ACTTCACCCA CCTGGTGTGG CTGGTGGCCT ACGCCTGGGC CATCTACTGG GGCGCATCCT 1921 ACTTCACCGA GCAGGACGGC ACCTGGCATC AGACGATCGT GCGCGACACC GACTTCACGC 1981 CGTCGCACAT CATCGAGTTC TATCTGAGCT ACCCGATCTA CATCATCACC GGTTTTGCGG 2041 CGTTCATCTA CGCCAAGACG CGTCTGCCGT TCTTCGCGAA GGGCATCTCG CTGCCGTACC 2101 TGGTGCTGGT GGTGGGTCCG TTCATGATTC TGCCGAACGT GGGTCTGAAC GAATGGGGCC 2161 ACACCTTCTG GTTCATGGAA GAGCTGTTCG TGGCGCCGCT GCACTACGGC TTCGTGATCT

College Calculus
Limits & ContinuityIf we start at the point 1 0 and travel once around the unit circle we travel a dis tance of 27 units and arrive back where we started at the point 1 0 If we continue around the unit circle a second time we will repeat all the values of x and y that occurred during our first trip around Use this discussion to evaluate the following expressions 27 sin 27 29 sin 27 7 2 2n 2 28 cos 2 2 33 30 cos 27