Calculus Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Calculus
Application of derivativesWrite the first five terms of the sequence defined recursively 1 2ak a a2 a3 a4 a5 a 80 ak 1

Calculus
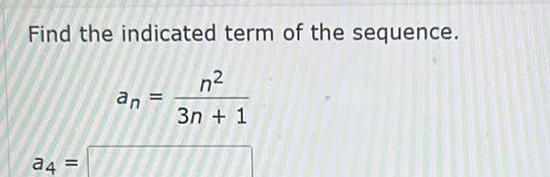
Application of derivativesWrite an expression for the apparent nth term an of the sequence Assume that n begins with 1 1 6 11 16 21 an

Calculus
Application of derivatives2 The graph of which of the following quadratics does NOT have a vertex on the x axis 1x 1x A y 3 x 2 x 2 Ich B 5 x 3 yx x 16 Dy x 4 x 4 8 4 6 Za 1 2 6 X 4 y 4 86 48 16
Calculus
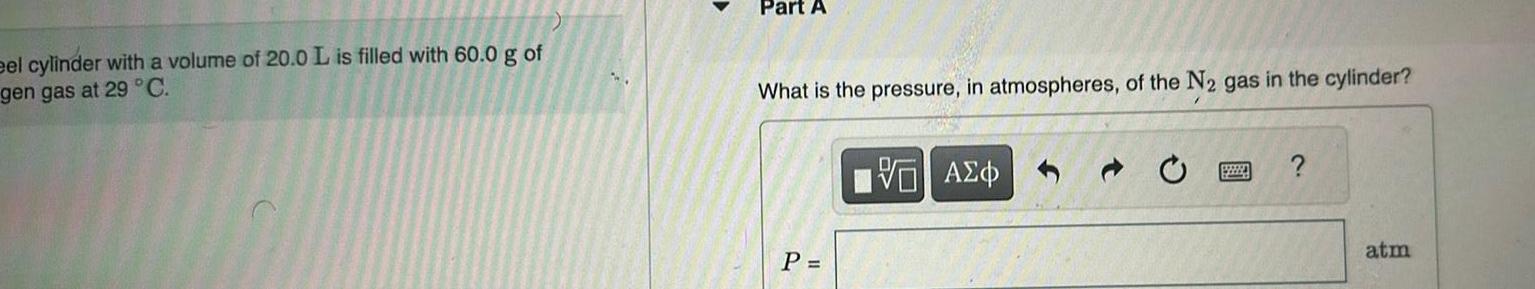
Vector Calculuseel cylinder with a volume of 20 0 L is filled with 60 0 g of gen gas at 29 C Part A What is the pressure in atmospheres of the N gas in the cylinder P VAEO 199224 atm
Calculus
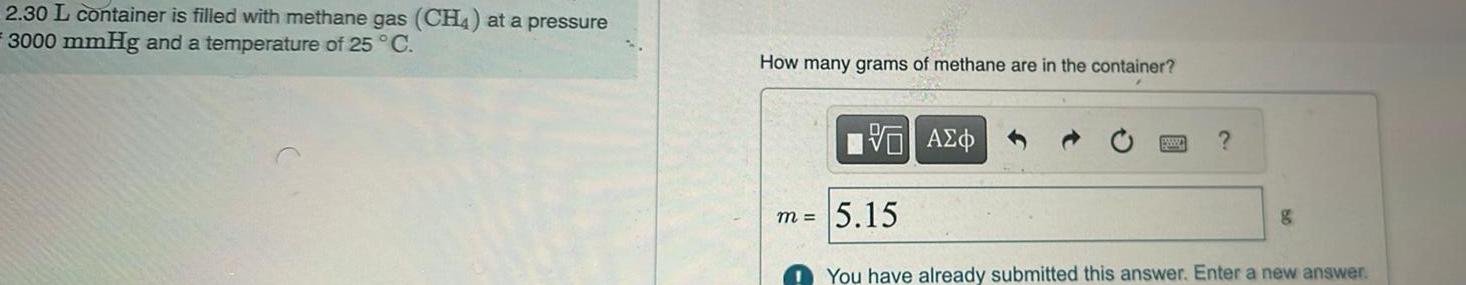
Vector Calculus2 30 L container is filled with methane gas CH4 at a pressure 3000 mmHg and a temperature of 25 C How many grams of methane are in the container m VO A O 5 15 g You have already submitted this answer Enter a new answer
Calculus
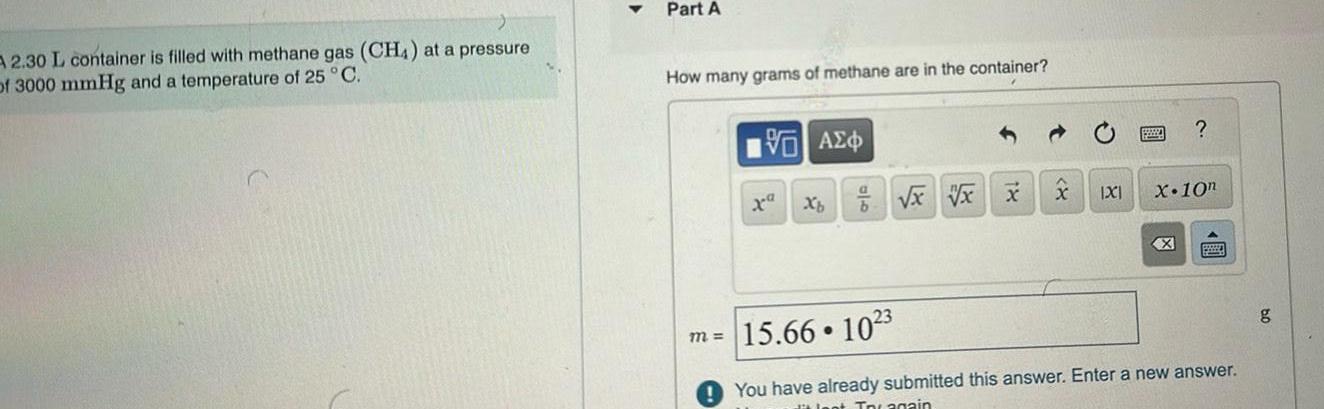
DifferentiationA 2 30 L container is filled with methane gas CH4 at a pressure of 3000 mmHg and a temperature of 25 C Part A How many grams of methane are in the container VAE m Xb b x xx x IXI X 10n X pay 15 66 1023 You have already submitted this answer Enter a new answer Jest Incagain g

Calculus
Definite IntegralsFind the most general antiderivative of the function Check your answer by differentiation Use C for the constant of the antiderivative f x x 3 5x 3 F x 5 3 6x 9x cx

Calculus
Indefinite IntegrationThe portion of the graph Distance is measured in W 3360 651 y tan x between x 0 and x 1 is rotated around the y axis to form a container The container is filled with water Use n 6 subintervals and Simpson s rule to approximate the work required to pump all of the water out over the side of the container Give your a meters the density of water is 1000 kg m and use 9 8 m s for g
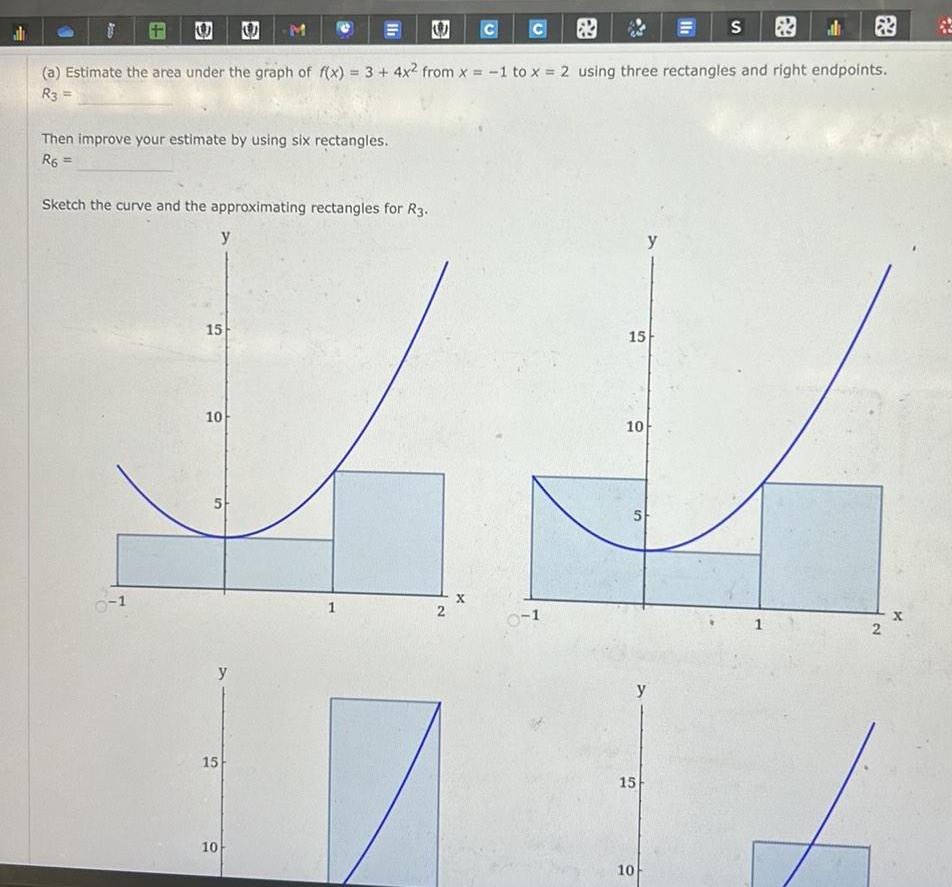
Calculus
Vector CalculusThen improve your estimate by using six rectangles R6 1 Sketch the curve and the approximating rectangles for R3 a Estimate the area under the graph of f x 3 4x from x 1 to x 2 using three rectangles and right endpoints R3 15 y 10 M 5 15 II 10 e 2 X 0 1 15 10 5 15 10 y y Ih S 1 2 x

Calculus
Application of derivativesIs your estimate an underestimate or an overestimate underestimate overestimate b Repeat part a using left endpoints L4 4 Sketch the graph and the rectangles y y 4 M 3 A 8 R 4 f x 4 cos x 3 8 f x 4 cos x 8 E X 4 S f x 4 cos x 3x 8 f x 4 cos x X
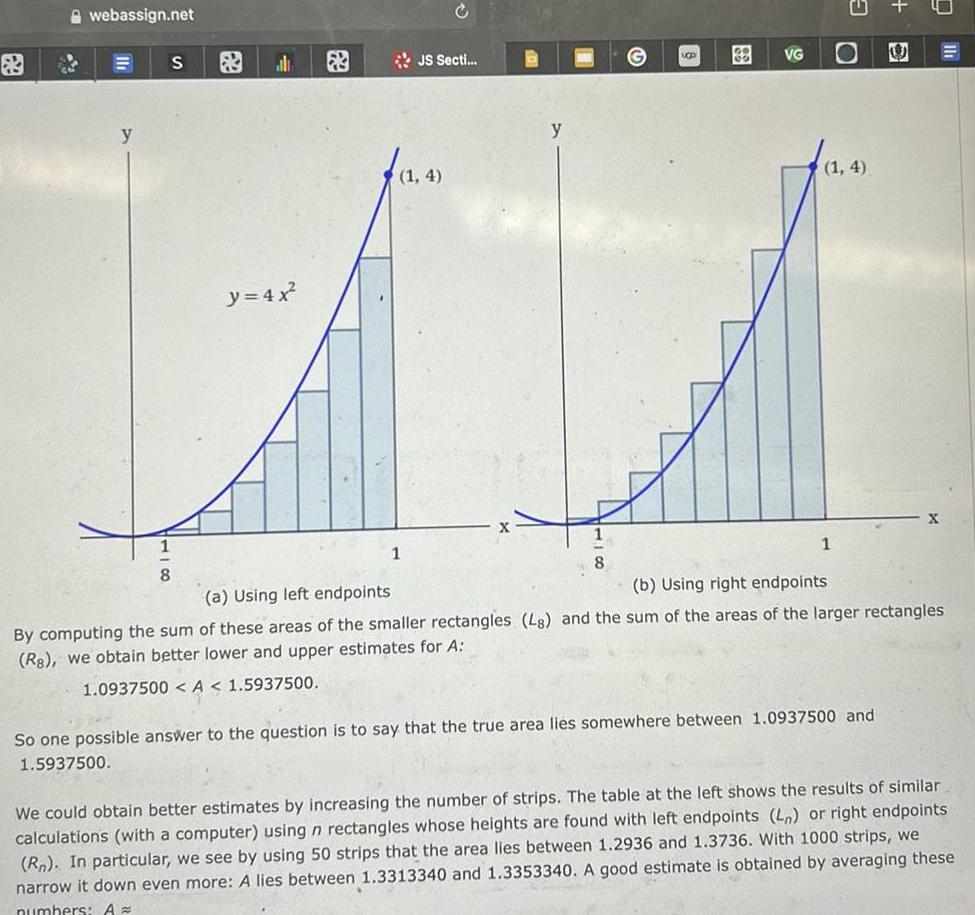
Calculus
Indefinite Integrationwebassign net y S 1 8 al y 4x JS Secti 1 4 1 y 8 UGD VG 1 4 1 U So one possible answer to the question is to say that the true area lies somewhere between 1 0937500 and 1 5937500 X a Using left endpoints b Using right endpoints By computing the sum of these areas of the smaller rectangles Ls and the sum of the areas of the larger rectangles Rs we obtain better lower and upper estimates for A 1 0937500 A 1 5937500 We could obtain better estimates by increasing the number of strips The table at the left shows the results of similar calculations with a computer using n rectangles whose heights are found with left endpoints L or right endpoints Rn In particular we see by using 50 strips that the area lies between 1 2936 and 1 3736 With 1000 strips we narrow it down even more A lies between 1 3313340 and 1 3353340 A good estimate is obtained by averaging these numbers A
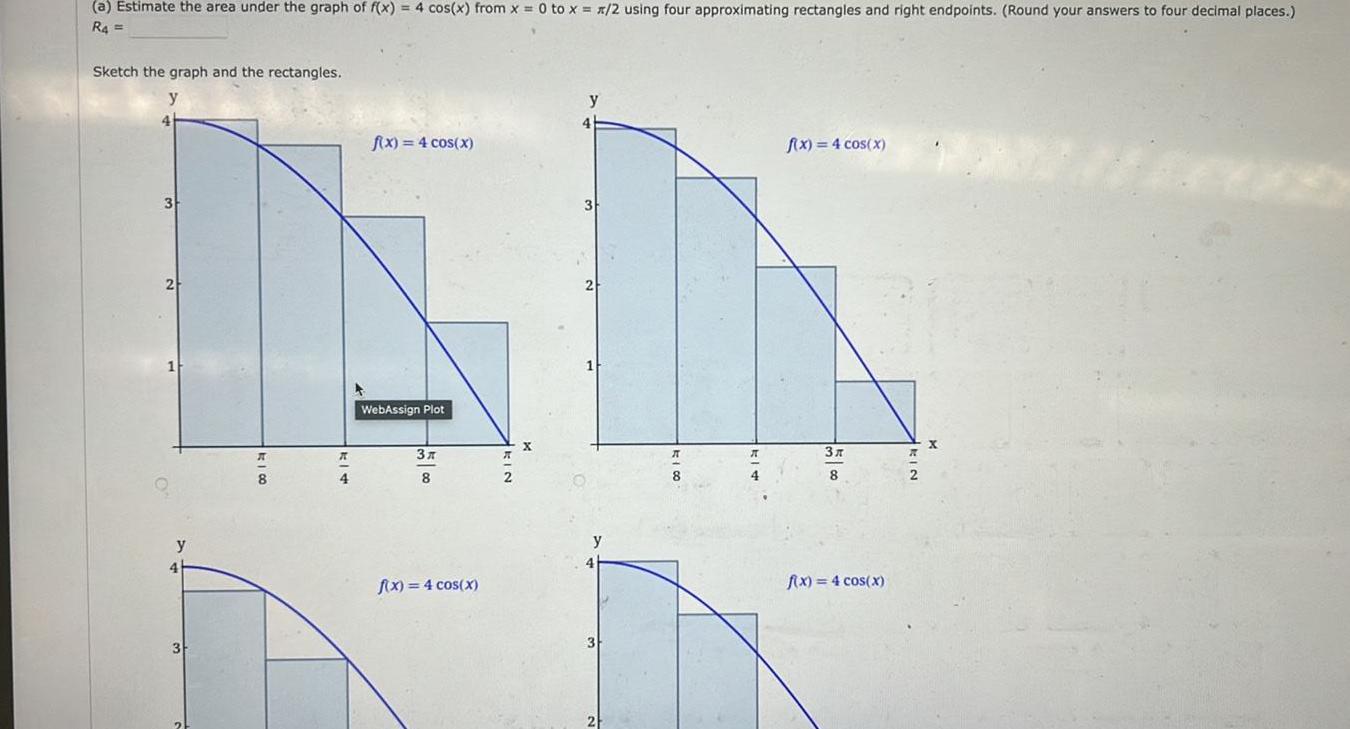
Calculus
Application of derivativesa Estimate the area under the graph of f x 4 cos x from x 0 to x x 2 using four approximating rectangles and right endpoints Round your answers to four decimal places R4 Sketch the graph and the rectangles y 4 R 8 R 4 f x 4 cos x WebAssign Plot 3x 8 f x 4 cos x X A A 4 f x 4 cos x 3 8 f x 4 cos x

Calculus
Indefinite Integration11 S R4 1 4 S y 4x 2 HIC al 2 S3 3 4 S4 1 Each rectangle has width and the heights are areas of these approximating rectangles we get JS Secti 201 2 4 4 4 4 2 1 4 1 BB 38 3 VG 1 a b We can approximate each strip by a rectangle whose base is the same as the strip and whose height is the same as the right edge of the strip as in Figure b above In other words the heights of these rectangles are the values of the function f x 4x at the right endpoints of the subintervals 0 and 4 1 4 4 2 and 4 1 If we let R4 be the sum of the We see that the area A is less than R4 so A Instead of using the rectangles above we could use the smaller rectangles whose heights are the values of fat the left endpoints of the sub intervals The leftmost rectangle has collapsed because its height is 0 The sum of the areas of these approximating rectangles is U La 4 0 4 1 4 4 We see that the area is larger than L4 so we have lower and upper estimates for A A We can repeat this procedure with a larger number of strips The figure shows what happens when we divide the night X
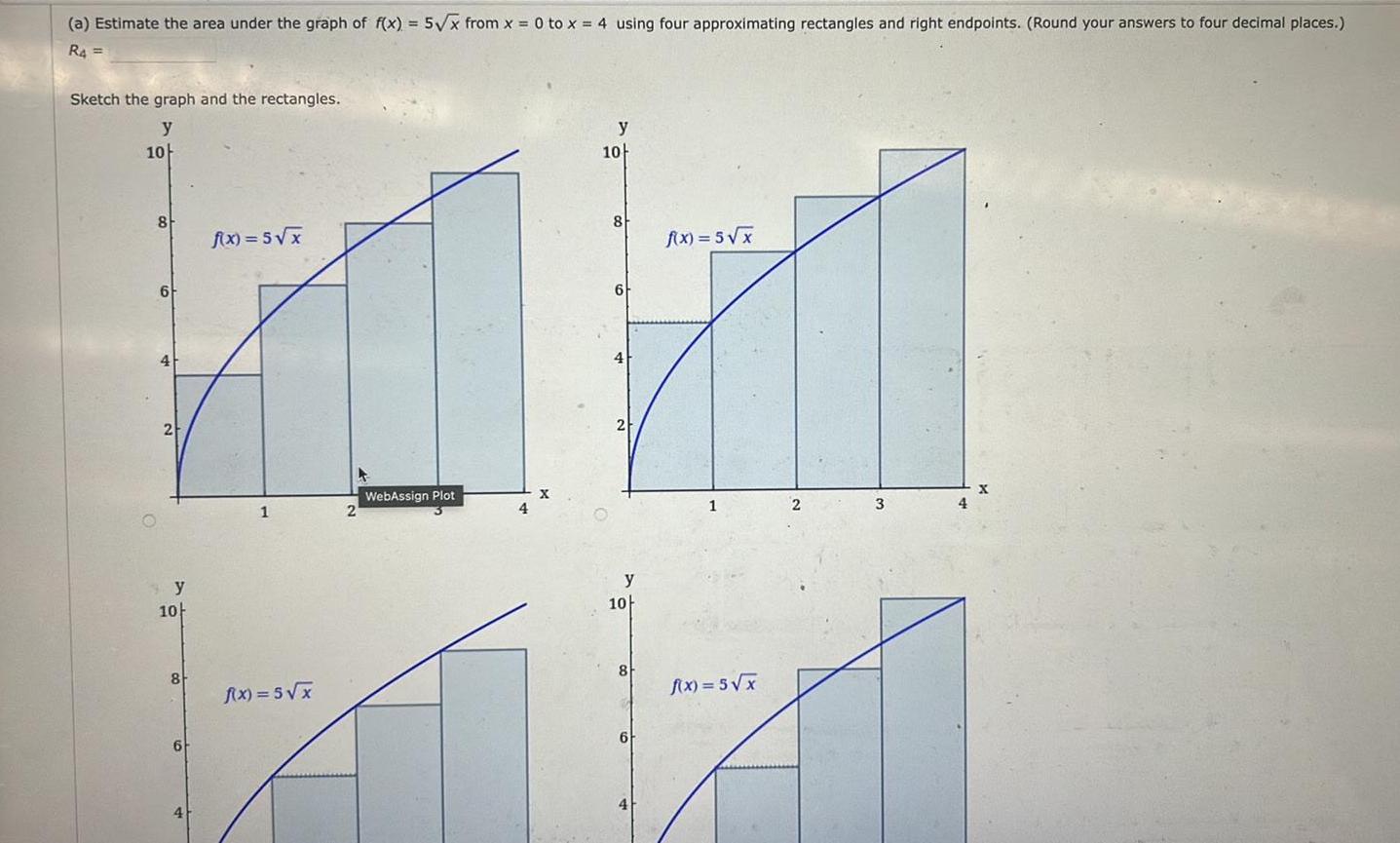
Calculus
Indefinite Integrationa Estimate the area under the graph of f x 5 x from x 0 to x 4 using four approximating rectangles and right endpoints Round your answers to four decimal places R4 Sketch the graph and the rectangles y 10 8 6 2 y 10 8 6 4 f x 5 x 1 f x 5 x WebAssign Plot 4 y 10 8 2 f x 5 x 1 2 3 y 10 8 f x 5 x At 6 X


Calculus
Limits & ContinuityA pharmacist has 80 milliliters of a 25 saline solution Which expression can be used to find the percentage concentration of the final solution if she adds x milliliters of a 10 saline solution y 0 25 80 0 1x 100
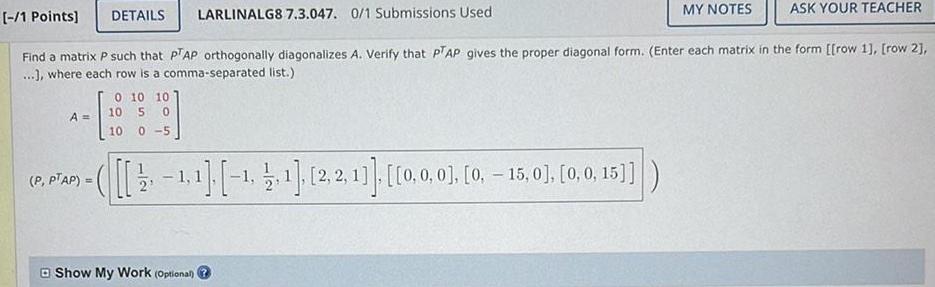
Calculus
Limits & Continuity1 Points DETAILS LARLINALG8 7 3 047 0 1 Submissions Used ASK YOUR TEACHER Find a matrix P such that PTAP orthogonally diagonalizes A Verify that PTAP gives the proper diagonal form Enter each matrix in the form row 1 row 2 where each row is a comma separated list A P PTAP 0 10 10 10 50 10 0 5 2 1 1 1 1 1 2 2 1 0 0 0 0 15 0 0 0 15 MY NOTES Show My Work Optional
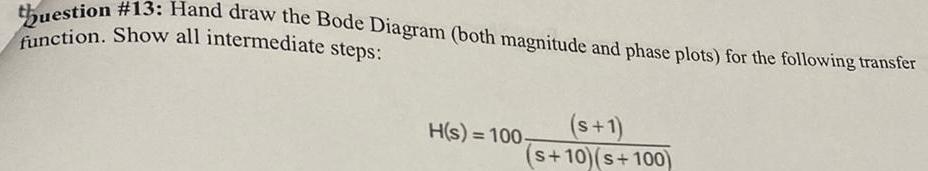
Calculus
Application of derivativesquestion 13 Hand draw the Bode Diagram both magnitude and phase plots for the following transfer function Show all intermediate steps s 1 s 10 s 100 H s 100
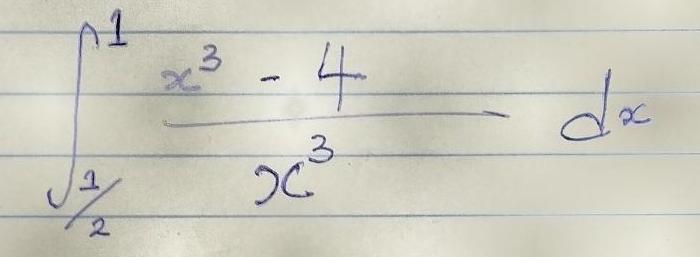

Calculus
Definite Integrals3 6 pts Use the properties of logarithms to fully expand and simplify the expression log 8 x 7 Z 4 6 pts Use the properties of logarithms to write the expression as a single logarithm 3 log x 2 log y 3 log y 5 6 pts Use the properties of logarithms to fully expand and simplify the expression 5 x 1 25 z logs

Calculus
Vector Calculus5 6 pts Use the properties of logarithms to fully expand and simplify the expression 5 x 1 25 3 Z log5

Calculus
DifferentiationThe Narso ring is a circular test track for cars It has a circumference of 12 5km Cars travel around the track at a constant speed of 100km h A car starts at the easternmost poijt of the ring and drives for 22 5 minutes at this speed what is the distance in km does the car travel
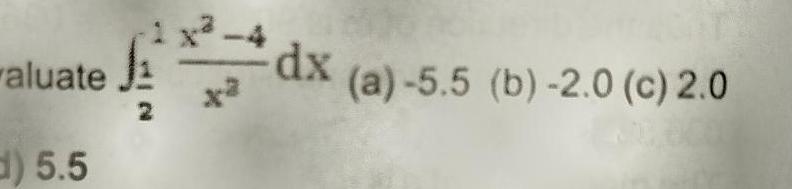


Calculus
Vector Calculusa What frequency is received by a person watching an oncoming ambulance moving at 29 2 m s and emitting a steady 713 Hz sound from its iren The speed of sound on this day is 345 m s Hz b What frequency does she receive after the ambulance has passed Hz

Calculus
Vector CalculusFind the a amplitude b penod c phase shift if any d vertical translation if any and e range of the following function Then graph the function over at least one period y cos a The amplitude is Simplify your answer b The period is Simplify your answer Type an exact answer using x as needed Use integers or fractions for any numbers in the expression SCODE c Select the correct choice below and if necessary fill in the answer box to complete your choice OA The phase shift is Type an exact answer using as needed Use integers or fractions for any numbers in the expression OB There is no phase shift d Select the correct choice below and it necessary fill in the answer box to complete your choice OA The vertical translation is unit s down Type an integer or a simplified fraction OB The vertical translation is unit s up Type an integer or a simplified fraction OC There is no vertical translation 48 G Find the a amplitude b period c phase shift if any d vertical translation if any and e range of the following function Then graph the function over at least one period y cos 0 e The range is Type your answer in interval notation Select all the transformations that are needed to graph the given function using y cos x A Reflect the graph across the x axis 1 c Stretch the graph vertically by a factor of E Translate the graph units to the left G Translate the graph units down OL Translate the graph units up Use the graphing tool to graph the function Click to enlarge graph GOLES B Stretch the graph horizontally by a factor of 2 D Translate the graph units to the right X F Reflect the graph across the y axis H Shrink the graph vertically by a factor of J Shrink the graph horizontally by a factor of 2

Calculus
Application of derivativesName Instructions Use algebraic techniques to solve problems unless otherwise instructed Show the calculations leading to your answers except basic arithmetic Simplify and circle your final answers 1 6 pts What is the domain of the function f x log X 3 7 x Show the work sign analysis 2 8 pts a What is the average rate of change of f x log x between 1 9 and 27 Write the answer as a fraction no decimals or mixed numbers b What is the equation of the secant line through 1 9 f 1 9 and 27 f 27 Use fractions in the
Calculus
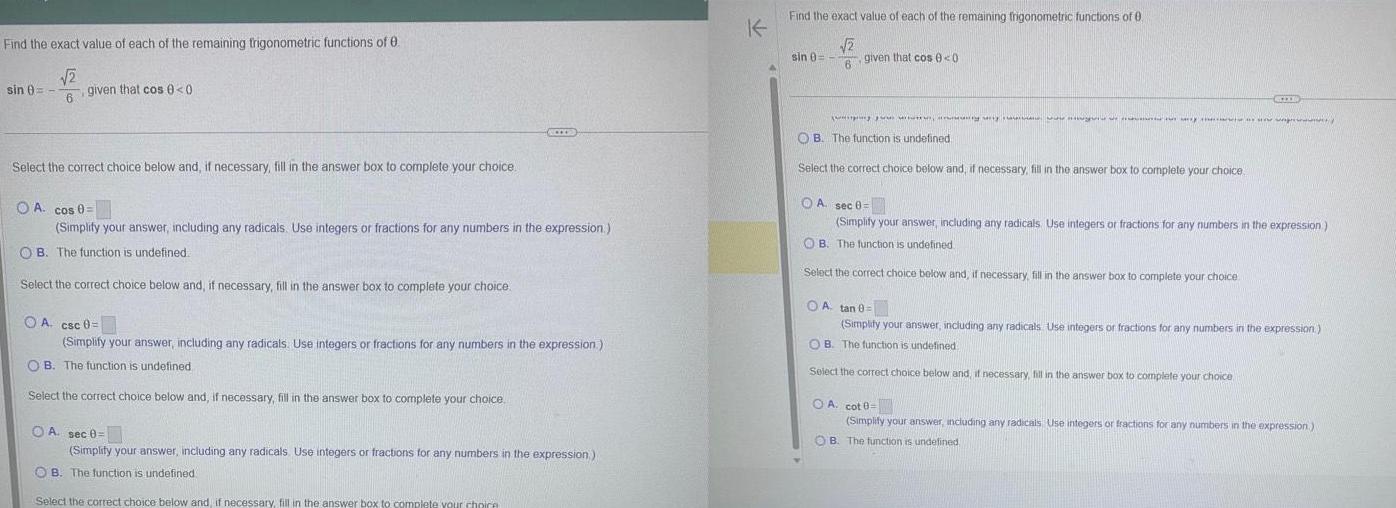
Application of derivativesFind the exact value of each of the remaining trigonometric functions of 0 2 6 sin given that cos 0 0 Select the correct choice below and if necessary fill in the answer box to complete your choice ACCESS OA cos 0 Simplify your answer including any radicals Use integers or fractions for any numbers in the expression OB The function is undefined Select the correct choice below and if necessary fill in the answer box to complete your choice OA csc 0 Simplify your answer including any radicals Use integers or fractions for any numbers in the expression OB The function is undefined Select the correct choice below and if necessary fill in the answer box to complete your choice OA sec 8 Simplify your answer including any radicals Use integers or fractions for any numbers in the expression OB The function is undefined Select the correct choice below and if necessary fill in the answer box to complete your choice Find the exact value of each of the remaining trigonometric functions of 0 2 6 sin 0 given that cos 0 0 i wy OB The function is undefined Select the correct choice below and if necessary fill in the answer box to complete your choice KIXE OA sec 8 Simplify your answer including any radicals Use integers or fractions for any numbers in the expression OB The function is undefined Select the correct choice below and if necessary fill in the answer box to complete your choice OA tan 0 Simplify your answer including any radicals Use integers or fractions for any numbers in the expression B The function is undefined Select the correct choice below and if necessary fill in the answer box to complete your choice OA cot 8 Simplify your answer including any radicals Use integers or fractions for any numbers in the expression OB The function is undefined

Calculus
Application of derivativesTunction graphed to the right is of the form y a tan bx or y a cot bx for some a 0 b 0 etermine the equation of the function Half and quarter points are identified by dots an equation of the function shown is y Ay IN

Calculus
Differential equationsSome biologists model the number of species S in a fixed area A such as an island by the species area relationship log S log c k log A where c and k are positive constants that depend on the type of species and habitat a Solve the equation for S S h Hein

Calculus
DifferentiationSolve the equation and express the solution in exact form In 4x 3 In 9 In x 3 O A OB O C 0 15 15 19 4 3 2 OD
Calculus
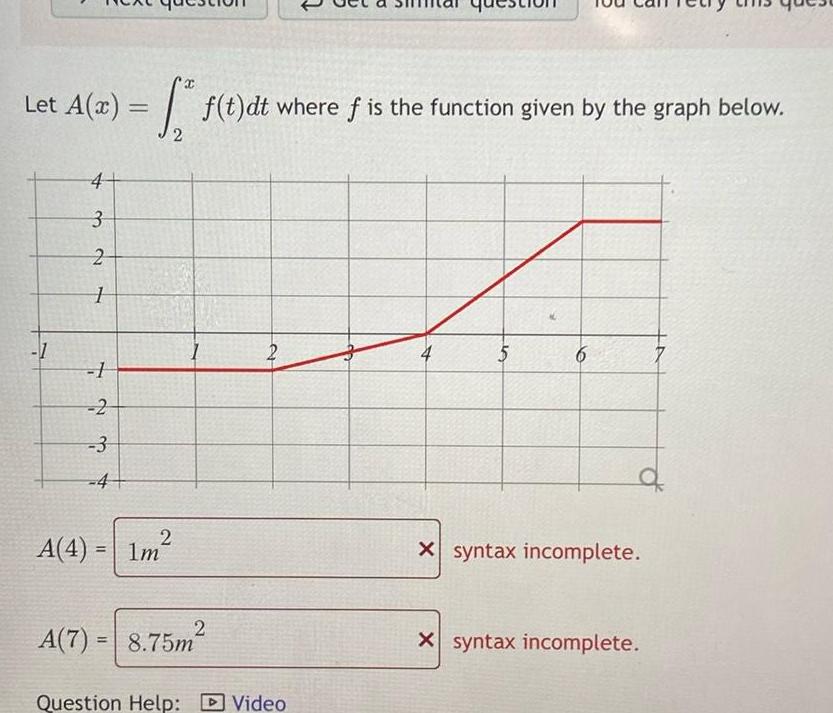
Definite IntegralsLet A x f t dt where f is the function given by the graph below 2 1 4 3 2 1 1 2 34 4 A 4 1m 2 2 A 7 8 75m 2 Question Help Video 5 X syntax incomplete X syntax incomplete

Calculus
Application of derivativesSolve the equation Round to the nearest thousandth 3e5x 3 12 O A 0 323 OB 0 766 OC 0 877 OD 3 000

Calculus
DifferentiationThe logarithm of a number raised to a power is the same as the power times the logarithm of the number So log5 2512 12

Calculus
Limits & Continuityind the domain of the function x log 10 x 13x 42 OA 0 6 U 7 00 OB 7 00 OC 6 7 OD 6
Calculus
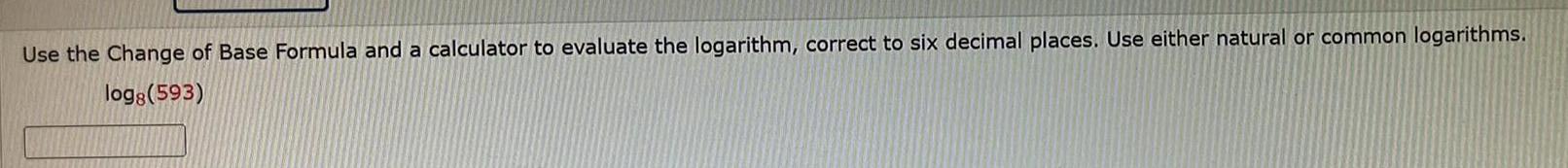
DifferentiationUse the Change of Base Formula and a calculator to evaluate the logarithm correct to six decimal places Use either natural or common logarithms logg 593

Calculus
Vector CalculusSuppose that in a memory experiment the rate of memorizing is given by M t 0 006t 0 3t where M t is the memory rate in words per minute How many words are memorized in the first 11 minutes words Round your answer to the nearest whole word

Calculus
Application of derivativesUse the Laws of Logarithms 2 In 2 4 In x In x to combine the expression In In x 5
Calculus
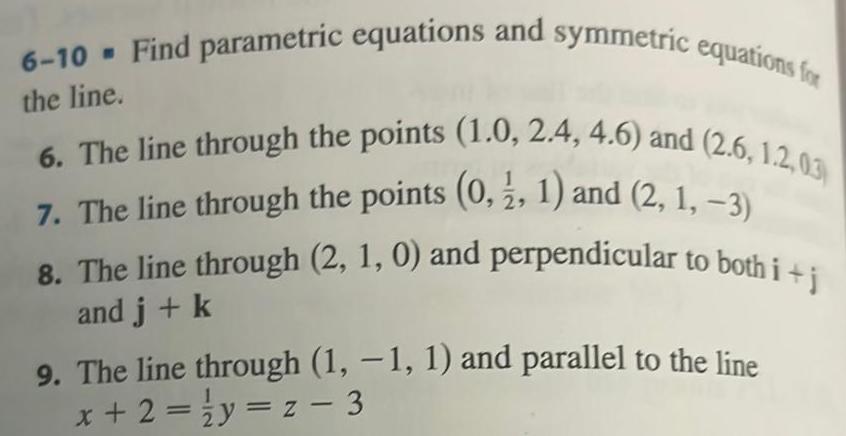
Vector Calculus6 10 Find parametric equations and symmetric equations for the line 6 The line through the points 1 0 2 4 4 6 and 2 6 1 2 03 7 The line through the points 0 2 1 and 2 1 3 8 The line through 2 1 0 and perpendicular to both i j and j k 9 The line through 1 1 1 and parallel to the line x 2 y z 3
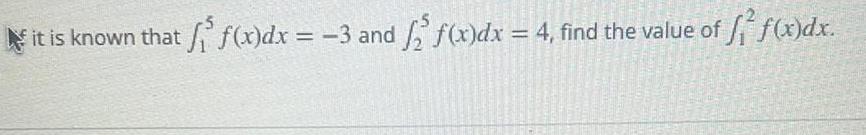
Calculus
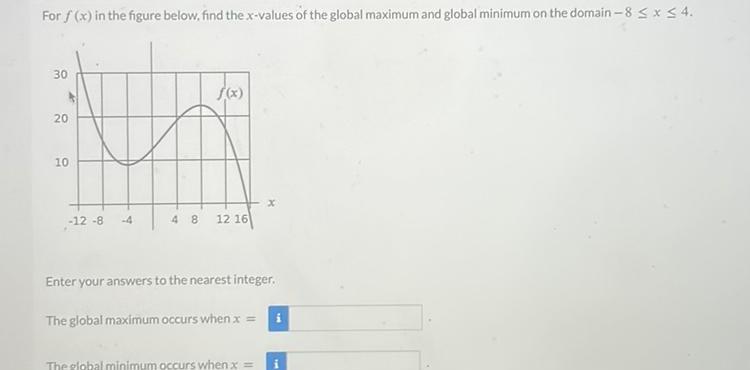
Limits & ContinuityFor f x in the figure below find the x values of the global maximum and global minimum on the domain 8 x 4 30 20 10 12 8 J f x 4 8 12 16 Enter your answers to the nearest integer The global maximum occurs when x The global minimum occurs when x i


Calculus
Definite IntegralsThe logarithm of a product of two numbers is the same as the sum of the logarithms of these numbers So log4 16 64 log4 16
Calculus
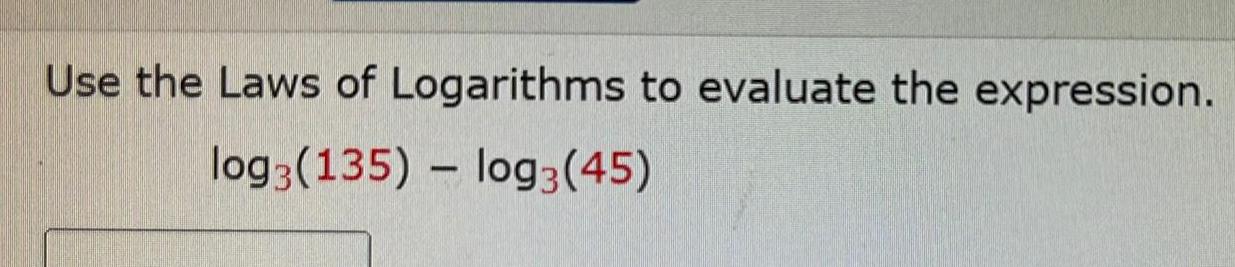
Application of derivativesUse the Laws of Logarithms to evaluate the expression log3 135 log3 45
Calculus
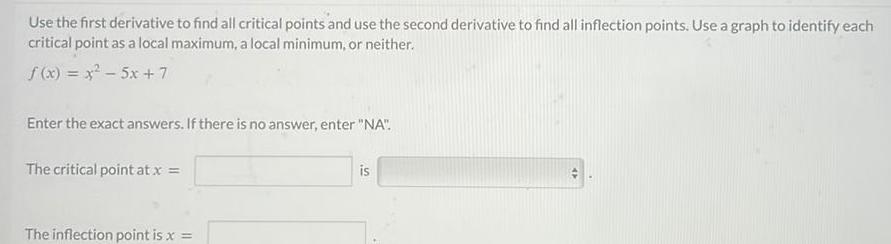
Differential equationsUse the first derivative to find all critical points and use the second derivative to find all inflection points Use a graph to identify each critical point as a local maximum a local minimum or neither f x x 5x 7 Enter the exact answers If there is no answer enter NA The critical point at x The inflection point is x is

Calculus
Vector Calculus17 20 Determine whether the lines L and L are parallel skew or intersecting If they intersect find the point of intersection 17 L x 3 2t y 4 t z 1 3t L x 1 4s y 3 2s z 4 5s z 1 3t 18 L x 5 12t y 3 9t L x 3 8s y 6s z 7 2s 19 L y 3 1 z x 2 1 2 x 3 y 4 z 2

Calculus
Application of derivatives2 5 Find a vector equation and parametric equations for the line 2 The line through the point 6 5 2 and parallel to the vector 1 3 3 3 The line through the point 2 2 4 3 5 and parallel to the vector 3i 2j k