Calculus Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Calculus
Application of derivatives1 x 1 You may use the following derivatives Let y 3 Find the values of x for which y is increasing most rapidly and decreasing most rapidly y x 2x x 1 is where y is increasing most rapidly is where y is decreasing most rapidly and y x 2 3x 1 x 1
Calculus
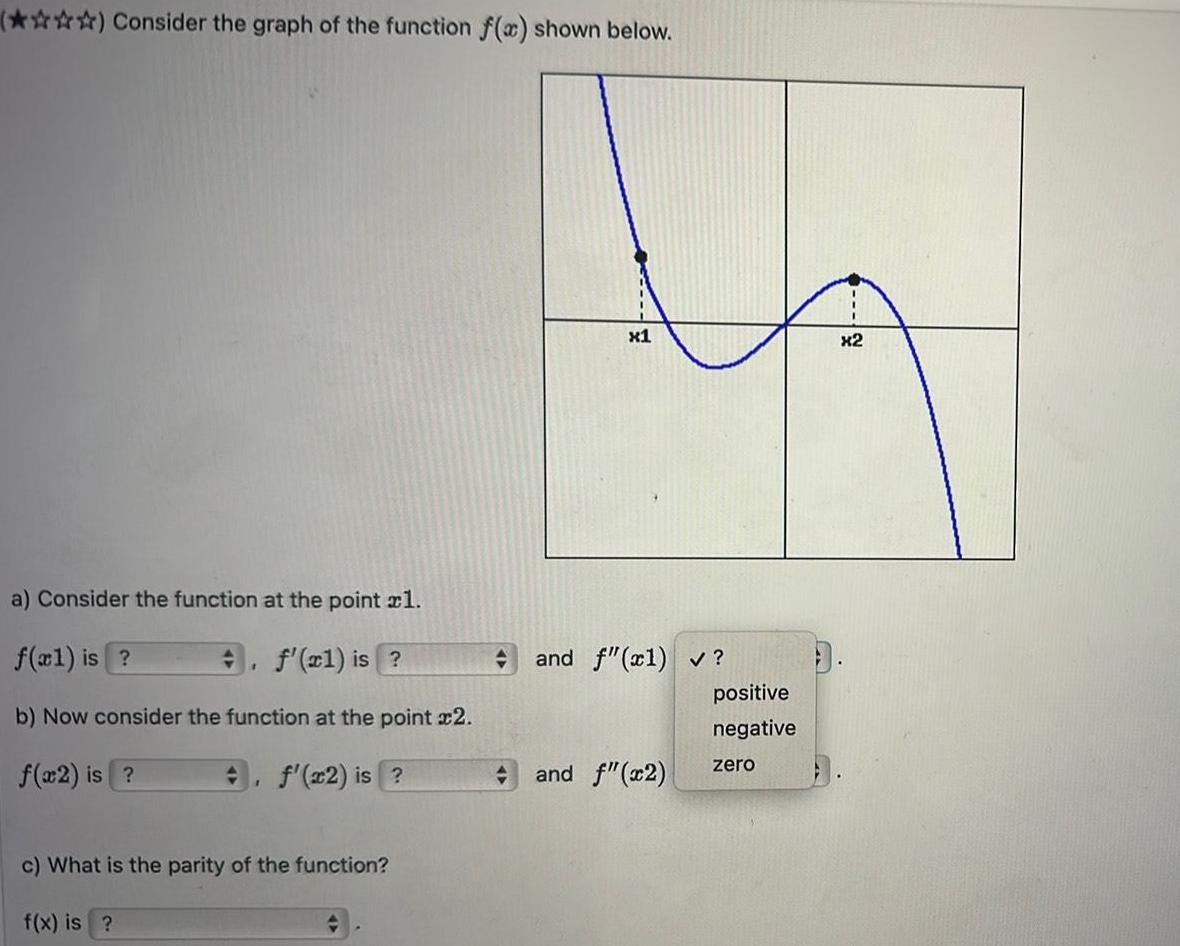
Application of derivativesConsider the graph of the function f x shown below a Consider the function at the point xl f xl is f xl is b Now consider the function at the point x2 f x2 is f x2 is 1 c What is the parity of the function f x is x1 and f x1 and f x2 positive negative zero x2


Calculus
DifferentiationIf f is the focal length of a convex lens and an object is placed at a distance p from the lens then its image will be at a distance q from the lens where f p and q are related by the lens equation 1 1 1 f p 9 What is the rate of change of p with respect to q if q 5 m and f 6 m Make sure you have the correct sign for the rate m s

Calculus
Differential equationsLet a h and k be arbitrary real numbers with a Which of the following statements are true about f Select all that apply A An extreme value occurs at the point h k B The graph of y f x is a parabola C If a 0 then f has a global minimum D The graph of y f x is a line E The function has a critical point at x h F The function has an inflection point at x h G The maximum value of f x is h H If a 0 then f has a global maximum I None of the above 0 and let f be the function defined as f x a x h k
Calculus
Application of derivatives6 e 1 e x Let f x On what interval is f increasing B ln 6 C ln 6 D ln 5 E In 5 F A In 6 G In 6 0 H In 5
Calculus
Application of derivativesGiven the following function find the x value of the critical point Determine if the function is increasing or decreasing on the given intervals and deduce what that means for the critical point a The x value of the critical point is at c b The function f x is on o c and on c oo so the critical point thus give a minimum a maximum f x x 6x 4

Calculus
Application of derivativesNewton s Law of Gravitation says that the magnitude F of the force exerted by a body of mass m on a body of mass Mis GmM 72 where G is the gravitational constant and r is the distance between the bodies F a Find d F dr What does the minus sign mean b Suppose it is known that Earth attracts an object with a force that decreases at a rate of 2 N km when 7 20000 km How fast does this force change when r 10000 km The force changes at a rate of N km
Calculus
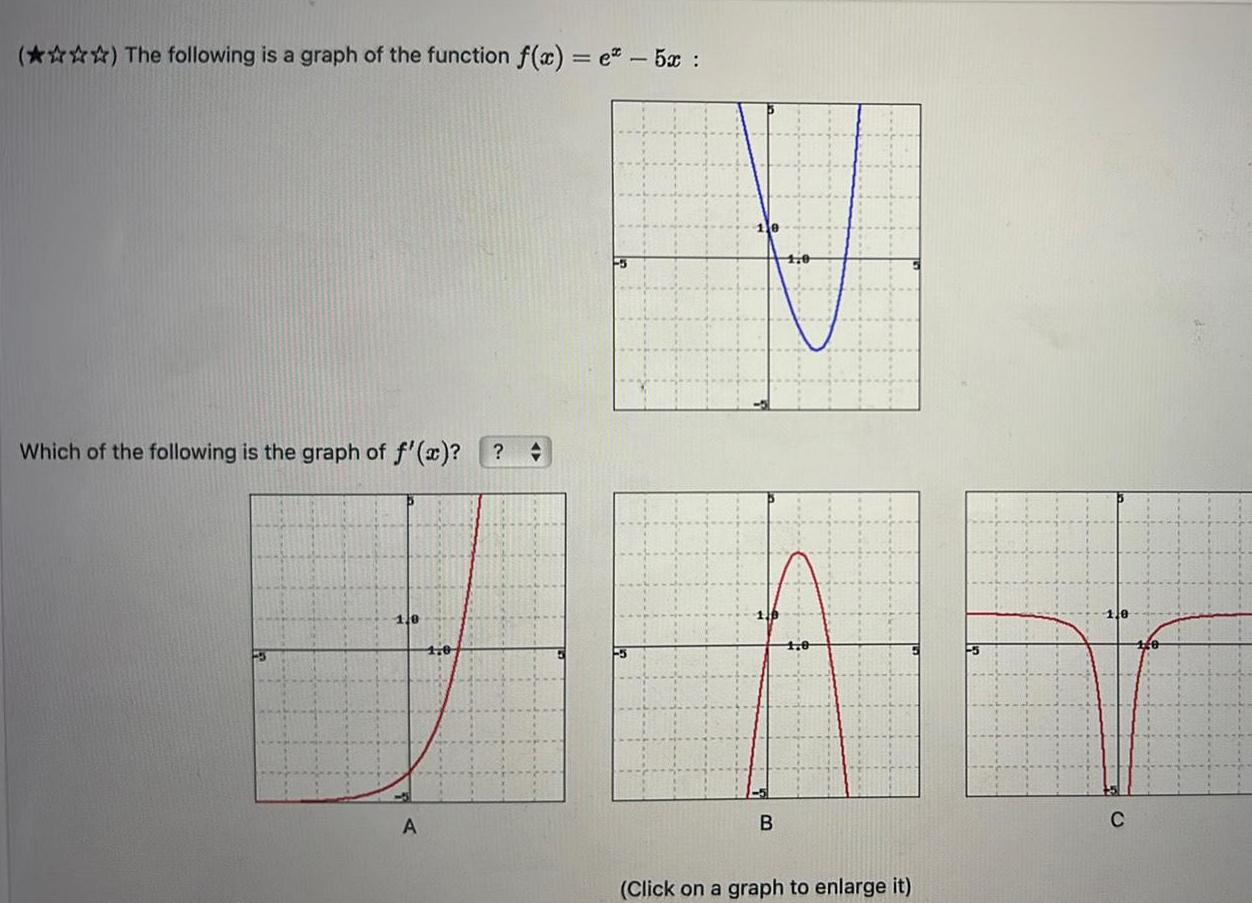
DifferentiationThe following is a graph of the function f x e 5x Which of the following is the graph of f x A B Click on a graph to enlarge it 48 C
Calculus
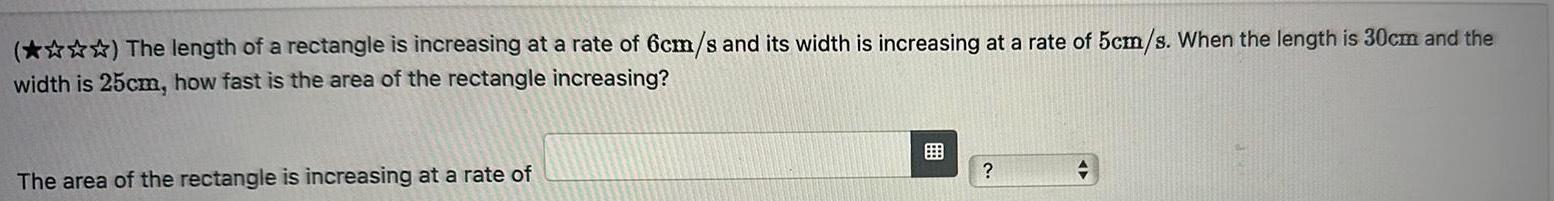
Application of derivativesThe length of a rectangle is increasing at a rate of 6cm s and its width is increasing at a rate of 5cm s When the length is 30cm and the width is 25cm how fast is the area of the rectangle increasing The area of the rectangle is increasing at a rate of

Calculus
Limits & ContinuityConsider the growth of a cell assumed to be spherical in shape Suppose that the radius of the cell r increases at a constant rate per uni time Call the constant k Express your answers in terms of the radius of the cell r and its growth rate k a At what rate does the volume V increase dV dt b At what rate does the surface area S increase dS dt c At what rate does the ratio of surface area to volume S V change d S dt V Does the ratio S V decrease or increase as the cell grows

Calculus
Application of derivativesThe pressure P and volume V of an expanding gas are related by the formula PV C where b and Care constants this model assumes adiabatic expansion without heat gain or loss Find if b 1 2 P 6kPa V 150cm and 80cm min d P dt kPa min

Calculus
Application of derivativesIn 1905 a Bohemian farmer accidentally allowed several muskrats to escape an enclosure Their population grew and spread occupying increasingly larger areas throughout Europe In a classical paper in ecology it was shown by the scientist Skellam 1951 that the square root of the occupied area increased at a constant rate k Determine the rate of change of the distance from the site of release that the muskrats had spread For simplicity you may assume that the expanding area of occupation is circular with radius r t Simplify your answer so that it only depends on k r t
Calculus
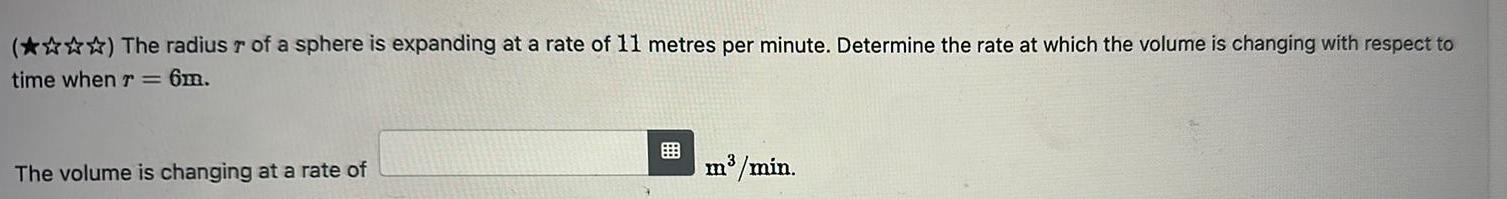
Vector CalculusThe radius r of a sphere is expanding at a rate of 11 metres per minute Determine the rate at which the volume is changing with respect to time when r 6m The volume is changing at a rate of m min
Calculus
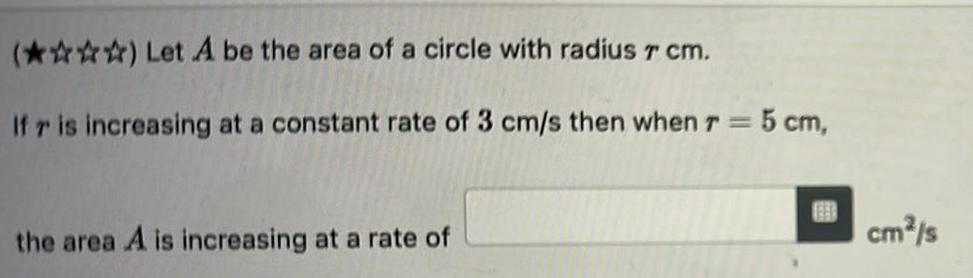
Application of derivativesLet A be the area of a circle with radius 7 cm If r is increasing at a constant rate of 3 cm s then when r 5 cm the area A is increasing at a rate of cm s
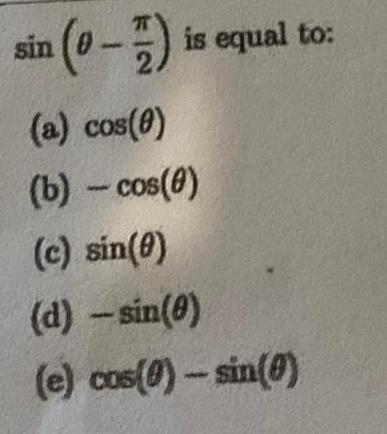
Calculus
Application of derivativessin 0 1 is equal to a cos 0 b cos 8 c sin 0 d sin 0 e cos 0 sin 0 3 E

Calculus
Application of derivativesQuestion 2 2 3 5 points a 2 points Suppose a curve is described implicitly by the equation y sin x e cos y Find the equation of the tangent line to this curve at the point 0 2

Calculus
Application of derivativesA 16 foot ladder is leaning against a wall If the top of the ladder slides down the wall at a rate of 3 feet per second how fast is the bottom of the ladder moving along the ground when the bottom of the ladder is 4 feet from the wall Leave your answer in exact form simplified as much as possible Do not include units

Calculus
DifferentiationA company plans to manufacture a rectangular container with a square base an open top and a volume of 214 cm The cost of the material for the base is 0 3 cents per square centimeter and the cost of the material for the sides is 0 5 cents per square centimeter Determine the dimensions of the container that will minimize the cost of manufacturing it What is the minimum cost Round any calculations and the final answer to the nearest hundredth if necessary

Calculus
Application of derivativesA company plans to manufacture a rectangular box with a square base an open top and a volume of 458 in The cost of the material for the base is 0 5 cents per square inch and the cost of the material for the sides is 0 4 cents per square inch Determine the dimensions of the box that will minimize the cost of manufacturing it What is the minimum cost per box rounded to the nearest cent Round any calculations and the final answer to the nearest hundredth if necessary C

Calculus
DifferentiationYou and a friend both leave the same restaurant to drive home You are heading directly west at 30 miles per hour and he or she is heading directly south at 40 miles per hour After half an hour how fast in mph is the distance between you changing Do not include units in your answer

Calculus
Application of derivativesA box with a square base and open top must have a volume of 2500 cm What is the minimum possible surface area in cm of this box Round to the nearest whole number and omit units

Calculus
Limits & ContinuityQuestion A car is travelling due north at a speed of 46 miles per hour If the car started off 153 miles directly west of the city of Smithville how fast is the distance between the car and Smithville changing when the car has travelled 137 miles Do include units in your answer and round to the nearest hundredth Provide your answer below
Calculus
Vector CalculusThe 15 tenure track professors in a university math department are paid an average of 90 000 per year while the 10 adjunct professors are paid an average of 60 000 per year What is the average salary among all these math professors

Calculus
Application of derivativesA rectangular bin is going to be made with a volume of 492 in The base of the bin will be a square and the top will be open The cost of the material for the base is 0 8 cents per square inch and the cost of the material for the sides is 0 6 cents per square inch Determine the dimensions of the bin that will minimize the cost of manufacturing it What is the minimum cost Round any calculations and the final answer to the nearest hundredth if necessary Provide your answer below
Calculus
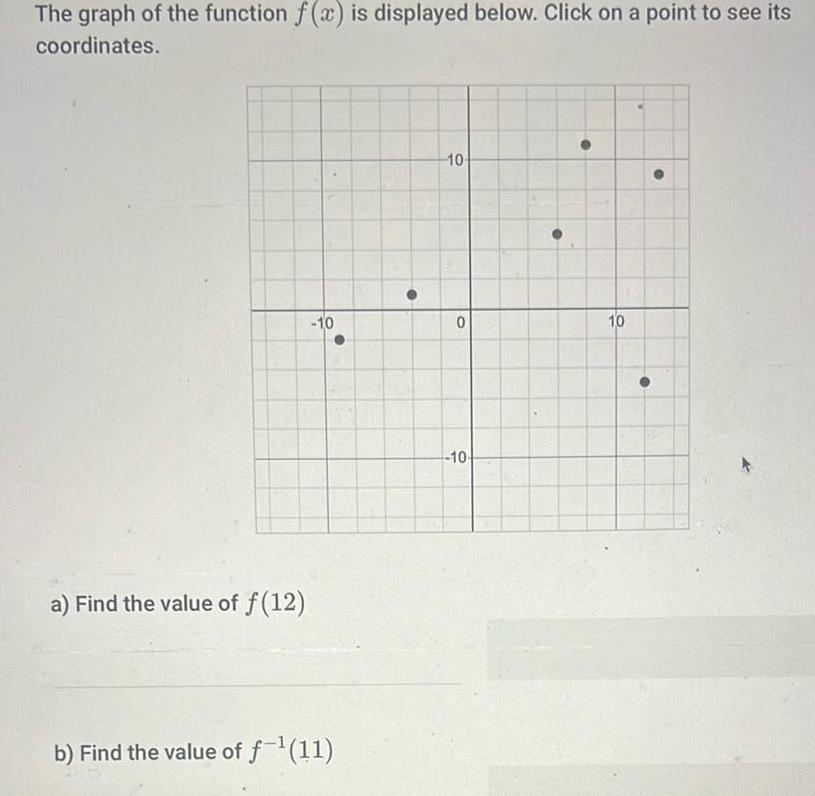
Vector CalculusThe graph of the function f x is displayed below Click on a point to see its coordinates a Find the value of f 12 10 b Find the value of f 11 10 0 10 10
Calculus
Application of derivativesBeginning 34 miles directly west of the city of Hartville a van travels due north The van is travelling at a speed of 52 miles per hour Let 0 be the angle that is opposite the northward path Determine the rate of change of 0 in radians per hour when the van has been travelling for 31 miles Submit an exact answer in fractional form Provide your answer below d0 dt rad h 0

Calculus
Application of derivativesA cylindrical can is partially filled with sand The radius of the cylindrical can is 15 cm The height of the cylindrical can is 14 cm If the volume of the sand is increasing at a rate of 555 cubic cm per second what is the rate in cm per second at which the height of the sand is changing when the height of the sand is 5 cm Submit an exact simplified answer Remember that the volume of a cylinder is V r h Provide your answer below

Calculus
Differential equations2 Find a polar equation for the curve represented by the Cartesian equation 2x y 1

Calculus
Application of derivativesOwners of a car rental company that charges customers between 60 and 175 per day have determined that the number of cars rented per day n can be modeled by the linear function n p 1050 6p where p is the daily rental charge How much should the company charge each customer per day to maximize revenue Do not include units or a dollar sign in your answer Provide your answer below



Calculus
Application of derivativesQuestion the A cylindrical can is partially filled with water The radius of the cylindrical can is 8 inches and its height is 9 inches If volume of the water is decreasing at a rate of 527 cubic inches per minute what is the rate in inches per minute at which the height of the water is changing when the height of the water is 5 inches Submit an exact answer Do not forget to include a negative sign if the height is decreasing Remember that the volume of a cylinder is V r h Provide your answer below
Calculus
Definite IntegralsCurrent Attempt in Progress The concentration C in ng ml of a drug in the blood as a function of the time t in hours since the drug was administered is given by C 15te 0 21 The area under the concentration curve is a measure of the overall exposure of a person to the drug Find the total exposure provided by the drug between t 0 and t 5 Round your answer to two decimal places Exposure Modia ng ml hours Attempts 0 of 5 used Submit Answer

Calculus
Application of derivativesA circle has a radius that is decreasing at a rate of 18 mm per minute What is the rate of change of the area of the circle when the radius is 4 mm Do not include the units in your answer
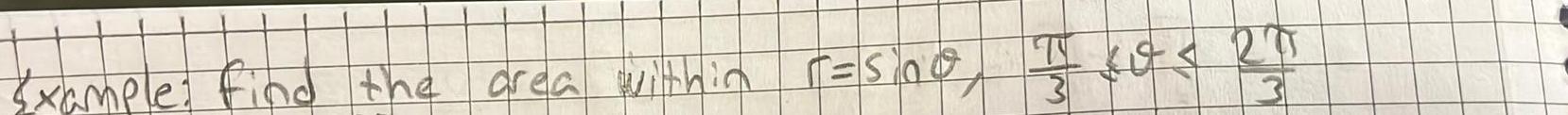
Calculus
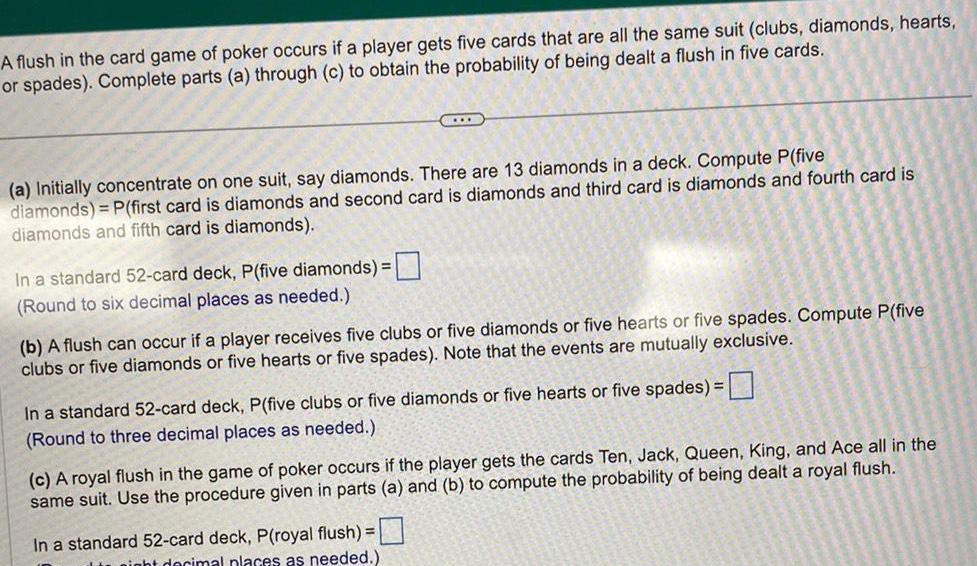
Vector CalculusA flush in the card game of poker occurs if a player gets five cards that are all the same suit clubs diamonds hearts or spades Complete parts a through c to obtain the probability of being dealt a flush in five cards a Initially concentrate on one suit say diamonds There are 13 diamonds in a deck Compute P five diamonds P first card is diamonds and second card is diamonds and third card is diamonds and fourth card is diamonds and fifth card is diamonds In a standard 52 card deck P five diamonds Round to six decimal places as needed b A flush can occur if a player receives five clubs or five diamonds or five hearts or five spades Compute P five clubs or five diamonds or five hearts or five spades Note that the events are mutually exclusive In a standard 52 card deck P five clubs or five diamonds or five hearts or five spades Round to three decimal places as needed c A royal flush in the game of poker occurs if the player gets the cards Ten Jack Queen King and Ace all in the same suit Use the procedure given in parts a and b to compute the probability of being dealt a royal flush In a standard 52 card deck P royal flush night decimal places as needed

Calculus
Application of derivativesUse the parent function f x b and transformation techniques to graph h x 4 2 2 Also indicate which transformation s is are applied Determine the domain range and horizontal asymptote of h Select all transformations that apply Shift the graph of f 2 units to the left Shift the graph of f 2 units down Shift the graph of f 2 units up Reflect the graph of f about the y axis Horizontally stretch the graph of f by a factor of 2 Reflect the graph of f about the x axis Vertically stretch the graph of f by a factor of 2 Shift the graph of f 2 units to the right Vertically compress the graph of f by a factor of 2 Horizontally compress the graph of f by a factor of 2
Calculus
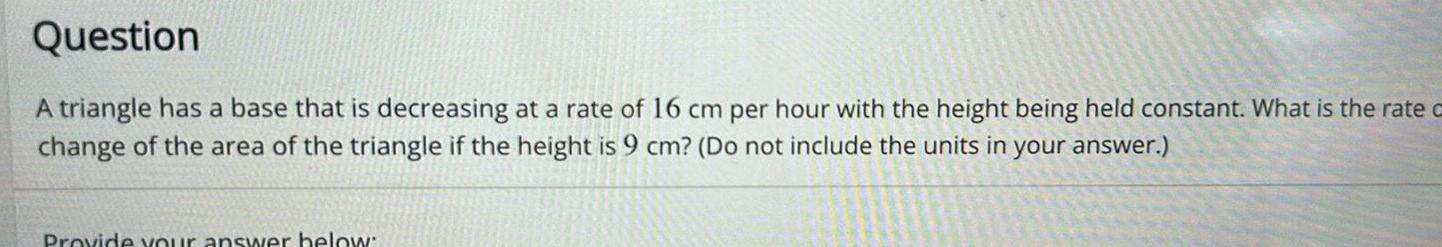
Application of derivativesQuestion A triangle has a base that is decreasing at a rate of 16 cm per hour with the height being held constant What is the rate o change of the area of the triangle if the height is 9 cm Do not include the units in your answer Provide your answer below

Calculus
Application of derivativesFor a dosage of x cubic centimeters cc of a certain drug the resulting blood pressure B is approximated by the function below Find the maximum blood pressure and the dosage at which it occurs B x 355x 1420x 0 x 0 25 The maximum is obtained for a dosage of 0 17 Round to two decimal places as needed The maximum blood pressure obtained is Round to two decimal places as needed

Calculus
Application of derivativesFor a dosage of x cubic centimeters cc of a certain drug the resulting blood pressure B is approximated by the function below Find the maximum blood pressure and the dosage at which it occurs B x 355x 1420x 0 x 0 25 The maximum is obtained for a dosage of Round to two decimal places as needed
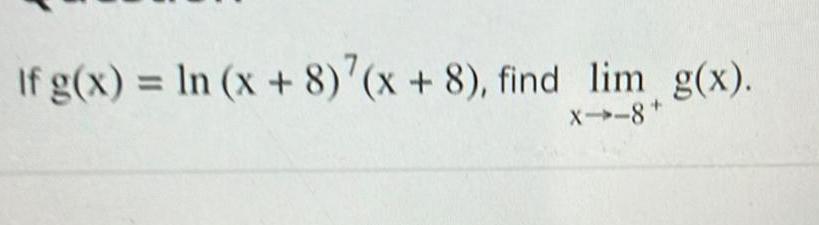
Calculus
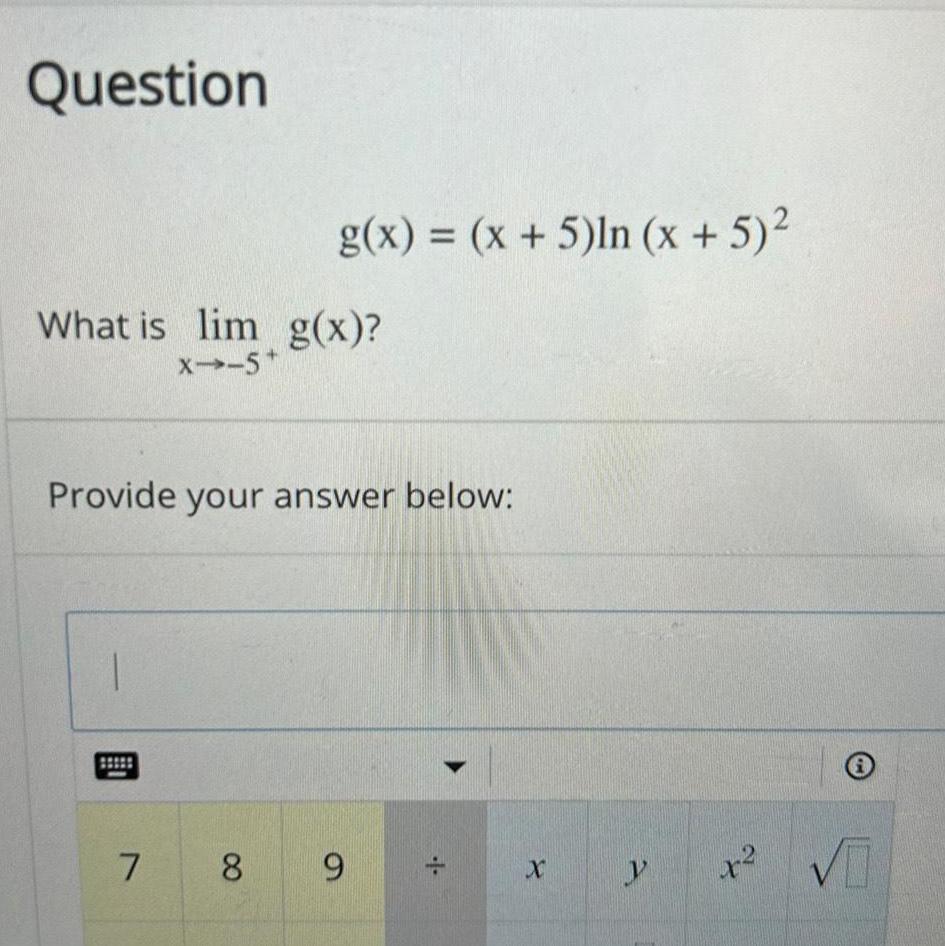
Limits & ContinuityQuestion g x x 5 ln x 5 What is lim g x X 5 Provide your answer below 7 8 9 4 X V y 6 4 VO
Calculus
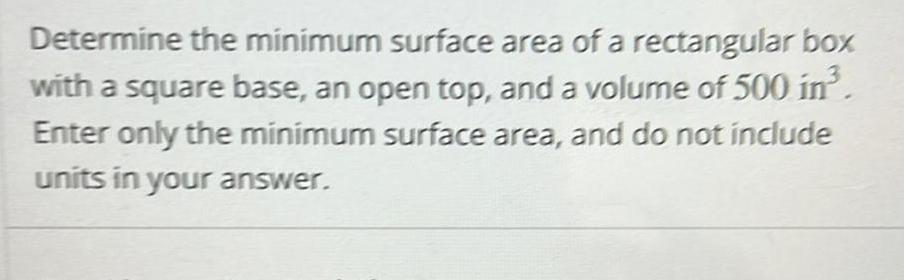
Application of derivativesDetermine the minimum surface area of a rectangular box with a square base an open top and a volume of 500 in Enter only the minimum surface area and do not include units in your answer

Calculus
Application of derivativesA lifeguard needs to rope off a rectangular swimming area in front of Long Lake Beach using 800 yd of rope and floats What dimensions of the rectangle will maximize the area What is the maximum area Note that the shoreline is one side of the rectangle Let x be the length of a side of the rectangle perpendicular to the shoreline Write the objective function for the area in terms of x A x Type an expression using x as the variable The length of the shorter side of the rectangular region is The length of the longer side of the rectangular region is The maximum area of the rectangular region is
Calculus
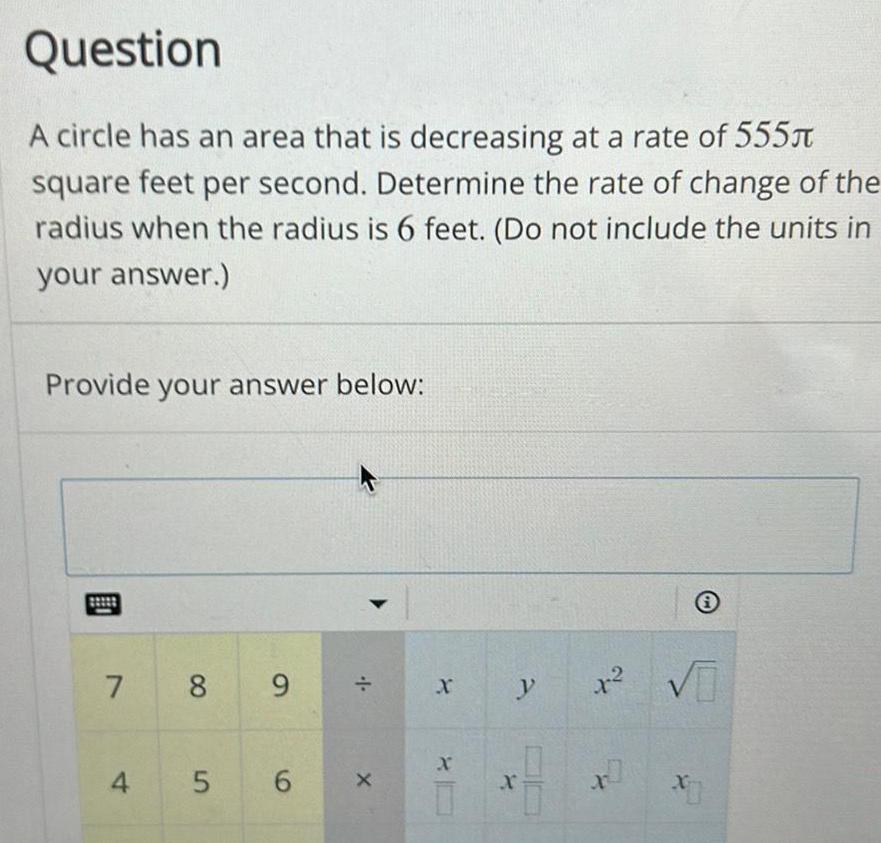
Application of derivativesQuestion A circle has an area that is decreasing at a rate of 555 square feet per second Determine the rate of change of the radius when the radius is 6 feet Do not include the units in your answer Provide your answer below 7 8 9 4 5 6 X X X y X x X
Calculus
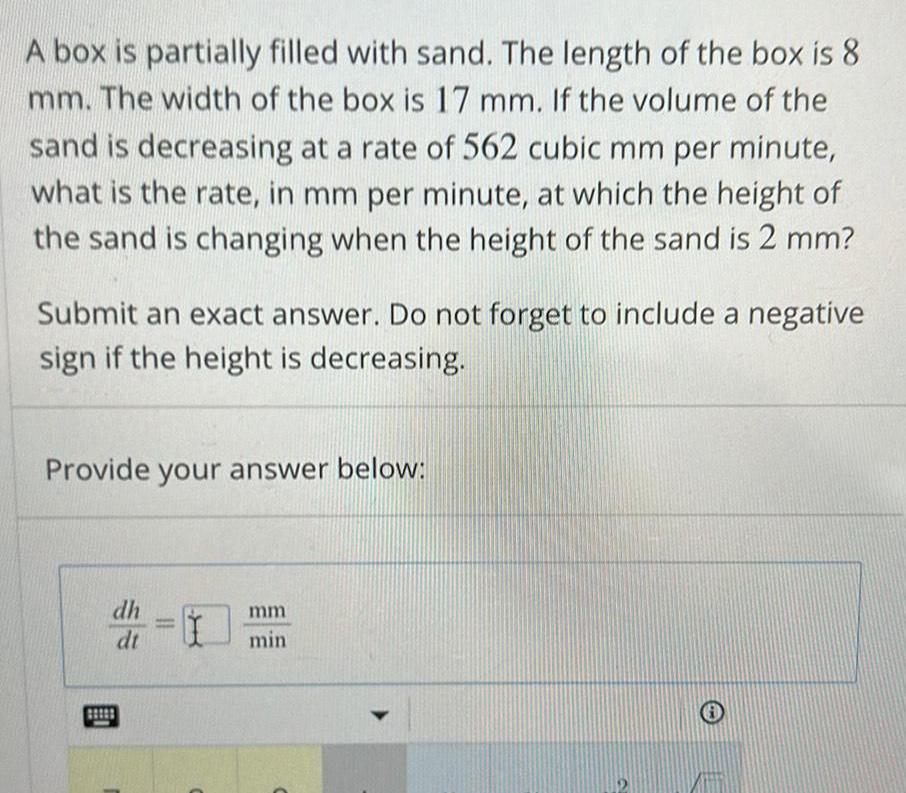
Application of derivativesA box is partially filled with sand The length of the box is 8 mm The width of the box is 17 mm If the volume of the sand is decreasing at a rate of 562 cubic mm per minute what is the rate in mm per minute at which the height of the sand is changing when the height of the sand is 2 mm Submit an exact answer Do not forget to include a negative sign if the height is decreasing Provide your answer below dh dt 1 mm min Y
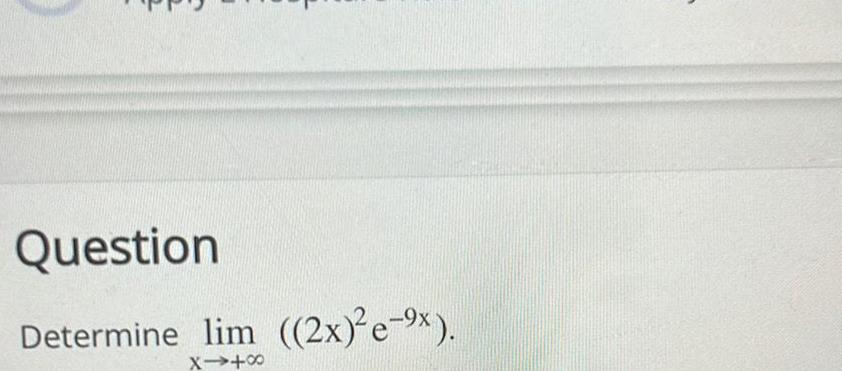
Calculus
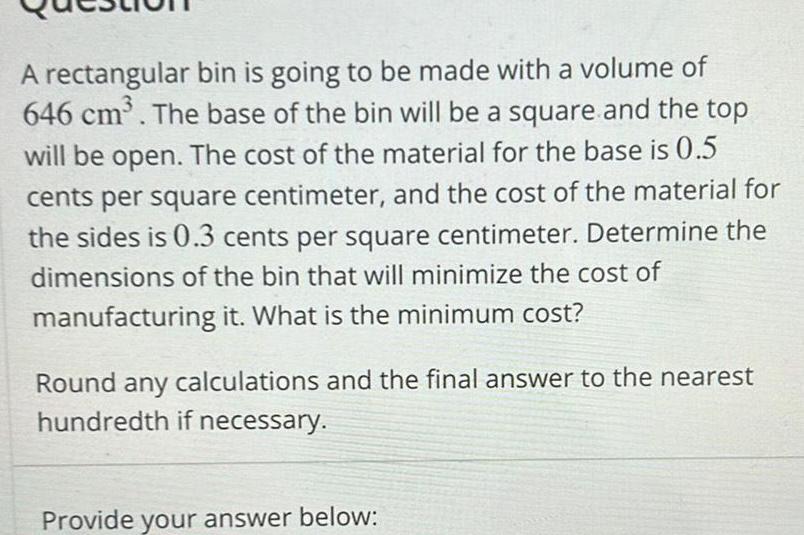
Application of derivativesA rectangular bin is going to be made with a volume of 646 cm The base of the bin will be a square and the top will be open The cost of the material for the base is 0 5 cents per square centimeter and the cost of the material for the sides is 0 3 cents per square centimeter Determine the dimensions of the bin that will minimize the cost of manufacturing it What is the minimum cost Round any calculations and the final answer to the nearest hundredth if necessary Provide your answer below

Calculus
Application of derivativesAn employee s monthly productivity M in number of units produced is found to be a function of the number t of years of service For a certain product a productivity function is shown below Find the maximum productivity and the year in which it is achieved M t 3t 1441 140 0st 40