Matrices & Determinants Questions and Answers

Algebra
Matrices & DeterminantsSuppose you have been given the following set of data representing the test scores of 10 college algebra students 78 92 85 67 88 94 72 90 79 81 a Calculate the mean of the test scores of the students 753820 18 92 85 67 88 94 42 90 79 81 b Calculate the median of the test scores of the students 67 72 78 79 81 85 88 90 92 94 85 81 83 82 6 c Suppose the lowest score 67 was an outlier due to an error in grading Calculate the revised mean and median for the data set after removing the outlier How do these values change and what does this indicate about the impact of outliers
Algebra
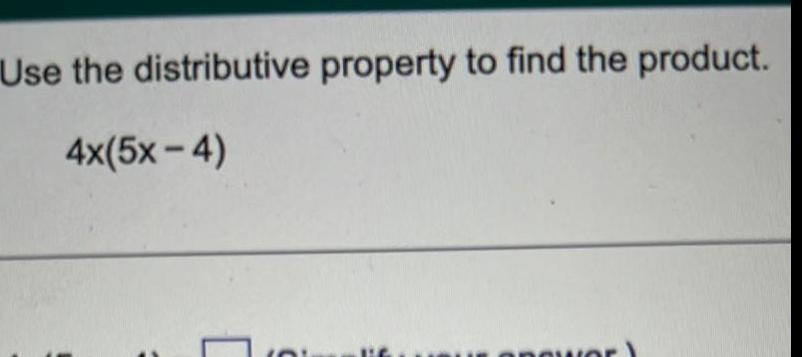
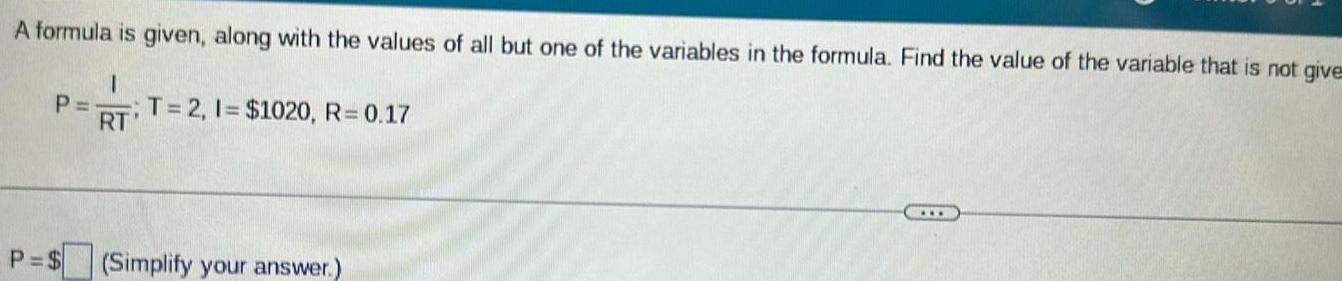
Matrices & DeterminantsA formula is given along with the values of all but one of the variables in the formula Find the value of the variable that is not give 1 P T 2 1 1020 R 0 17 RT P Simplify your answer
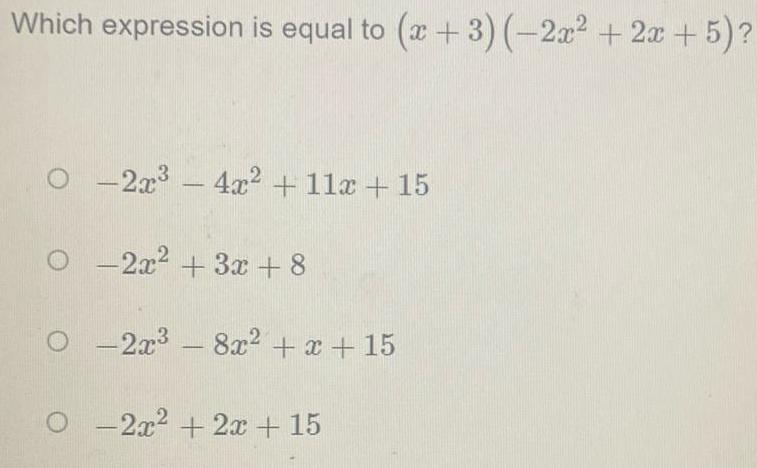
Algebra
Matrices & DeterminantsWhich expression is equal to x 3 2x 2x 5 O 2x 4x 11x 15 O 2x 3x 8 O 2x 8x x 15 O 2x 2x 15
Algebra
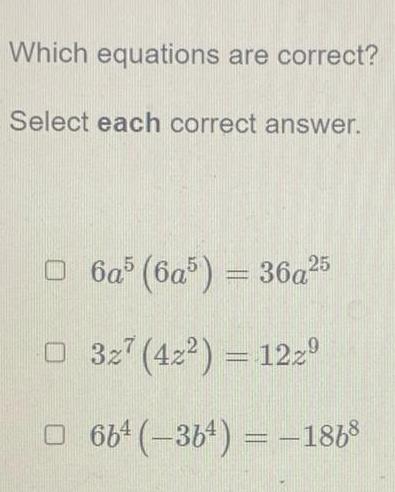
Matrices & DeterminantsWhich equations are correct Select each correct answer 6a5 6a5 36a 5 3z7 42 122 664 364 1868
Algebra
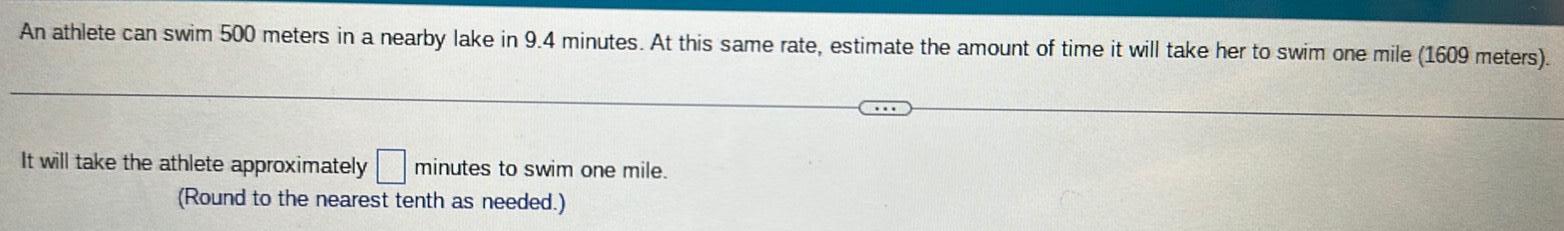
Matrices & DeterminantsAn athlete can swim 500 meters in a nearby lake in 9 4 minutes At this same rate estimate the amount of time it will take her to swim one mile 1609 meters It will take the athlete approximately minutes to swim one mile Round to the nearest tenth as needed
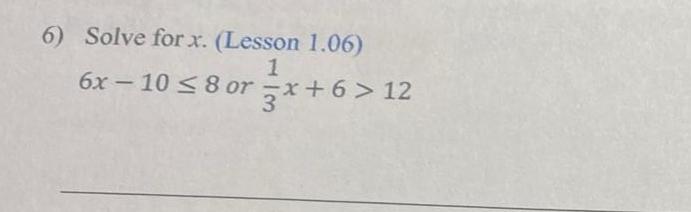

Algebra
Matrices & Determinantsb 1 to b t b k20 c xkZ is uniformly Cauchy on t b t b verification of these properties is left for the reader We consider the uniform limit of the sequence x 1 8 to 8 B x b C which is given by x t lim x 1 Thus x t lim xo k 00 40 D 1 0 1 2 x lim x dx dt x 1 x MKk k 1 to 0 10 X 1 x 0 1e1 b t b ff s lim x s ds x Thus the function rx 1 satisfying the integral equation dt f s x s ds ff s x s ds Finally the validity of this integral equation has the following two implications x ff s x s ds for all t t b t tb f s x s ds x 0 x ff s x s ds lim f s x s ds f s x s ds f t X t 20 2 3 UNIQUENESS OF A SOLUTION This now proves that the curve X 1 8 1 82 thus obtained is a solution of the initial value problem s ds Chapter 2 Systmes of First Order ODE We prove an inequality which will lead us to the uniqueness of the solutions Proposition 2 Gronwall s Inequality Let f a b 0 g a b 000 be continuous functions and A 0 a constant satisfying fi A ff fsgs ds for all te a b Then ft A e Proof First we assume A 0 and put ht A te ab Then ht 0 for all te a b and h t f t g t for all 1 a b Sf fsgs ds for all
Algebra
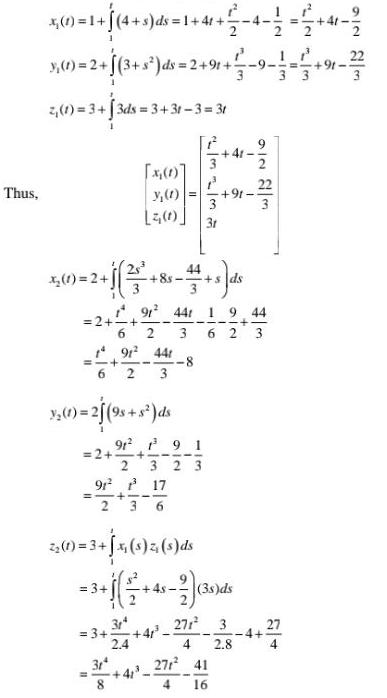
Matrices & DeterminantsThus x 1 4 5 1 4 4 444 4 3 0 2 1 3 2 9 9 2 1 3 3ds 3 31 3 3r 2 6 191 441 2 3 6 5 00 2 1 8 1 91 441 19 44 2 3 623 32 0 25 9s ds x 1 y 1 500 3 91 191 2 1 1 2 323 3 91 17 2 3 6 2 1 3 x s z s ds 31 44 31 2 4 8 3r 41 91 38 ds 271 41 4 16 41 271 3 4 2 8 22 4
Algebra
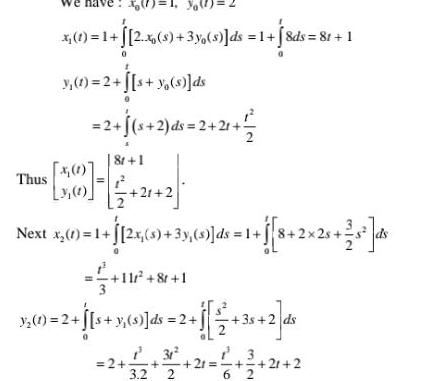
Matrices & Determinants300 1 12400 300 ds 1 j8dx 8r 1 y t 2 s y s ds Thus 2 x 2 2 2 8r 1 30 21 2 Next x 1 1 2x s 3y s ds 1 8 2 2s 25 ds 3 1 2 s x 6 ds 2 3x 2 ds 2 1 3 21 21 2 6 2 2 3 2 2

Algebra
Matrices & Determinants10 1000 0100 0001 0010 Perform a spectral decomposition on both matrices form UDU where D is diagona matrix U is unitary matrix


Algebra
Matrices & Determinantscourse the ODE has order k Note that the function X t X t is a vector valued function of the real variable t and as such it is a curve in R Each X has n components X t X 1 X 1 X t and therefore all the derivative of it has n components X 1 X 1 d for 1 l k Consequently the function f appearing on the right hand side of 3 has n components f f f f each f being a real valued function d X 1 dt MATHEMATICS Consequently the DE 3 is actually the following system of ODE in the functions t X t t X t t X t dk X dit 1 dk 1X d x f X d X di di d X dik t X t 1 X 1 X dx dt d X dt dX dt dX dt t dX At this stage we become more specific about the features of the ODE 3 or equivalently about the system 4 Let I be an open internal and let 2 denote an open subset of R We consider the open sets IxNxR x XR there being k 1 copies of R in the above Cartesian product This set is being designated to accommodate the variable quantities d x dr 1 3 dk X dit 1 1 X Clearly the function f appearing on the right hand side of 3 must have this set as its domain of definition dX dt Choosing 1 1 x En and w w w in R we form the initial condition fox W W W Now the initial value problem for the ODE 3 is the following pair 4 dk X 5

Algebra
Matrices & DeterminantsProof Recall that both x and y being solutions of the initial value problem satisfy the integral equations on their domain intervals x 1 xo f s x s ds y t x ff s y s ds Therefore x t y t Sis s x s f s y s ds which implies x 1 y 1 0 s x s s y s 0 Kx s y s for all t te Applying Gronwalls result with A 0 we get 0 x 1 y 1 0 for all t t This gives the desired equality x t y t for all tJJ Towards the uniqueness of the solution of the initial value problem 3 we consider all the solutions of the initial value problem 3 Let the totality of them be denoted by x J XEA the solutions x being thus indexed by a suitable indexing set A Above we have verified that any two solutions say x and x are equal on the overlap of their domains Therefore we patch together all the solutions to get a maximal solution is the solution defined on the largest open interval It is obtained as follows Let J U J 2 A clearly Jis an open sub internal of I with XJ and all the solutions x patch up to get a solution x J of the initial value problem 3
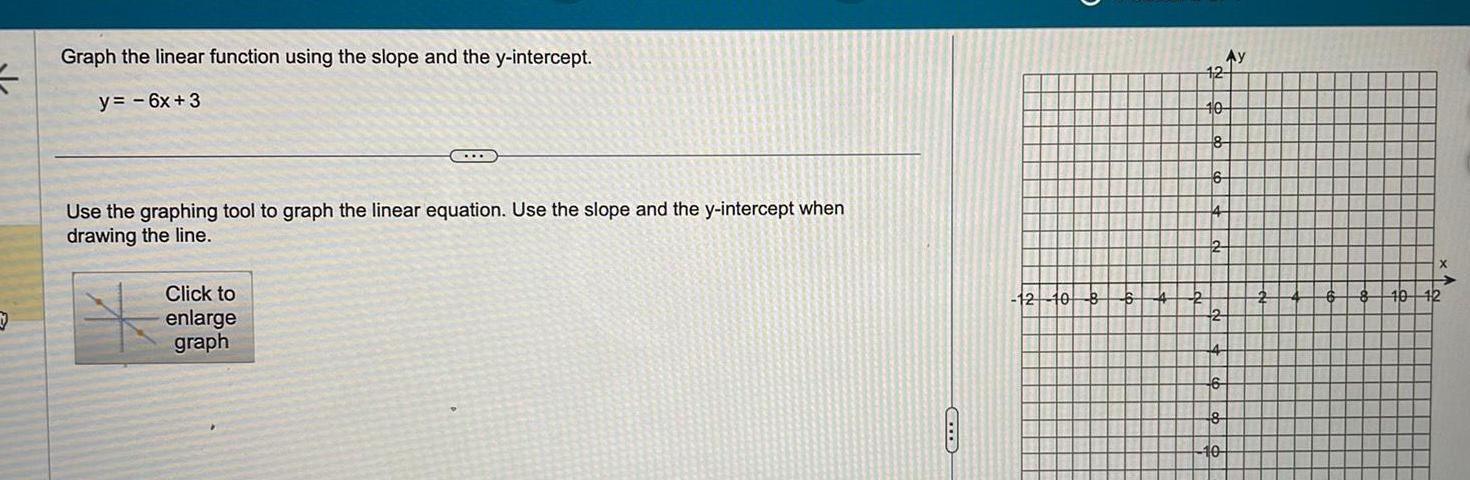
Algebra
Matrices & DeterminantsD Graph the linear function using the slope and the y intercept y 6x 3 Use the graphing tool to graph the linear equation Use the slope and the y intercept when drawing the line Click to enlarge graph 12 10 12 10 18 6 Ay 48 10 10 42

Algebra
Matrices & DeterminantsFind the equation for the line shown on the right What is the equation for the line shown on the right 10 B Ay 10
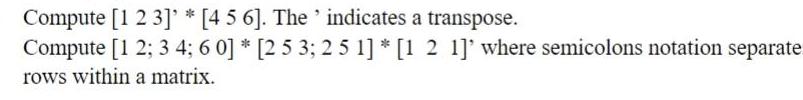
Algebra
Matrices & DeterminantsCompute 1 2 3 4 5 6 The indicates a transpose Compute 1 2 3 4 6 0 2 5 3 2 5 1 1 2 1 where semicolons notation separate rows within a matrix

Algebra
Matrices & DeterminantsUse an identity to find the value of the expression Do not use a calculator sec 359 tan 359 sec 359 tan 359 Type an exact answer Simplify your answer

Algebra
Matrices & DeterminantsCan a 2x2 system of linear equations have exactly two solutions Explain why or why not by describing the 3 types of linear systems and their solutions Suggested vocabulary Point of intersection Slope Lines Equation

Algebra
Matrices & Determinantsa Silva looks down at her computer on the floor with an angle of depression being 30 degrees Since she knows she is 5 feet tall how far away is the computer
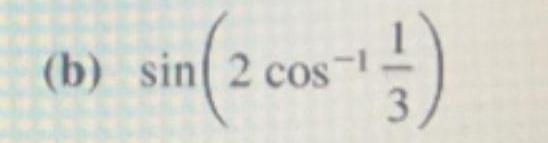

Algebra
Matrices & Determinants1 Exercise 7 2 A plane P in R is defined by x 2y 3y 0 It has v 2 and w b For u 1 3 T vectors a Find 3 vectors v and w so that v v and w w are orthogonal bases for P there are many possible answers as basis 1 B 1 compute Projp u in terms of the bases v v and w w that you found in part a i e find scalars a b and c d for which Projp u av bv and Projp u cw dw c Consider the two forms for Projp u that you derived in part b Show that they describe the same vector

Algebra
Matrices & DeterminantsThe length of a rectangular garden is 7 meters longer than its width If the garden s perimeter is 190 meters what is the area of the garden in square meters m

Algebra
Matrices & DeterminantsSuppose A is an invertible matrix with A LU where L is lower triangular and U is upper triangular In terms of L U please find L and U such that A U L U is upper triangular L is lower triangular
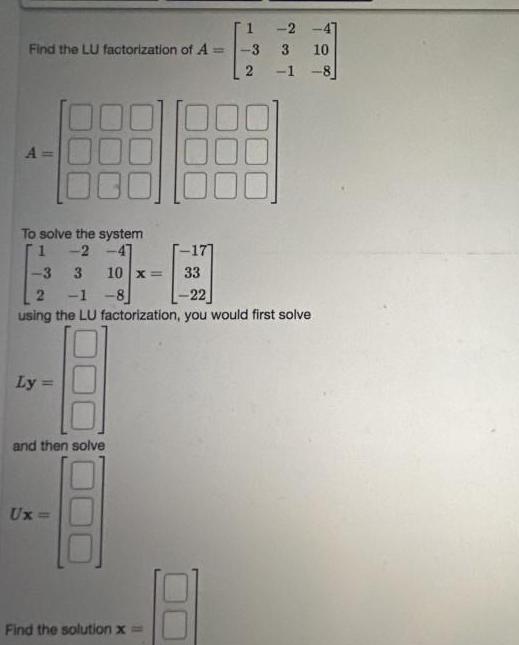
Algebra
Matrices & DeterminantsFind the LU factorization of A Ly To solve the system 1 2 47 3 3 2 1 8 22 using the LU factorization you would first solve and then solve Ux 10 x 33 18 2 41 3 10 Find the solution x 3 2 1
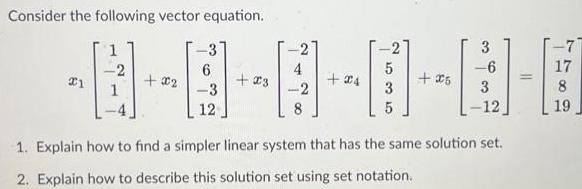
Algebra
Matrices & DeterminantsConsider the following vector equation 1 1 2 002 3 6 3 12 23 2 24 3 5 25 3 6 3 12 1 Explain how to find a simpler linear system that has the same solution set 2 Explain how to describe this solution set using set notation 7 17 8 19

Algebra
Matrices & Determinantsavail We have to drink dirty water The colour and smell of the water shows that it needs has not been cleaned for two mo cleaning It is requested to look into the matter and do the needful With best regards Yours obediently Kunal Kalara Class XI C The Principal Govt Senior Secondary School Sardarshahar babara bject Con 9 Non availability of Towel and Soap at Washbasin You are student of Gout Senior Secondary School Sardarshahar There are no towels and ons at the washbasine of the toilets Write a letter to the principal of your school to make the proper arrangement of towels and soaps Ans Near Post Office Sardarshahar 18 December 20 Subject For non availability of towels and soaps at the washbasins of the toilets Sir 40 Spectfulls Midday me ater in th Thursd at burnt a Students requested With best Yours fai Aradhya Presiden Student Respectfully I want to bring into your kind attention to the problem of non availability of soups and towels for washing and wiping hands at the washbasins of toilets There are no soaps at the washbasins outside the toilets Sometimes there are towels but they are dirty and stinking It is very difficult for the students to wash hands properly and then wipe on such a dirty towel It is requested to look into the matter and make the arrangement of clean towels and soaps as early as possible 11 A L You are sit in t Fort F Bundi 15 No
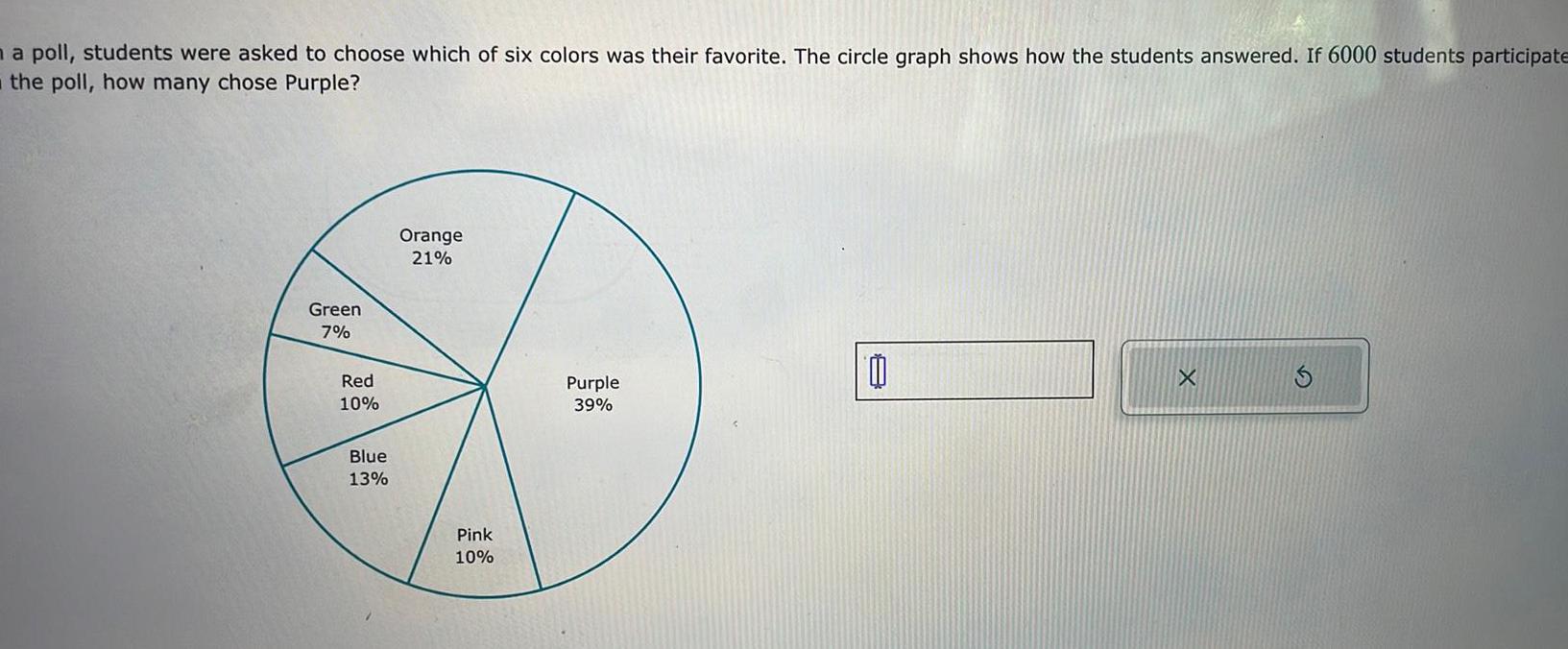
Algebra
Matrices & Determinantsna poll students were asked to choose which of six colors was their favorite The circle graph shows how the students answered If 6000 students participate the poll how many chose Purple Green 7 Red 10 Blue 13 Orange 21 Pink 10 Purple 39 00 X

Algebra
Matrices & Determinants7 Graph the inequality Label at least two points and Lesson 1 07 6x 2y 8 10 8 6 4 2 10 8 6 4 2 2 4 6 8 10 2 4 LO 6 8 10
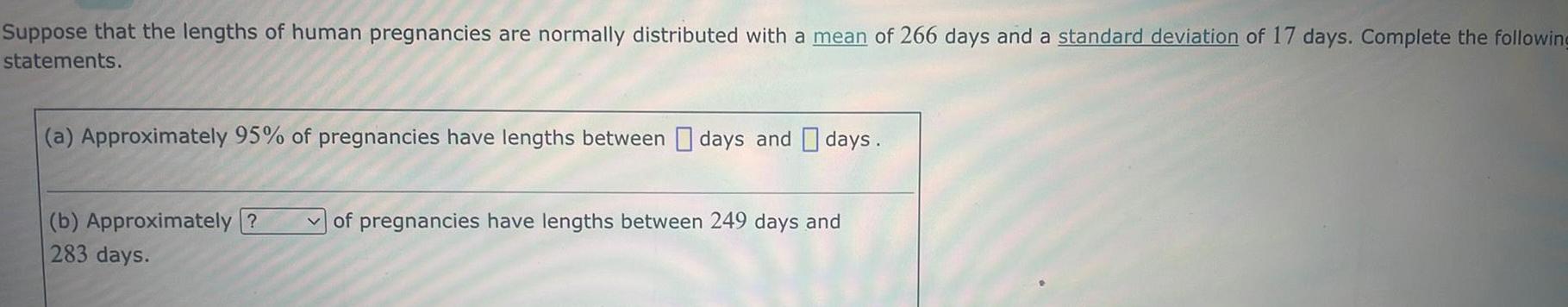
Algebra
Matrices & DeterminantsSuppose that the lengths of human pregnancies are normally distributed with a mean of 266 days and a standard deviation of 17 days Complete the following statements a Approximately 95 of pregnancies have lengths between days and days b Approximately 283 days of pregnancies have lengths between 249 days and

Algebra
Matrices & DeterminantsLet G be the set of 2 x 2 matrices with integer coefficients in the range 0 hrough 7 odd integer determinants and binary operation given by ordinary multiplication followed by reduction of each entry mod 8 Your task is to rove that G is a group Follow these four steps a Prove closure is satisfied b Prove associativity is satisfied c Prove that there is an identity element d Prove that each element has an inverse

Algebra
Matrices & DeterminantsEither use an appropriate theorem to show that given set W is a vector space or find a specific example to contrary a b 8 b a b c 2 b 2d 5 d b 3d d b d are real

Algebra
Matrices & Determinants9 Three questions are asked to some US households when the U S Bureau of Labor Statistics BLS conducts a nationwide random survey to determine who is employed and who is not employed Based on information below what is the unemployment rate What about the labor force participation rate Questions Yes 1 Are you under 16 or institutionalized 10 million 2 Are you currently working 40 million 3 Have you been actively seeking work in the past four 8 million weeks No 50 million 10 million 2 million
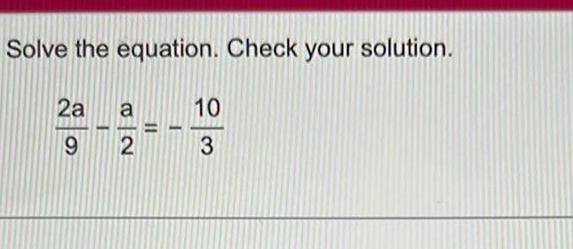
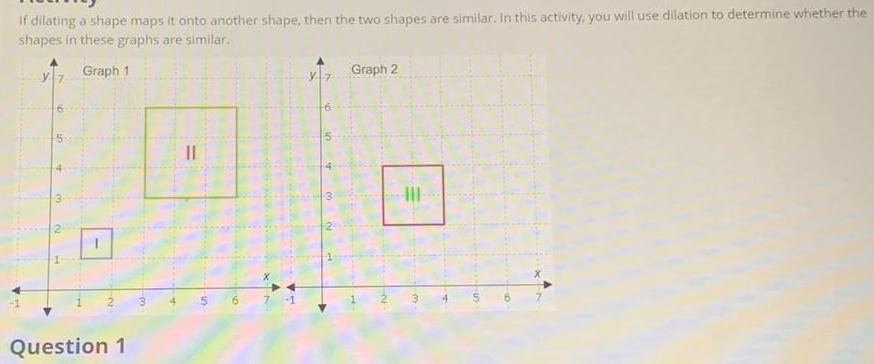
Algebra
Matrices & Determinantsif dilating a shape maps it onto another shape then the two shapes are similar In this activity you will use dilation to determine whether the shapes in these graphs are similar Graph 1 Question 1 3 5 D 7 Hi A 3 Graph 2 III a

Algebra
Matrices & DeterminantsWhich of the following graphs are functions Select all t apply A B 64444 12345 5482 11145 C D E F

Algebra
Matrices & Determinantsoblem 7 Use Gaussian elimination to find a row echelon form not reduced row echelon form of the augmented matri for the following system and then use it to determine for which value of a the following system has infinitel many solutions x 2y 3z 1 x 2y 7 2x 3y az 0
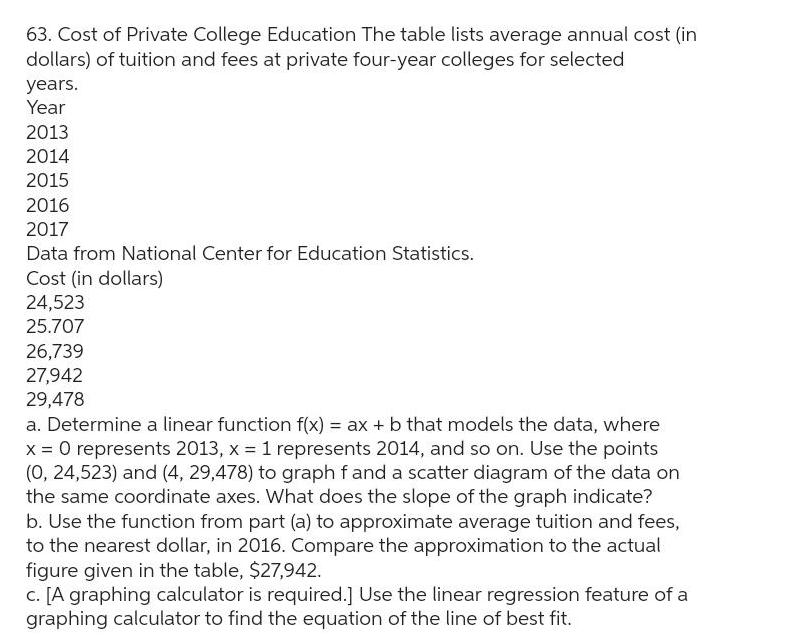
Algebra
Matrices & Determinants63 Cost of Private College Education The table lists average annual cost in dollars of tuition and fees at private four year colleges for selected years Year 2013 2014 2015 2016 2017 Data from National Center for Education Statistics Cost in dollars 24 523 25 707 26 739 27 942 29 478 a Determine a linear function f x ax b that models the data where x 0 represents 2013 x 1 represents 2014 and so on Use the points 0 24 523 and 4 29 478 to graph f and a scatter diagram of the data on the same coordinate axes What does the slope of the graph indicate b Use the function from part a to approximate average tuition and fees to the nearest dollar in 2016 Compare the approximation to the actual figure given in the table 27 942 c A graphing calculator is required Use the linear regression feature of a graphing calculator to find the equation of the line of best fit

Algebra
Matrices & DeterminantsD for the matrix A 01 4 1 is No Decomposition possible NO Find pumutation matrix P such tha PA LU for come lower I upper triangular makine 13 3 PAZLU mu
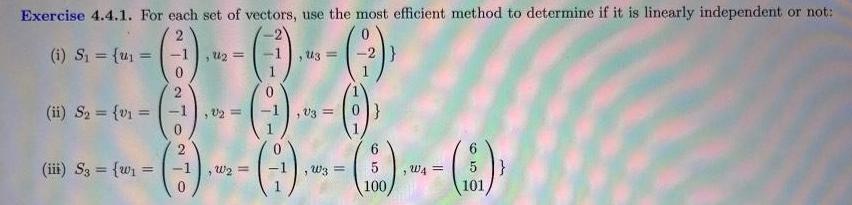
Algebra
Matrices & DeterminantsExercise 4 4 1 For each set of vectors use the most efficient method to determine if it is linearly independent or not 0 2 1 U i S ii S v1 iii S3 2 2 1 2 22 W 1 1 1 Uz V3 0 G W4 5 100 101 2 1
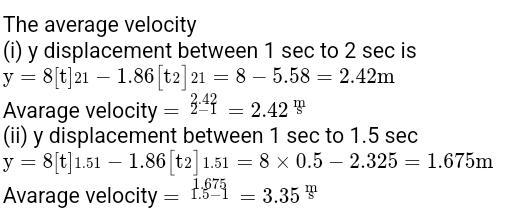
Algebra
Matrices & DeterminantsThe average velocity i y displacement between 1 sec to 2 sec is y 8 t 21 1 86 t2 21 8 5 58 2 42m Avarage velocity 3 42 2 42 ii y displacement between 1 sec to 1 5 sec y 8 t 1 51 1 86 t2 1 51 8 x 0 5 2 325 1 675m Avarage velocity 1 675 3 35
Algebra
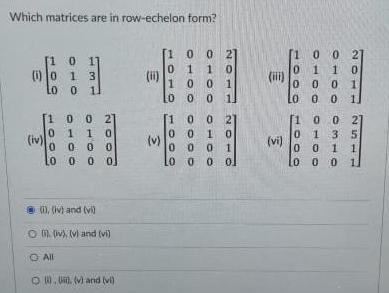
Matrices & DeterminantsWhich matrices are in row echelon form 1 0 11 i 0 1 3 Lo 0 11 iv 131 All 010 0100 N i iv and vi O iv v and vi O U v and vi ii 00 OHOO 0000 0100 0010 NOTO NDIO 10 1 HF vi 10 0010 00000310 21002 251 0 0 0 0 11

Algebra
Matrices & Determinants1 point If the linear system has infinitely many solutions then k and h 7x 7y 4z 3 3x 7y 6x 4 13a 7y hz k
Algebra
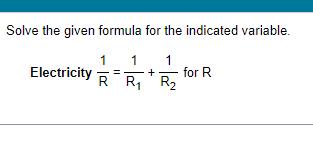
Matrices & DeterminantsSolve the given formula for the indicated variable Electricity RR for R 1 1 1 R
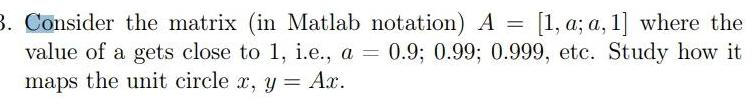
Algebra
Matrices & Determinants3 Consider the matrix in Matlab notation A 1 a a 1 where the value of a gets close to 1 i e a 0 9 0 99 0 999 etc Study how it maps the unit circle x y Ax
Algebra
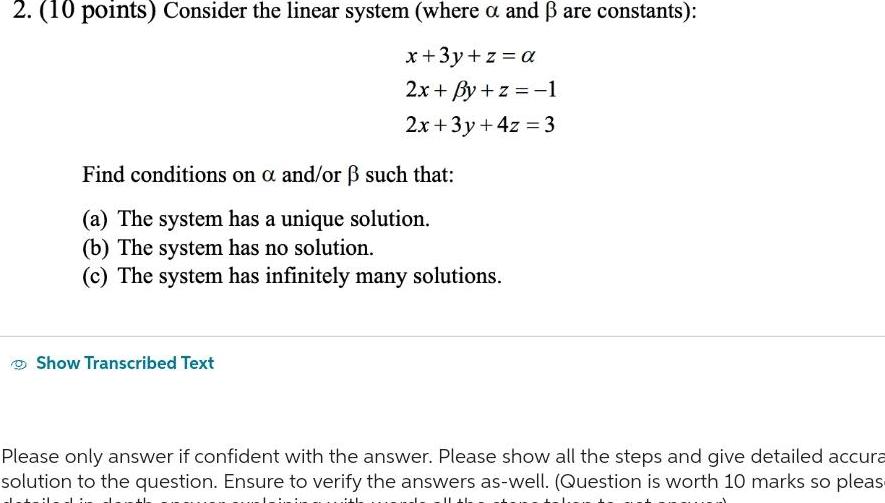
Matrices & Determinants2 10 points Consider the linear system where a and are constants x 3y z a 2x By z 1 2x 3y 4z 3 Find conditions on a and or such that a The system has a unique solution b The system has no solution c The system has infinitely many solutions Show Transcribed Text Please only answer if confident with the answer Please show all the steps and give detailed accura solution to the question Ensure to verify the answers as well Question is worth 10 marks so pleas
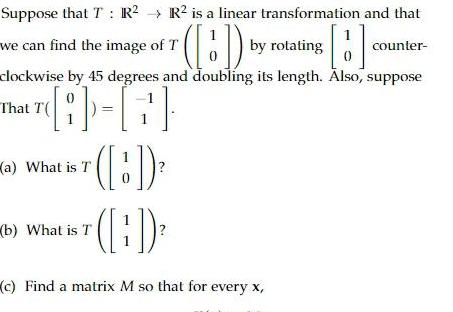
Algebra
Matrices & DeterminantsR is a linear transformation and that BD by rotating B counter clockwise by 45 degrees and doubling its length Also suppose That T T Suppose that T R we can find the image of T a What is T b What is T 1 1 c Find a matrix M so that for every x

Algebra
Matrices & Determinants23 How much water should be added to 50 ml of 80 percent acid solution to dilute it to a 70 percent acid solution ml 24 How many ml of 40 percent acid should be added to pure acid to make 40 ml of 45 percent acid Write your answer as a decimal rounded to the nearest thousandth if necessa x ml 25 An investment firm invested 3000 into two accounts 2200 went to an 12 annual rate account The rest went to a 7 annual rate account The interest the first year amounted to YOU

Algebra
Matrices & Determinants20 The Drama Club receives 1259 25 by selling 341 tickets to the opening night of the musical If the full price of a ticket is 5 25 and discount tickets for students and seniors are 0 75 each how many full price tickets were sold 21 Given two circles J and K with radii 5 in and 8 in respectively the ratio of the areas is O O both of these choices are equi ont

Algebra
Matrices & Determinants22 Given two similar triangles AABC and ADEF if AB 11 and DE 16 and AC 15 then what is the length of side DF Round to the nearest tenth O 11 7 O 21 8 O 21 3 O 10 3 23 How many ml of 10 percent acid should be added to pure acid to make 50 ml of 35 percent acid Write your answer as a decimal rounded to the nearest thousandth if necessary ml