Calculus Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.
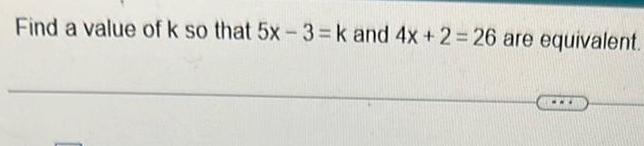

Calculus
Indefinite IntegrationOn summer days on Mars close to its equator the temperature can be around 55 Celsius What is this temperature in degree Fahrenheit 55 C F It

Calculus
Limits & Continuity3 2 Sketch a function that meets all of these conditions a b lim f x 2 814 C lim f x 4 X1 8 e f lim f x co 8 X 3 d lim f x 2 X 3 f 3 5 lim f x 7 X 0 g f 0 1 9 8
Calculus
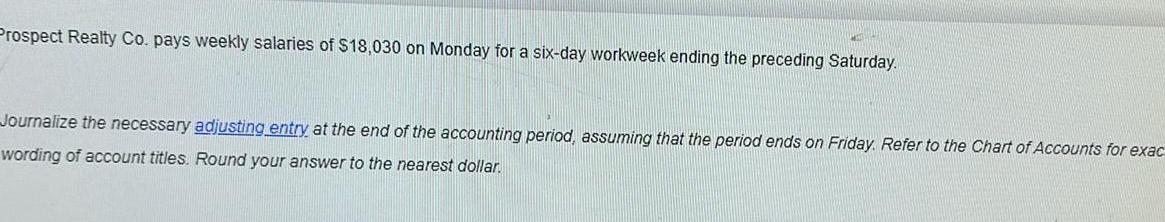
Application of derivativesProspect Realty Co pays weekly salaries of 18 030 on Monday for a six day workweek ending the preceding Saturday Journalize the necessary adjusting entry at the end of the accounting period assuming that the period ends on Friday Refer to the Chart of Accounts for exac wording of account titles Round your answer to the nearest dollar

Calculus
Limits & ContinuityOn June 1 20Y2 Herbal Co received 25 830 for the rent of land for 12 months Journalize the adjusting entry required for unearned rent on December 31 20Y2 Round your answers to the nearest dollar amount If an am does not require an entry leave it blank Dec 31 Unearned Rent

Calculus
Vector CalculusLet S x represent the total sales in thousands of dollars for the month x in the year 2005 at a certain department store Represent each statement to the right by an equation involving S or S a Choose the option with equations that best represent a above OA S 7 136 020 S 7 1 7 OC S 7 1 7 S 7 136 020 a The sales at the end of July reached 136 020 and were rising at the rate of 1700 per month b At the end of December the sales for this month dropped to 60 000 and were falling by about 6 day Use 1 month 30 days OB S 7 1 7 S 7 136 020 OD S 7 136 020 S 7 1 7

Calculus
Limits & ContinuityIn the figure the straight line is tangent to the graph of f x Find f 2 and f 2 f 2 Type an integer or a fraction Simplify your answer 8 0 2 2 y f x

Calculus
DifferentiationDifferentiate the function in two ways f x 2x 2x 3 a Use the general power rule b Multiply 2x2 2x 3 by itself and then differentiate the resulting polynomial a Rewrite f x using the general power rule 0 1 f x

Calculus
Limits & ContinuityAt the end of the holiday season in January the sales at a department store are expected to fall It is estimated that for the x day of January the sales will be 16 S x 3 thousand dollars x 1 a Find the total sales for January 9 and determine the rate at which sales are falling on that day b Compare the rate of change of sales on January 3 to the rate on January 9 What can you infer about the rate of change of sales a The total sales for January 9 were thousand dollars Sales y 4 Days in January

Calculus
Application of derivativesA stone is dropped into a lake creating a circular ripple that travels outward at a speed of 40 cm s a Express the radius r of this circle as a function of the time t in seconds r t b If A is the area of this circle as a function of the radius find A o r Aor t Interpret the answer found in part b O This formula gives the radius of the rippled area in cm at any time t This formula gives the circumference of the rippled area in cm at any time t O This formula gives the extent of the rippled area in cm at any time t

Calculus
Definite Integralsnd each of the following unctions f x 3 x g x x 1 a f g State the domain of the function Enter your answer using interval notation b f g State the domain of the function Enter your answer using interval notation c fg State the domain of the function Enter your answer using interval notation d f g State the domain of the function Enter your answer using interval notation

Calculus
Application of derivativesThe tangent line to the curve y x 2x 5x 3 is parallel to the line 10x 5y 2 at two points on the curve Find the two points The two points are Type an ordered pair Use a comma to separate answers as needed

Calculus
DifferentiationSuppose f 5 8 f 5 4 g 5 5 and g 5 6 Find h 5 and h 5 where h x 5f x 2g x h 5 Simplify your answer


Calculus
Application of derivativesScotland the price of gasoline averages around 1 55 per liter sing unit analysis calculate the cost of gasoline in Scotland in dollars per gallon 1 55 1 L X 1000 mL rag and drop your selection from the following list to complete the answer 1 gal gal 128 fl oz 3 79 L 29 6 mL




Calculus
Limits & ContinuityFind the functions and their domains Enter the domains in interval notation x 5 x 2 f x x a fog fog x domain b gof X gof x domain fof fof x domain d gog gog x domain g x

Calculus
Vector CalculusSet up the problem below and calculate the length of the dime in centime 0 0185 m x 100 m 1 85 Drag and drop your selection from the following list to complete the ans 100 cm 1 cm cm 1 m

Calculus
Differentiatione number 2 511863 rounded to 2 significant figures is e number 553 7165 rounded to 4 significant figures is e number 25 58464 rounded to 3 significant figures is Submit Answer Retry Entire Group 9 more group atter

Calculus
Vector Calculuserform each of the following calculations 1 72 x 10 1 00 10 8 X 3 62 x 10 4 3 72 x 10 4 5 30 10 5 8 72 10 x 1 00 10 6 53 104 1

Calculus
Differentiationften necessary to do calculations using scientific notation when working chemistry proble rm each of the following calculations x 105 2 31 x 10 x 10 5 9 48 x 105 3 36 x 104 7 00 10 5 X 05 2 3 18 x 105 mit Answer 10 2 31 10 Retry Entire Group 9 more group attempts remaining

Calculus
Vector Calculusmany hours are there in exactly 26 d hours answer has bmit Answer significant figures Retry Entire Group

Calculus
Differentiationthermometer reads 32 1 C Calculate this temperature in Fahrenheit degr ahrenheit temperature Submit Answer Retry Entire Group F 9 more group attempts remaining

Calculus
Differentiation63 43 0 08852 177 24 0 823958 Submit Answer Retry Entire Group 8 more e proup attempts remaining

Calculus
Vector Calculusby of your chemistry textbook is found to have a mass of 2360 grams gunit analysis show what the mass of this copy of your chemistry textbook is in gx 2 20 lb and drop your selection from the following list to complete the answer 454 g 237 g OZ 12 oz 28 3 g

Calculus
Limits & ContinuityHow many significant figures are there in each of the following numbers 63 43 0 08852 177 24 0 823958

Calculus
Limits & ContinuityThe boiling point of a liquid is calculated to be 311 K What is this temperature on the Celsius and Fahrenheit scales F

Calculus
Application of derivativesWrite each of the following numbers to 3 significant figures in exponential or scientific notation Write each number with only one non zero digit before the decimal point 254 0 00711 74500 0 00221

Calculus
Vector CalculusThe boiling point of a liquid is calculated to be 334 K What is this temperature on the Celsius and Fahre C F


Calculus
Application of derivativesHow many significant figures are there in each of the following numbers 57 30 0 07084 0 9923 821 65

Calculus
Application of derivativesConsider the following x y 3 y 4 2 2 2 4 6 8 Use the Vertical Line Test to determine whether y is a function of x Yes y is a function of x No y is not a function of x X Solve for y to describe how you can use a graphing utility to produce the given graph

Calculus
DifferentiationDetermine the open intervals on which the function is increasing decreasing or constant Enter your answers using interv notation If an answer does not exist enter DNE f x x 2x increasing decreasing constant f x 0 f x 0 DNE 2 1 X X y 8 9 4 2 1 2 3 4 X
Calculus
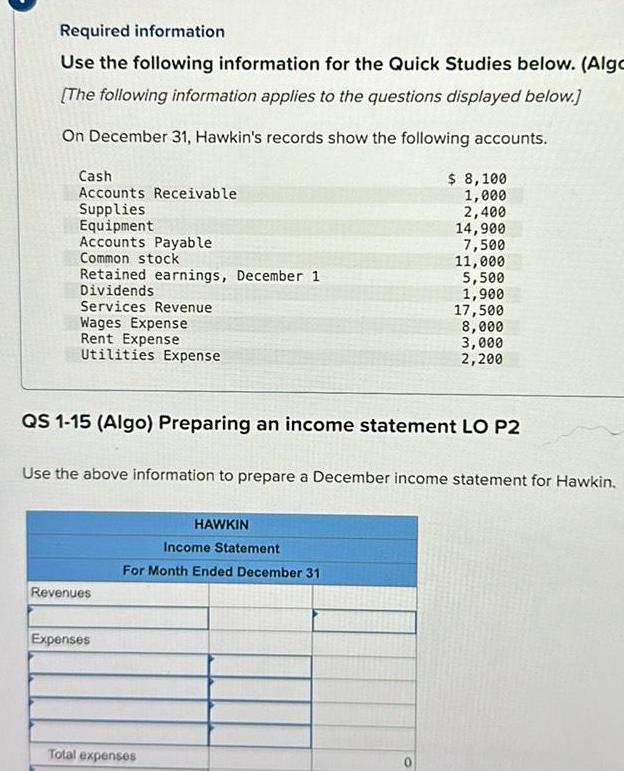
Application of derivativesRequired information Use the following information for the Quick Studies below Algo The following information applies to the questions displayed below On December 31 Hawkin s records show the following accounts Cash Accounts Receivable Supplies Equipment Accounts Payable Common stock Retained earnings December 1 Dividends Services Revenue Wages Expense Rent Expense Utilities Expense QS 1 15 Algo Preparing an income statement LO P2 Revenues Use the above information to prepare a December income statement for Hawkin Expenses 8 100 1 000 2 400 14 900 HAWKIN Income Statement For Month Ended December 31 Total expenses 7 500 11 000 5 500 1 900 17 500 8 000 3 000 2 200

Calculus
Definite IntegralsFind if is between 0 and 90 Round your answer to the nearest tenth of a degree 63 cos 80 9770 66 cos 0 5490 69 sin 0 7038 64 sin 0 3971 67 sin 0 9813 70 cos 0 0 9153 65 tan 0 6873 68 tan 8 0 6273 71 cos 0 0 4112

Calculus
Application of derivativesUse a calculator to find each of the following Round all answers to four places past the decimal point 33 sin 27 2 36 sin 42 34 cos 82 9 37 tan 87 32 35 cos 18 38 tan 81 43

Calculus
Definite IntegralsFor the following exercises split the region between the two curves into two smaller regions then determine the area by integrating over the x axis Note that you will have two integrals to solve 3 y x and y x x 2 1 YA 5 4 3 2 1 1 g x x x f x x 1 2X

Calculus
DifferentiationMaintenance money for an athletic complex has been sought Mr Kendall the Athletic Director would like to solicit donation to cover all future expected maintenance costs for the building These maintenance costs are expected to 1 5 million each year for the first five years 1 8 million each year for years 6 through 10 and 2 million each yea after that The building has an indefinite service life If the money is placed in an account that will pay 8 interest compounded annually how large should the gift be Click the icon to view the interest factors for discrete compounding when i 8 per year The gift should be S million Round to two decimal plagos

Calculus
Application of derivativesConvert each of the following to degrees and minutes 15 35 4 16 63 2 17 16 25 18 18 75

Calculus
Application of derivativesAdd and subtract as indica 1 37 45 26 24 3 51 55 37 45 5 61 33 45 16 7 90 34 12 9 180 120 17

Calculus
DifferentiationAdd and subtract as indicated 1 37 45 26 24 3 51 55 37 45 5 61 33 45 16 7 90 34 12 9 180 120 17 11 76 24 13 70 40 22 34 30 50

Calculus
Limits & ContinuityUse a calculator to find an approximation to each of the following expressions Round to four decimal places 51 sin 60 53 V3 55 sin 45 57 1 2 52 cos 30 54 V 56 cos 45 58 3

Calculus
Application of derivativesFind exact values for each of the following 43 sec 30 45 csc 60 47 cot 45 49 sec 45 44 csc 30 46 sec 60 48 cot 30 50 csc 45

Calculus
Vector CalculusFind exact values for each of the following 43 sec 30 45 csc 60 44 csc 30 46 sec 60
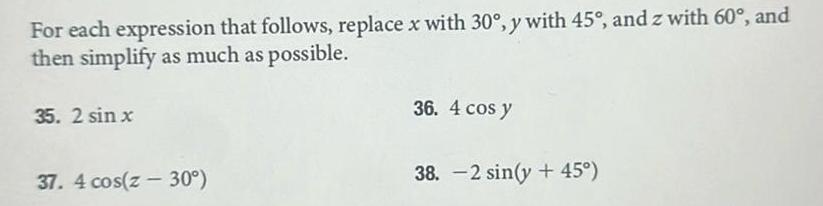
Calculus
Application of derivativesFor each expression that follows replace x with 30 y with 45 and z with 60 and then simplify as much as possible 35 2 sin x 37 4 cos z 30 36 4 cos y 38 2 sin y 45

Calculus
Application of derivativesSimplify each expression by first substituting values from the table of exact value and then simplifying the resulting expression 23 4 sin 30 25 2 cos 30 24 5 sin 30 26 sin 30
Calculus
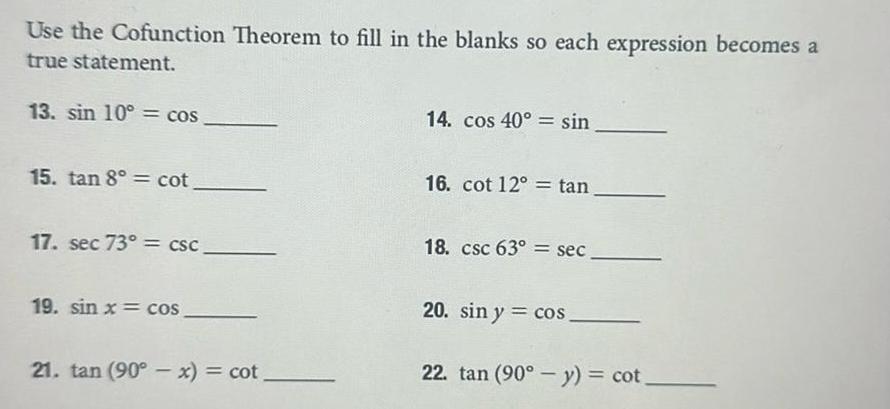
Application of derivativesUse the Cofunction Theorem to fill in the blanks so each expression becomes a true statement 13 sin 10 cos 15 tan 8 cot 17 sec 73 csc 19 sin x cos 21 tan 90 x cot 14 cos 40 sin 16 cot 12 tan 18 csc 63 sec 20 sin y cos 22 tan 90 y cot

Calculus
Application of derivativesUse the Cofunction Theorem to fill in the blanks so each expression becomes a true statement 13 sin 10 cos 14 cos 40 sin