Straight lines Questions and Answers

Math
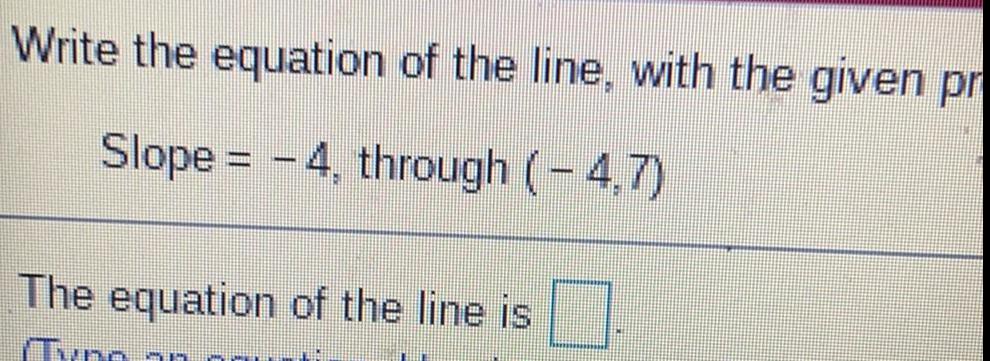
Straight linesGraph this line using the slope and y-inter
y = 8x - 7
Click to select points on the graph.

Math
Straight linesYou work in an electronics store. You earn a fixed amount of $35 per day, plus a 15% bonus on the merchandise you sell. Write an equation that models the amount y (in dollars) you earn for selling x dollars of merchandise in one day. Graph the equation.
Math
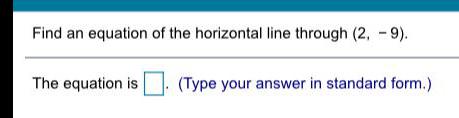
Straight linesFind an equation of the horizontal line through (2, -9).
The equation is
(Type your answer in standard form.)

Math
Straight linesFind an equation perpendicular to y = 1 and passing through (6,-1).
The equation is (Type your answer in standard form.)
Math
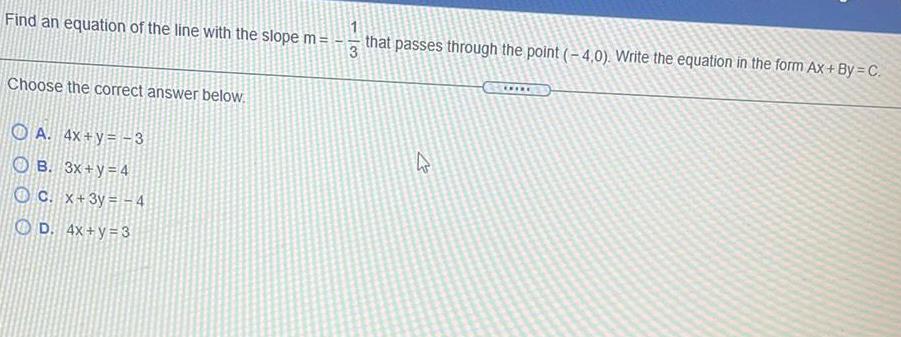
Straight linesFind an equation of the line with the slope m = 1/3 that passes through the point (-4,0). Write the equation in the form Ax+By=C.
Choose the correct answer below.
A. 4x+y=-3
B. 3x+y=4
C. x+3y=-4
D. 4x+y=3
Math
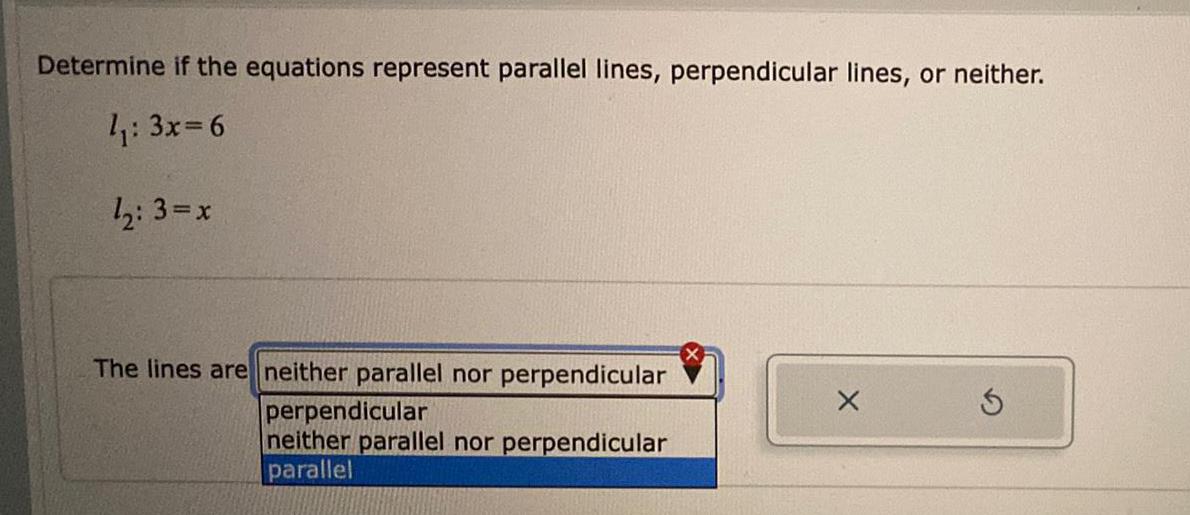
Straight linesDetermine if the equations represent parallel lines, perpendicular lines, or neither.
1₁:3x=6
1₂:3=x
The lines are neither parallel nor perpendicular
perpendicular
neither parallel nor perpendicular
parallel
X

Math
Straight linesWrite an equation of the line with the given slope, m, and y-intercept (0,b).
m = -3, b = -1/5

Math
Straight linesGraph using the x- and y-intercepts.
y = 3x +3
Use the graphing tool to graph the linear equation. Use the intercepts when
drawing the line. If only one intercept exists, use it and another point to draw the
line.


Math
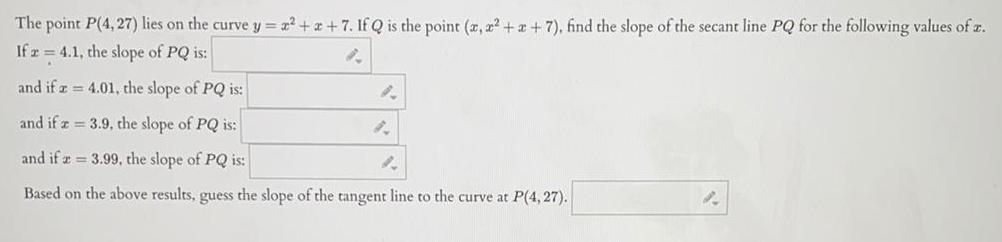
Straight linesThe point P(4, 27) lies on the curve y=x²+x+7. If Q is the point (x, x² + x + 7), find the slope of the secant line PQ for the following values of z.
If z = 4.1, the slope of PQ is:
and if = 4.01, the slope of PQ is:
and if z = 3.9, the slope of PQ is:
and if r = 3.99, the slope of PQ is:
Based on the above results, guess the slope of the tangent line to the curve at P(4, 27).
Math
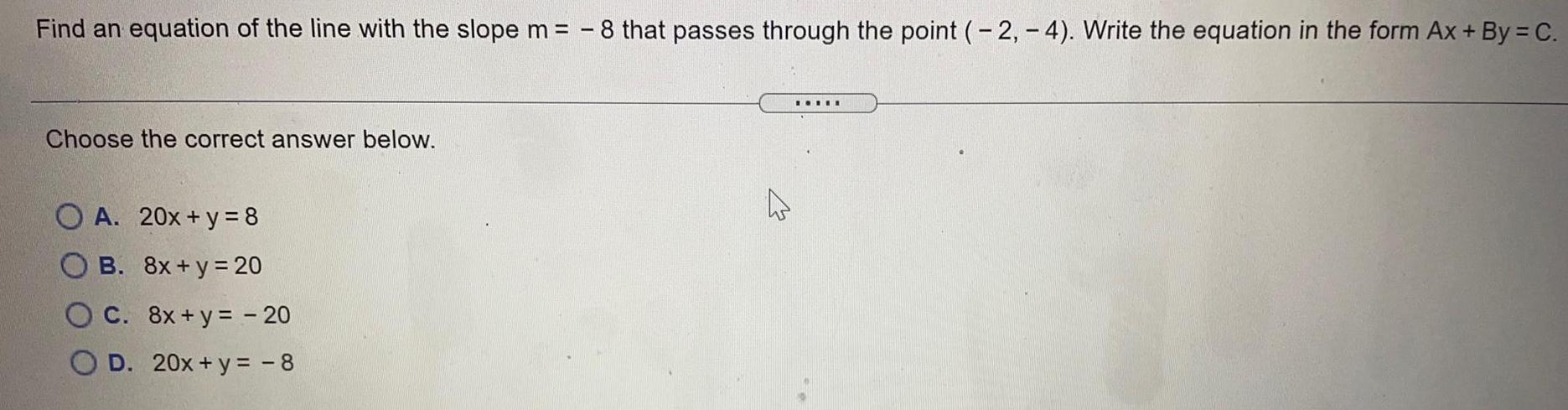
Straight linesFind an equation of the line with the slope m = - 8 that passes through the point (-2,-4). Write the equation in the form Ax+By = C.
Choose the correct answer below.
A. 20x + y = 8
B. 8x+y=20
C. 8x + y = -20
D. 20x + y = -8.

Math
Straight linesFind an equation of the line through the pair of points. Write the equation in the form Ax+By = C.
(-7,3) and (8,-5)
A. - 10x + 13y = 15
B. 8x - 15y = 11
c. 10x-13y = 15
D.-8x-15y = 11
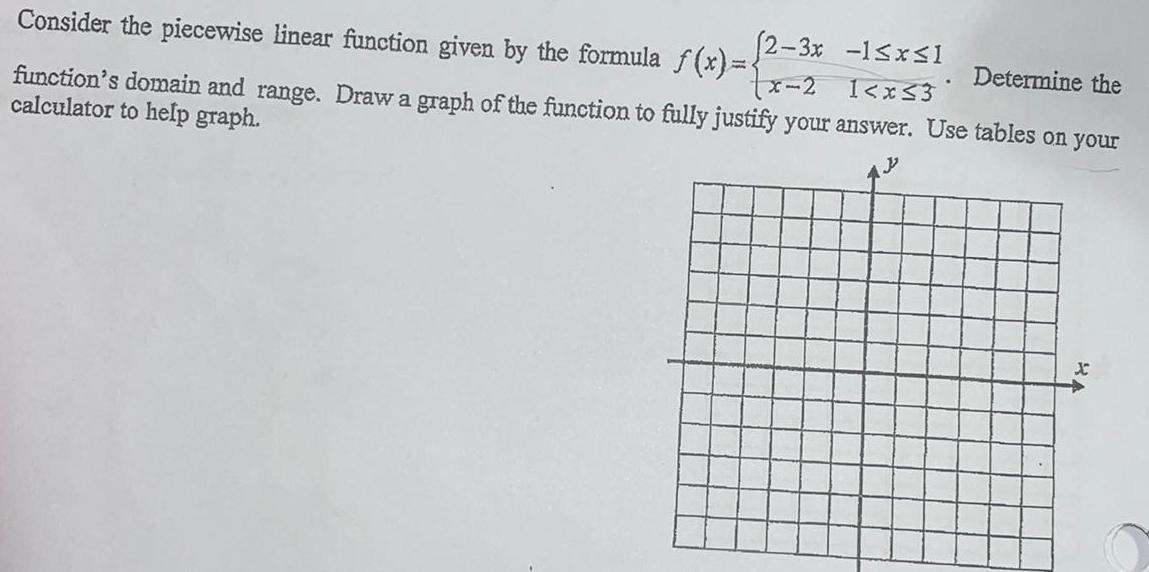
Math
Straight linesConsider the piecewise linear function given by the formula f(x)=(2-3x
tx-2
-1≤x≤l
1<x<3
.
Determine the
function's domain and range. Draw a graph of the function to fully justify your answer. Use tables on your
calculator to help graph.
Math
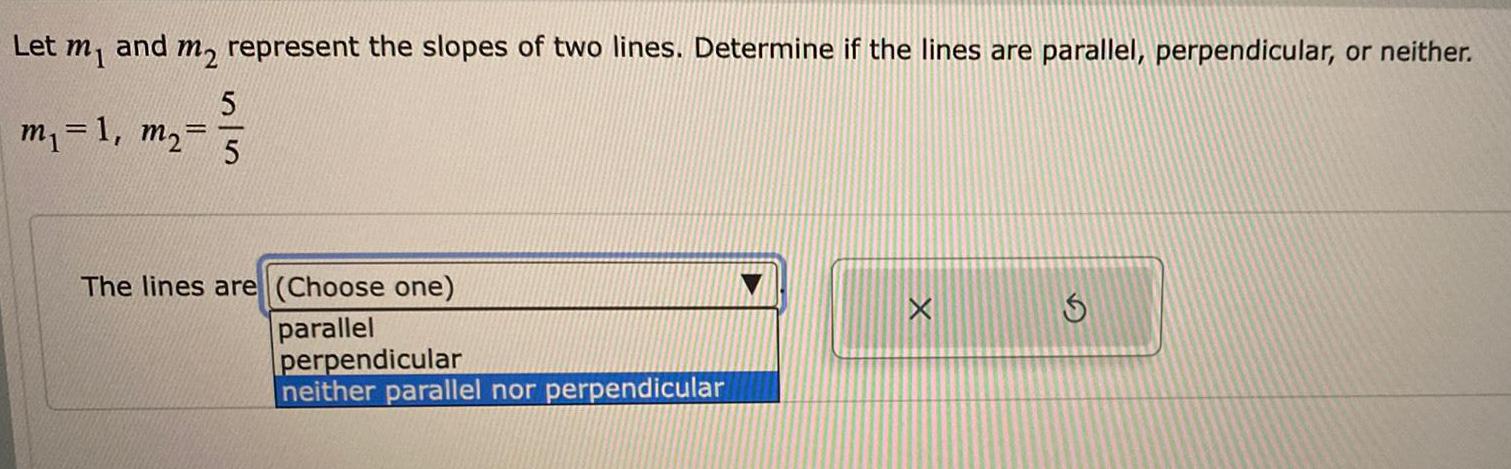
Straight linesLet m₁ and m2 represent the slopes of two lines. Determine if the lines are parallel, perpendicular, or neither.
m₁ = 1, m₂= 5/5
The lines are (Choose one)parallel perpendicular/neither parallel nor perpendicular

Math
Straight linesConsider the line with the equation: y = -4x-17
Give the equation of the line parallel to Line 1 which passes through (10, 4) :
Give the equation of the line perpendicular to Line 1 which passes through (10, 4):

Math
Straight linesDraw a line representing the "rise" and a line representing the "run" of the line. State the slope of the line in simplest form.
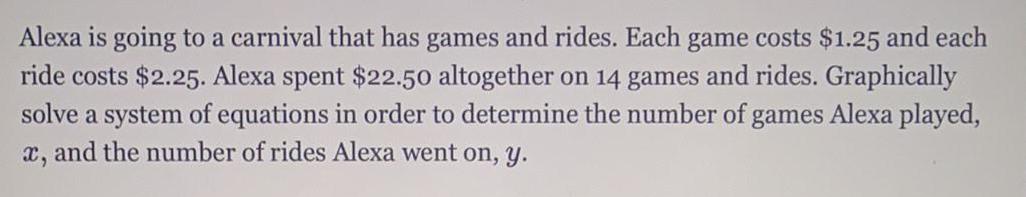
Math
Straight linesAlexa is going to a carnival that has games and rides. Each game costs $1.25 and each ride costs $2.25. Alexa spent $22.50 altogether on 14 games and rides. Graphically solve a system of equations in order to determine the number of games Alexa played, x, and the number of rides Alexa went on, y.
Math
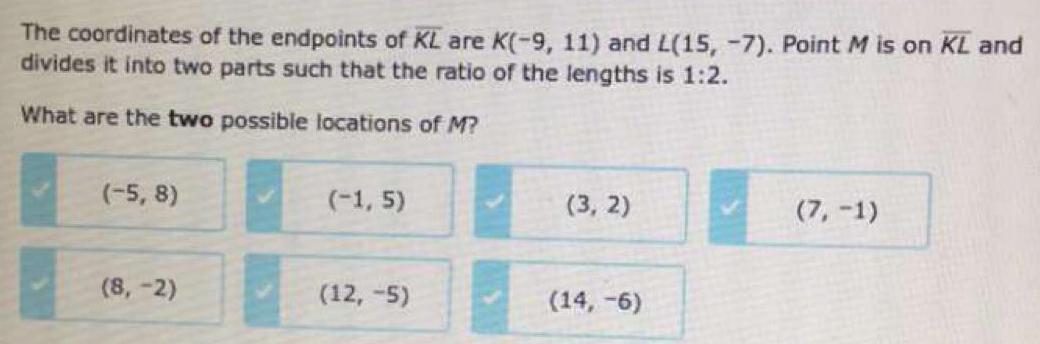
Straight linesThe coordinates of the endpoints of KL are K(-9, 11) and L(15, -7). Point M is on KL and
divides it into two parts such that the ratio of the lengths is 1:2.
What are the two possible locations of M?
(-5, 8)
(8,-2)
(-1, 5)
(12,-5)
(3, 2)
(14, -6)
(7, -1)

Math
Straight linesFrom the multiple choice, choose ALL that are true about vertical angles.
They can be adjacent
They can form a linear pair
They always add to 180
They are always congruent
They always add to 90
Math
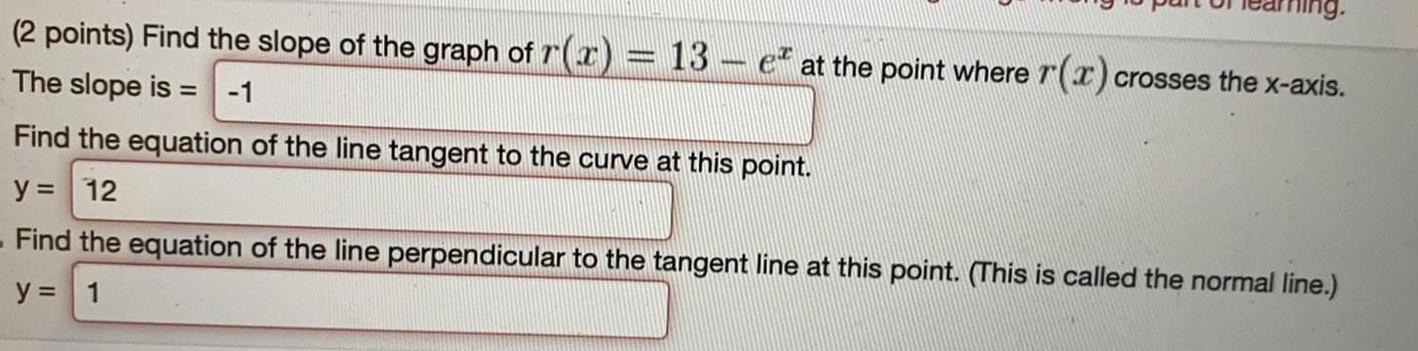
Straight linesFind the slope of the graph of 7(x) = 13 – eª at the point where r(x) crosses the x-axis.
The slope is =
Find the equation of the line tangent to the curve at this point.
y =
Find the equation of the line perpendicular to the tangent line at this point. (This is called the normal line.)
y =

Math
Straight linesFor a and b, write an equation in slope-intercept form that meets the given criteria.
a. A negative slope and passes through the origin
b. Slopes upward from left to right and has a y-intercept below the x-axis.
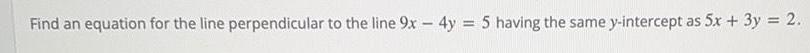
Math
Straight linesFind an equation for the line perpendicular to the line 9x - 4y = 5 having the same y-intercept as 5x + 3y = 2.

Math
Straight linesA line has this equation: y=-x-2
Write an equation for the parallel line that goes through (3, 7).

Math
Straight linesAn ostrich can run at a speed of 45 miles per hour.
a) Copy and complete the following table to show the distances an ostrich can run in various times.
Time in hours 0 1 2 3 4
Distance in miles 0
b) How does the distance vary with respect to time?
c) Write a formula for this function, letting d represent the distance and t represent the time.
Math
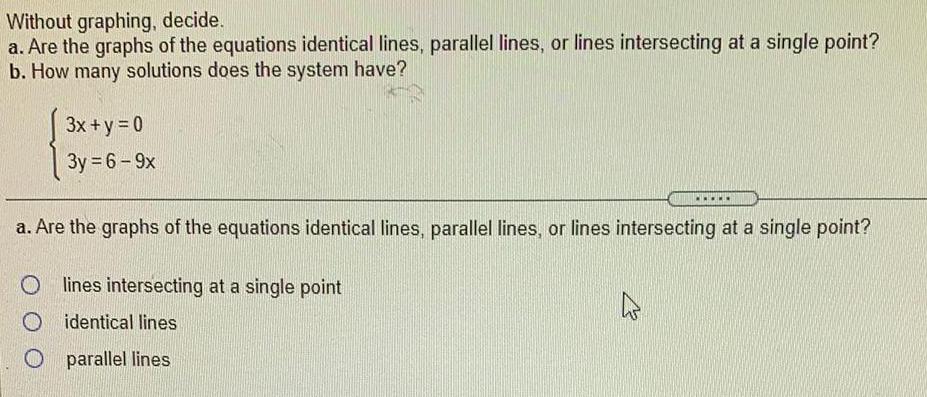
Straight linesWithout graphing, decide.
a. Are the graphs of the equations identical lines, parallel lines, or lines intersecting at a single point?
b. How many solutions does the system have?
3x + y = 0
3y = 6 - 9x
a. Are the graphs of the equations identical lines, parallel lines, or lines intersecting at a single point?
lines intersecting at a single point
identical lines
parallel lines

Math
Straight linesYour above-ground pool has sprung a leak, and you do not have a way to fix it. At 12 noon, the water in the pool is 5 feet deep (in other words, the water level is 5 feet above the ground). You are not sure how quickly the pool level is falling, so you check again after 40 minutes and see that the height of the water level is now 4 feet. You notice that there is a relationship between the time that has passed to the height of the water level in the pool.
(a) If you assume the height of the water level is decreasing at a constant rate, what will be the height of the water level at 1 p.m.?
(b) Write a linear function, h, that relates the time past noon, t, in minutes, and the height of the water level in the pool, h(t), in feet.
(c) Explain the meaning of the rate of change of function h.
(d) Evaluate h(75), and explain its meaning in the context of time passed and height of the water level.
(e) At what time, t, will h(t)=0.5? What does your solution mean in the context of the problem?
(f) When you check the pool at 3 p.m., the height of the water level is approximately 1 foot, 4 inches. What conclusions can you draw?

Math
Straight linesConsider the line y=7/9x+6.
Find the equation of the line that is perpendicular to this line and passes through the point (-7, -2).
Find the equation of the line that is parallel to this line and passes through the point (-7, -2).
Math
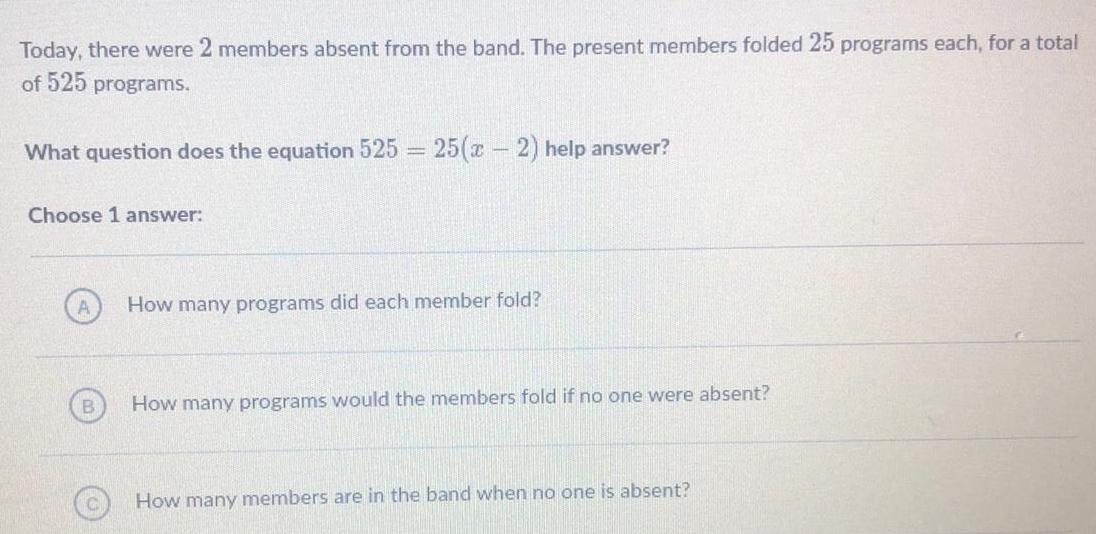
Straight linesToday, there were 2 members absent from the band. The present members folded 25 programs each, for a total of 525 programs.
What question does the equation 525 = 25(x - 2) help answer?
Choose 1 answer:
A How many programs did each member fold?
B How many programs would the members fold if no one were absent?
C How many members are in the band when no one is absent?

Math
Straight linesAt a particular restaurant, each mini hotdog has 100 calories and each chicken wing has 50 calories. A combination meal with chicken wings and mini hotdogs is shown to have 600 total calories and twice as many chicken wings as there are mini hotdogs. Graphically solve a system of equations in order to determine the number of mini hotdogs in the combination meal, x, and the number of chicken wings in the combination meal, y.

Math
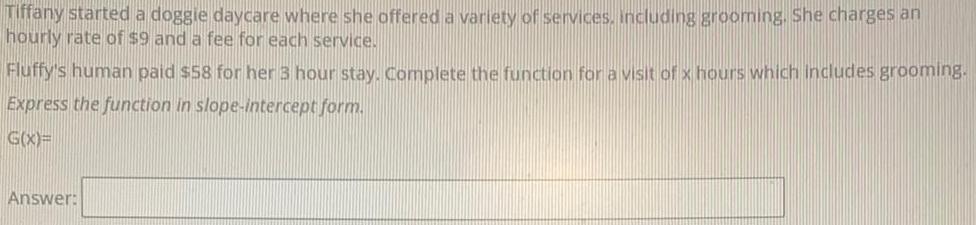
Straight linesTiffany started a doggie daycare where she offered a variety of services, including grooming. She charges an
hourly rate of $9 and a fee for each service.
Fluffy's human paid $58 for her 3 hour stay. Complete the function for a visit of x hours which includes grooming.
Express the function in slope-intercept form.
G(X)=
Answer:

Math
Straight linesA dilation centered at the origin is applied to the line y = 3x + 5.
What is true about the image of the line?
O It is the same line.
O It is another line parallel to y = 3x + 5.
O The image is not a line.
O The question cannot be answered without knowing the scale factor.

Math
Straight linesGabriella expects to earn $50 in tips during her shift as a waitress today. In addition to tips, she earns $8.00 an hour. If she wants to make at least $114 today, how many hours must she work at a minimum?
Math
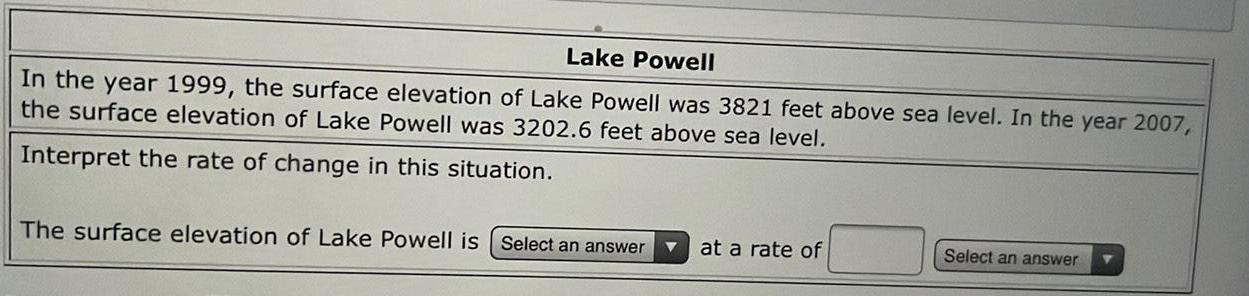
Straight linesIn the year 1999, the surface elevation of Lake Powell was 3821 feet above sea level. In the year 2007, the surface elevation of Lake Powell was 3202.6 feet above sea level.
Interpret the rate of change in this situation.
The surface elevation of Lake Powell is ... at a rate of ...

Math
Straight linesAn airplane begins a flight with a total of 39.3 gal of fuel stored in two separate wing tanks. During the flight, 25.3% of the fuel in one tank is used, and in the other tank 34.2% of the fuel is used. If the total fuel used is 12.1 gal, the amounts x and y available in each tank before flight can be found by solving the system of equations which is below.
x+y=39.3
0.253x+0.342y = 12.1
Find x and y.

Math
Straight linesA company that packages cold cuts needs to transport the packaged meat to the supermarket. It will cost $1,200 to rent a truck, and it will cost an additional $100 for every 50 pounds transported. Which equation represents the transport cost for every 50 pounds of cold cuts transported?
A y=1,200 - 100x
B. y=1,200 + 100x
C. y=1,200x+100
D. y=100x-1,200

Math
Straight linesA new car was purchased for $14,600, and 4 years later it was worth $6,600. Assume that the depreciation in value is given by a linear equation. Find the value of the car at the end of 5 years
A. $12,600
B. $4,600
C. $8,000
D. $20,600

Math
Straight linesThe intersection of the planes 3x+2y+5z = 22 and 2x+y+3z = 13 is a line, one of whose points is (1, 2, 3). Find another point on this line. Once you have found a second point, write the parametric description of all the points on the line.

Math
Straight linesAnumeha is mowing lawns for a summer job. For every mowing job, she charges an initial fee of $10 plus a constant fee for each hour of work. Her fee for a 5-hour job, for instance, is $35. Let y represent Anumeha's fee (in dollars) for a single job that took a hours for her to complete.

Math
Straight linesWrite an equation of the line containing the given point and parallel to the given line. Express your answer in the form y=mx+b.
(-4,-2); 2x-9y=5

Math
Straight linesA taxi driver buys a new car for $50,000. The car will have a scrap value of $10,000 after 8 years. Using the straight-line depreciation method, find the value of the car after 4 years.
(A) $10,000 (B) $13,000 (C) $15,000 (D) $25,000 (E) $30,000

Math
Straight linesFind the slope-intercept form of the equation of the line satisfying the given conditions. Do not use a calculator.
Through (3,12) and (0,3)
The slope-intercept form of the line is y=
(Simplify your answer. Use integers or fractions for any numbers in the equation. Do not factor.)
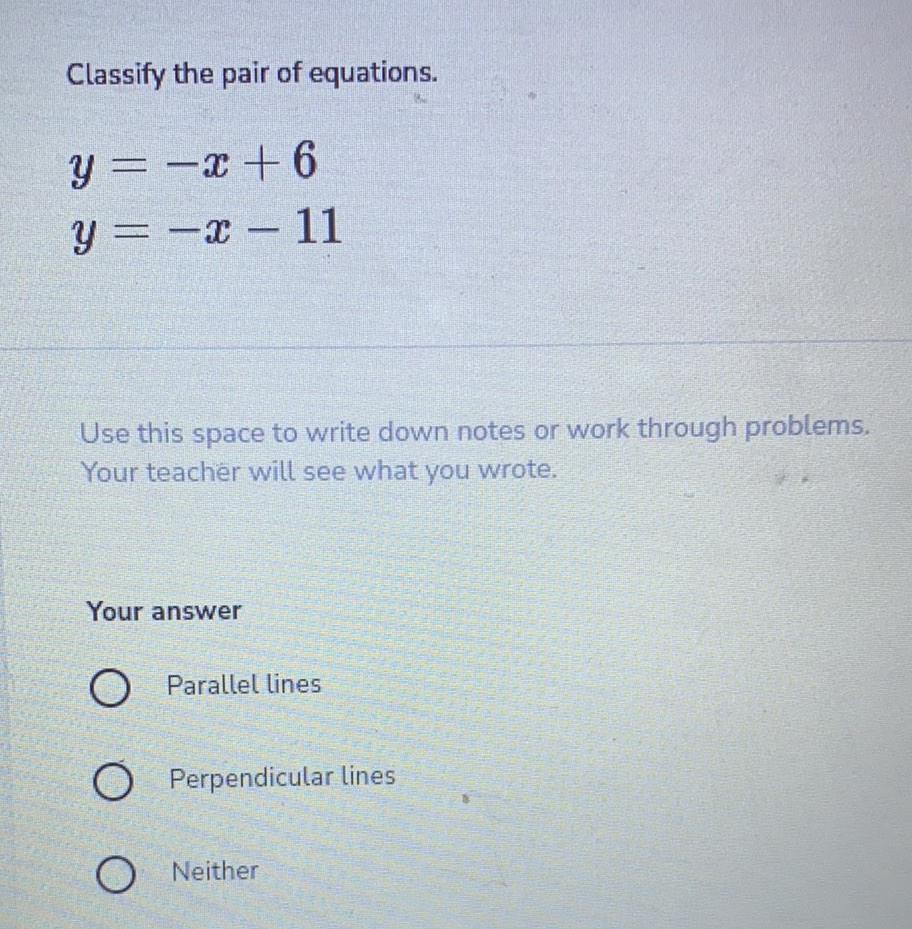
Math
Straight linesClassify the pair of equations.
y=-x+6
y=-x-11
Use this space to write down notes or work through problems.
Your teacher will see what you wrote.
Your answer
Parallel lines
Perpendicular lines
Neither

Math
Straight linesA linear equation is given in standard form: 4x + 5y = 20.
Find the x-intercept:
Find the y-intercept:
Rewrite the given equation in slope intercept form:

Math
Straight linesFind three integer or decimal solutions to the following equation, and then graph the solution set.
y = 3x - 2
(_________), (__________), (____,____),

Math
Straight linesGive the equation of the line passing through the points (-3/11,2/3),(-3/11,7).
The equation of the line is:

Math
Straight linesThe equation of the line that goes through the point (6, 4) and is parallel to the line 4x + 3y = 2 can be written in the form y = mx + b
where m is:
and where b is:

Math
Straight linesGive the slope and the y intercept of the line y + 6x = 9
Slope =
y intercept =
(just give the y-coordinate)