Probability Questions and Answers

Math
ProbabilityYou roll a six-sided die and select a card from a standard deck of cards. Find the probability that you roll a number less than five on the die and select a face card from the deck of cards. Write your answer as a reduced fraction. (Use the / symbol to write your fraction, ex. 1/2.) Hint (they are
independent events). P(A)*P(B)
The probability is

Math
ProbabilityA box contains 36 orange golf balls, 28 white golf balls, and 92 yellow golf balls. If a golf ball is drawn at random from the box, find the probability that it will NOT be an orange ball. Write your answer as a fraction in simplest form. (Use the /symbol to write your fraction, ex. 1/2) Hint: 1 - P(Orange Ball)
The probability is
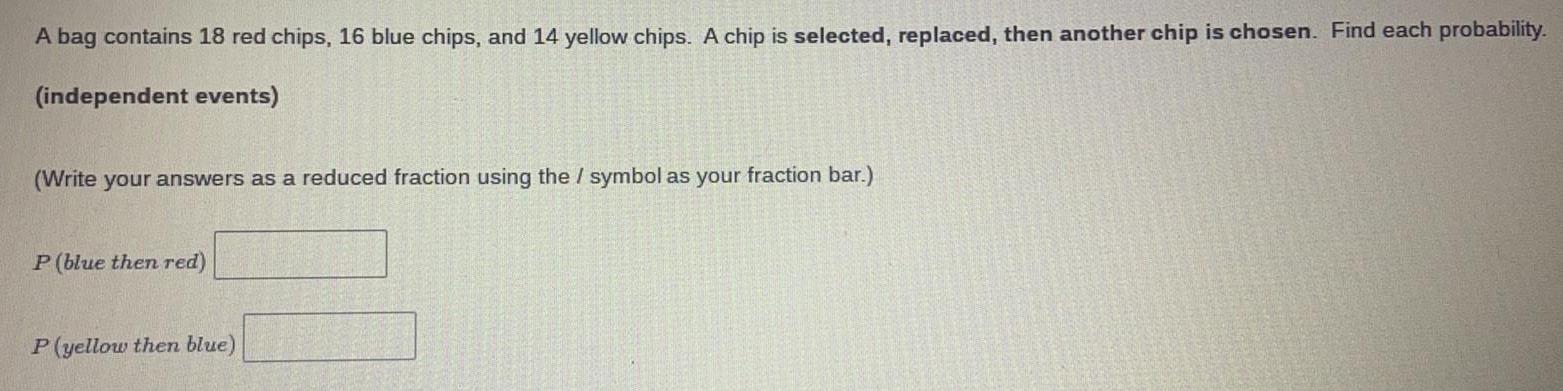
Math
ProbabilityA bag contains 18 red chips, 16 blue chips, and 14 yellow chips. A chip is selected, replaced, then another chip is chosen. Find each probability.
(independent events)
(Write your answers as a reduced fraction using the / symbol as your fraction bar.)
P (blue then red)
P(yellow then blue)

Math
ProbabilityFind the probability that when a couple has 2 children, they will have exactly 1 boy. Assume that boys and girls are equally likely and that the gender of any child is not influenced by the gender of another child.

Math
ProbabilityA box has twelve balls in it, seven white and five red. We select one ball, do not put it back in
the box, and select a second ball, etc. We are not returning any balls to the box. So we are
selecting multiple balls from the box. Find the probability of the following events (each
scenario below is separate and is not dependent upon another):
a. The probability of selecting a white ball on the first draw and selecting a white ball on the
second draw.
b. The probability of selecting a white ball on the first draw and selecting a red ball on the
second draw.
c. The probability of selecting a red ball on the first draw, selecting a white ball on the second
draw and selecting a white ball on the third draw.
30 916 tedt ginays to sing sediazab bna 91691) 81
d. The probability of selecting a red ball on the first draw, selecting a red ball on the second
draw, selecting a white ball on the third draw and selecting a white ball on the fourth draw.
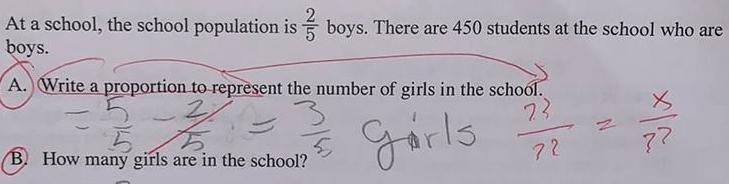
Math
ProbabilityAt a school, the school population is 2/5 boys. There are 450 students at the school who are
boys.
A. Write a proportion to represent the number of girls in the school.
B. How many girls are in the school?

Math
ProbabilityRound your answer to 3 decimal places when necessary.
Find the probability of selecting either a non-smoker or a woman when you select a person at random from a group composed of 50 female smokers, 45 male smokers, 20
female non-smokers, and 45 male non-smokers.
0.125
0.844
0.719
0.178

Math
ProbabilityA spinner is used for which it is equally probable that the pointer will land on any one of twelve regions. Four of the regions are colored green, six are colored yellow, and two are colored red. If the pointer is spun twice, find the probability it will land on green and then red.

Math
ProbabilityFour golfers will be randomly split into 2 groups of 2
for a tournament. If Jill and Ramona are among the 4,
what is the probability that they will be paired
together?
F. 1/12
G.1/8
H. 1/6
J. 1/4
K. 1/3

Math
ProbabilityThere are fourteen songs on your playlist. With random shuffle and no repetition, you listen to two songs. What is the probability that you listened to your favorite song first and your least favorite song second?

Math
ProbabilityRodney's Repair Service has a lug nut tightening machine that works well 86% of the time. They got a new machine that works well 96% of the time. Each machine is used 50% of the time. Use Bayes' Theorem to find the probability. If necessary, round your calculations to the nearest thousandths, and write your answer as a percent. The new machine malfunctioned. The new machine will malfunction % of the time.
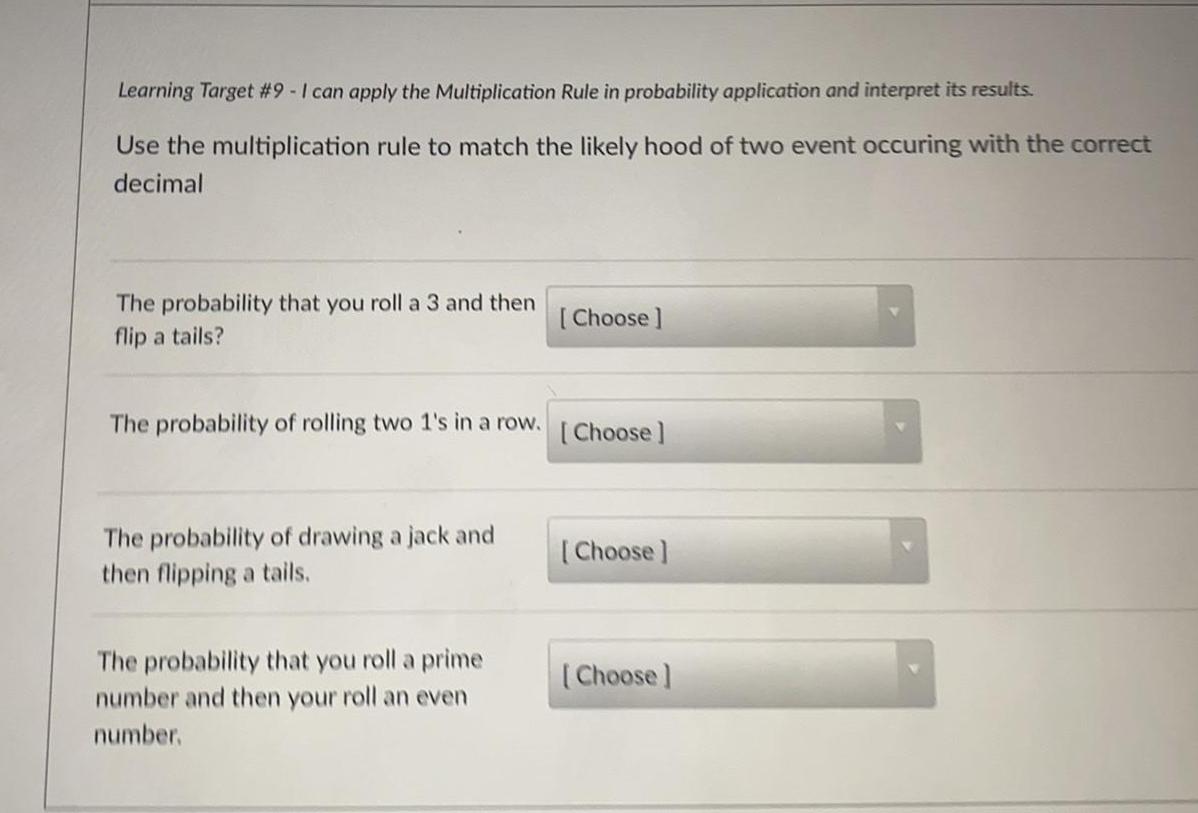
Math
ProbabilityUse the multiplication rule to match the likely hood of two event occuring with the correct decimal
The probability that you roll a 3 and then flip a tails?
The probability of rolling two 1's in a row.
The probability of drawing a jack and then flipping a tails.
The probability that you roll a prime number and then your roll an even number.

Math
ProbabilityI can calculate the probability of independent events using P(A U B) and P(A n B), and
interpret them in context.
Given the following events:
Event A: Drawing a red card
Event B: Drawing an ace
Calculate the probability of drawing a red ace. P (An B)

Math
ProbabilityGiven the two events:
Event A is drawing a queen.
Event B is drawing a red card.
Calculate the probability that you draw a queen or a red card? P(AUB)

Math
ProbabilityChoose the best definition of compound events
Consists of two or more simple events.
None of the other answers are correct
A selection of objects in which the order of the objects matters.
Combining the elements of two or more sets
The product of a given integer and all smaller positive integers

Math
ProbabilityMarcus reads in a magazine article that 62% of teenagers prefer Batman over Iron Man. He decides to test this so he takes a simple random sample of 50 people at Wal-Mart on a Saturday afternoon.
1. What is the probability that he finds exactly 40 Batman fans?
2. What is the probability that he finds fewer than 30 Batman fans?
3. What is the probability that Marcus will find more than 35 Batman fans?

Math
ProbabilityI can calculate the conditional probability of A, given B, and interpret the answer in context. In New England, 84% of the houses have a garage and 65% of the houses have a garage and a back yard. What is the probability that a house has a backyard given that it has a garage? Enter you answer a decimal rounded to the nearest hundredth.

Math
ProbabilityA cooler contains thirteen bottles of sports drink: seven lemon-lime flavored and six orange flavored. You randomly grab a bottle and give it to your friend. Then, you randomly grab a bottle for yourself. You and your friend both get lemon-lime.

Math
ProbabilityIn Europe, 88% of all households have a television. 51% of all households have a television and a VCR. What is the probability that a household has a VCR given that it has a television? Enter you answer a decimal rounded to the nearest hundredth.

Math
ProbabilityA bag contains 4 blue marbles, 6 red marbles, and 8 green marbles. What is the probability that a marble drawn randomly is not blue?

Math
ProbabilityIn the United States, 56% of all children get an allowance and 41% of all children get an allowance and do household chores. What is the probability that a child does household chores given that the child gets an allowance?

Math
ProbabilityJack has a bag of marbles. There are 30 marbles in the bag. 12 red marbles, 8 blue marbles and 10 green marbles. Each red marble is worth 5 point, each blue marble is worth 3 points and each green marble is worth 2 points. What is the approximate expected value of picking a marble from the bag? Round answer to the hundredth. *

Math
ProbabilityEach of 11 pieces of paper contains a different number from (4,6,7,9,10,11,12,13,15,17,19). The pieces of paper are folded and placed in a bag. In selecting a piece of paper without looking, find the probability of selecting an odd number or a number that is at least 12.
1/8
9/11
7/11
8/11
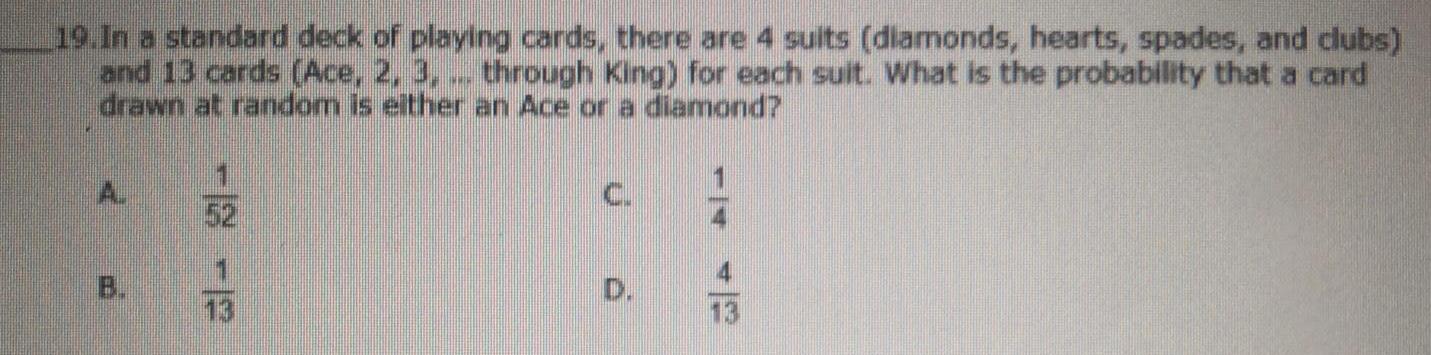
Math
Probability19.In a standard deck of playing cards, there are 4 suits (diamonds, hearts, spades, and clubs) and 13 cards (Ace, 2, 3, ... through King) for each suit. What is the probability that a card drawn at random is either an Ace or a diamond?

Math
ProbabilityLearning Target #8 - Interpret independence of events A and B using conditional probability.
If P(B|A)=P(B) then events A and B are
Independent
Cannot Determine
Dependent

Math
ProbabilityAt a middle school, 18% of all students play football and basketball and 32% of all students play football. What is the probability that a student plays basketball given that the student plays football?

Math
ProbabilityThe probability that a building's front door lock will jam on a random day is 5%, and the probability that the front door lock will jam and the locksmith will be out sick on a random day is 0.4%. Assuming these two events are independent, find the probability that the locksmith will be out sick on a random day.
(A) 2%
(B) 4.6%
(C) 8%
(D) 12.5%
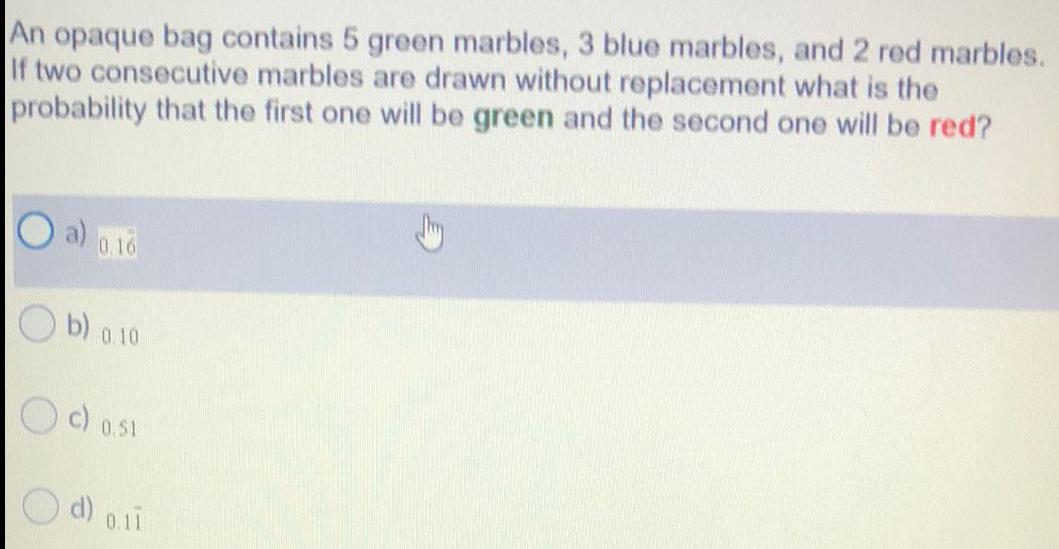
Math
ProbabilityAn opaque bag contains 5 green marbles, 3 blue marbles, and 2 red marbles.
If two consecutive marbles are drawn without replacement what is the
probability that the first one will be green and the second one will be red?
a)0.16
b) 0.10
c) 0.51
d) 0.11

Math
ProbabilityDetermine if the scenario Involves mutually exclusive events. Then find the probability. A box of chocolates contains three milk chocolates, four dark chocolates, and three white chocolates. You randomly select a chocolate. What is the probabil Is a milk chocolate or a dark chocolate?
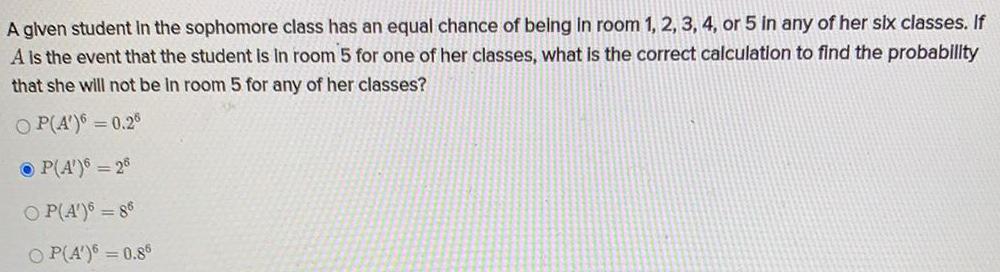
Math
ProbabilityA given student in the sophomore class has an equal chance of being in room 1, 2, 3, 4, or 5 in any of her six classes. If A is the event that the student is in room 5 for one of her classes, what is the correct calculation to find the probability that she will not be in room 5 for any of her classes?
P(A) = 0.28
P(A) = 28
P(A) = 88
P(A')6 = 0.86

Math
ProbabilityThe guidance department has reported that of the senior class, 2.3% are
members of Key Club, K, 8.6% are enrolled in AP Physics, P, and 1.9% are
in both. What is the probability of P given K?
a) 22.1%
b) 13.4%
c) 82.6%
d) 26.7%

Math
ProbabilityOn a particular day, the mean price of gasoline was $3.63 a gallon with a standard deviation of $0.20 a gallon. What Is
the probability that a gas station has a price between $3.43 and $3.63 a gallon? Use the 68-95-99.7 normal distribution
curve.
15.85%
49.85%
13.50%
O 2.35%
34.00%
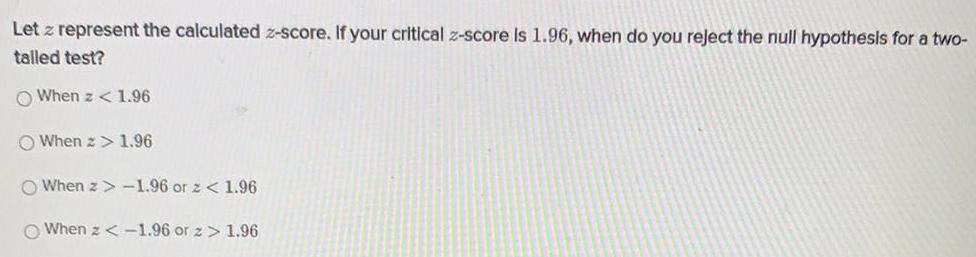
Math
ProbabilityLet z represent the calculated z-score. If your critical z-score is 1.96, when do you reject the null hypothesis for a two-talled test?
When z < 1.96
When z> 1.96
When z> -1.96 or z < 1.96
When z<-1.96 or z > 1.96

Math
ProbabilityThe size of fish is very important to commercial fishing. A study conducted in 2012 found the length of Atlantic cod caught in nets in Karlskrona to have a mean of 49.9 cm and a standard deviation of 3.74 cm (Ovegard, Berndt & Lunneryd, 2012). Assume the length of fish is normally distributed.
a. State the random variable..
b.Find the probability that an Atlantic cod has a length less than 52 cm.
c. Find the probability that an Atlantic cod has a length of more than 74 cm.
d. Find the probability that an Atlantic cod has a length between 40.5 and 57.5 cm.
e. If you found an Atlantic cod to have a length of more than 74 cm, what could you
conclude?
f.What length are 15% of all Atlantic cod longer than?

Math
ProbabilityStudents are raising money for a charity by asking for donations at a supermarket. The likelihood that a customer will donate is P(A) = 0.15. If students approach ten customers, what is the probability that none of those customers donates money?
P(A')10 = 0.10
P(A')10 = 0.15
P(A')10 = 0.20
P(A')10 = 0.85

Math
ProbabilityBroderick is playing a game where he must roll a number cube. The faces of the number cube are labeled 1 through 6. If Broderick rolls a 6 on the number cube, he must roll the cube again.
What is the probability that Broderick rolls a 6 on the number cube three times in a row?
A 1/6
B 1/18
C 1/2
D 1/216

Math
ProbabilityA jar contains 3 red, 5 green, 2 blue and 6 yellow marbles. A marble is chosen at random from the jar. After replacing it, a second marble is chosen. What is the probability of choosing a green and then a yellow marble?
a) 5/16
b) 30/16
c) 15/128
d) 30/128

Math
ProbabilityGIVEN: P(A) = 0.4 andP(B) = 0.35
If the probability of P(B∩A) = 0.75, are events A and B independent?
a) No, becauseP(A) · P(B) ≠ 0.75
b) Yes, becauseP(A) · P(B) = 0.75
c) No, becauseP(A) + P(B) = 0.75
d) Yes, becauseP(A) · P(B) = 0. 14

Math
ProbabilityWhich of the following illustrates the probability of the complement A' of an event A?
P(A')=1-P(A)
P(A) = 1- P(A')
A=P(A) + P(A')
A=P(A) P(A')
.

Math
ProbabilityThe following options are available for Inline skates.
• White, pink, yellow, blue
Tassels, striped, bells, straps
. Three, four, five wheels
What is the probability of selecting a skate with three wheels, assuming that the probabilities of selection are the same for all options?
1/48
1/12
1/4
1/3

Math
ProbabilityA hockey goaltender has a save percentage of 0.920. This means that the probability of any single shot taken on the goaltender being a goal is 0.08. What would be the expected number of goals scored on this goaltender in a game where she faced 35 shots?

Math
ProbabilityJess is baking ten batches of cookies for a bake sale. Each cookle batch has 10% probability of being burned. If A is the event that a cookle batch is burnt, what is the correct equation to find the probability that Jess will burn none of the ten batches of cookies?
P(A) = 0.9¹
P(A¹)10 = 0.910
P(A)10 = 9
P(A')= 0.110

Math
ProbabilityThe probability of a rainy day in November is 0.44. What is the probability that it rains four days in a row in November?
0.037
0.44
1.32
0.85
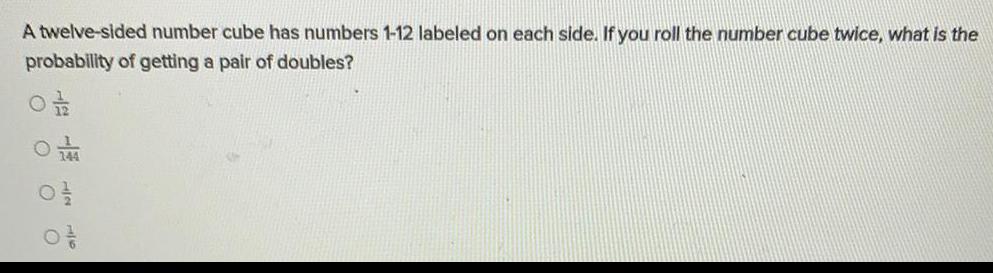
Math
ProbabilityA twelve-sided number cube has numbers 1-12 labeled on each side. If you roll the number cube twice, what is the probability of getting a pair of doubles?
1/12
1/144
1/2
1/6

Math
ProbabilityTwo students at an elementary school are to be selected at random from a group of twelve kindergartners, eight first- grade students, and five second-grade students. What is the probability that one student selected will be a second- grader and the other a kindergartner?

Math
ProbabilityTwo siblings, Alex and Brian, play a game three times in which each has an equal probability of winning. If A Is the event that Brian wins a game, what is the correct equation needed to find the probability that Brian will win none of the games?
P(A¹)³=0.5³
P(A')=5³
3P(4') = 1.5
P(A¹)³= 0.5

Math
ProbabilityA card is chosen at random from a deck of 52 cards. It is then replaced and a second card is chosen. What is the probability of choosing a jack and then an eight?
P(jack and 8)
a) 16/169
b) 4/52
c) 16/52
d)1/169

Math
ProbabilityA deck of thirty cards consists of ten red, ten yellow, and ten orange cards. If five cards are drawn, what is the probability of selecting five red cards?

Math
ProbabilityIn a class of 22 students, 5 have a brother and 13 have a sister. There are 2 students who have a brother and a sister. What is the probability that a student who has a brother also has a sister?

Math
ProbabilityJess is baking ten batches of cookies for a bake sale. For every batch of 10 cookles, there is a 0.9 probability that no cookies will be burned. What is the probability that at least one batch of cookies has a burned cookie?
0.35
0.65
0.81
0.90