Basic Math Questions and Answers
Math
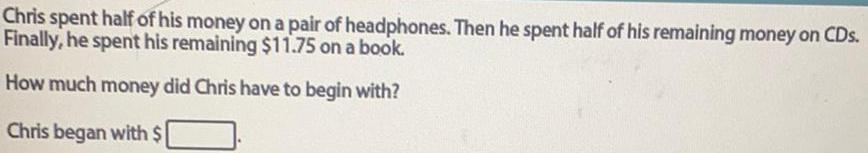
Basic MathChris spent half of his money on a pair of headphones. Then he spent half of his remaining money on CDs. Finally, he spent his remaining $11.75 on a book.
How much money did Chris have to begin with?
Chris began with $

Math
Basic MathOne pipe fills a storage pool in 20 hours. A second pipe fills the same pool in 10 hours. When a third pipe is added and all three are used to fill the pool, it takes only 5 hours. Find how long it takes the third pipe to do the job.
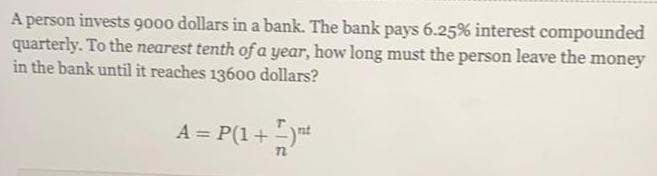
Math
Basic MathA person invests 9000 dollars in a bank. The bank pays 6.25% interest compounded
quarterly. To the nearest tenth of a year, how long must the person leave the money
in the bank until it reaches 13600 dollars?
A = P(1 + 7) n²
nt
Math
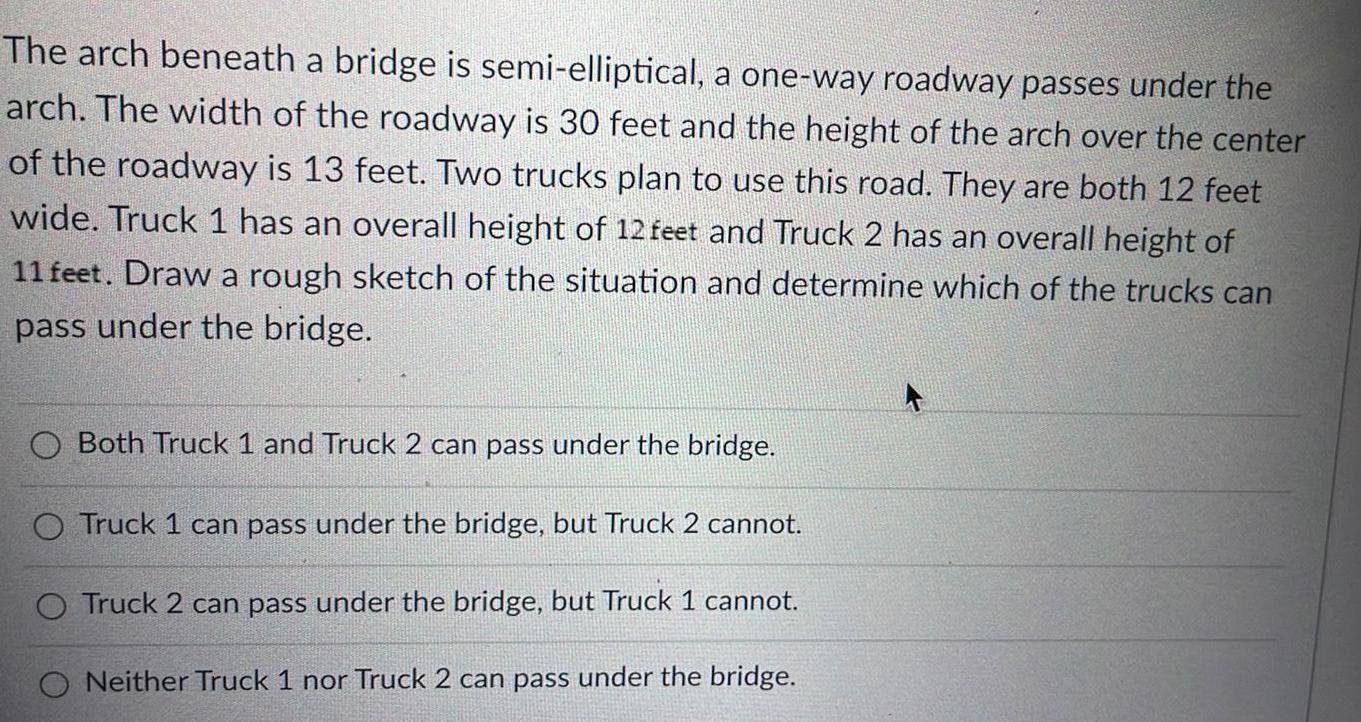
Basic MathThe arch beneath a bridge is semi-elliptical, a one-way roadway passes under the arch. The width of the roadway is 30 feet and the height of the arch over the center of the roadway is 13 feet. Two trucks plan to use this road. They are both 12 feet wide. Truck 1 has an overall height of 12 feet and Truck 2 has an overall height of 11 feet. Draw a rough sketch of the situation and determine which of the trucks can pass under the bridge.
Both Truck 1 and Truck 2 can pass under the bridge.
Truck 1 can pass under the bridge, but Truck 2 cannot.
Truck 2 can pass under the bridge, but Truck 1 cannot.
Neither Truck 1 nor Truck 2 can pass under the bridge.

Math
Basic MathPeople are waiting in line for a theater premiere. Every 5th person in line will receive a free theater ticket. Every 6th person will receive a gift card for $40. Which person is the first to receive both prizes? Explain.

Math
Basic Math(b) that a music download was neither tropical nor regional
Next, calculate the relative frequency that a download was neither tropical nor regional.
We are given that, of the 400 total downloads, 200 were regional, 110 were pop-rock, 80 were tropical, and 10 were urban. The categories of music that are not tropical and not regional are pop-rock and urban. So, the frequency of music downloads that are neither tropical nor regional is equal to the frequency of music downloads that are pop-rock or urban. The frequency of music downloads that are pop-rock or urban is 110 + 10 = 120 So the frequency of music downloads that are neither tropical nor regional is

Math
Basic MathPaul, a janitor, must move a large shipment of books from the first floor to the fifth floor. The sign on the elevator reads "maximum weight 900 pounds." If each box of books weighs 80 pounds, find the maximum number of boxes that Paul should place in the elevator if he does not ride.

Math
Basic MathThe population of a certain species of oak tree in a national forest declines each year by one-fourth. So, after each year, only
three-fourths of the trees present at the beginning of the year remain.
Which of the following describes the rate of decline in the population of the species of oak tree?
OA. neither linear nor nonlinear
SOB.
both linear and nonlinear
OC. nonlinear
O D.
linear

Math
Basic MathUse the formula R = log
t
In May 2008, an earthquake measuring 6.8 on the Richter scale struck near the east coast of Honshu, Japan. In March 2011, a quake measuring 9.0 struck that same region. How many times more
intense was the 2011 quake than the one in 2008? (Round your answer to one decimal place.)
times

Math
Basic MathA large wind turbine can
power 598 homes. A
company had 4 turbines
and then built 5 more.
homes can
How mar
many
the company power with
its wind turbines?
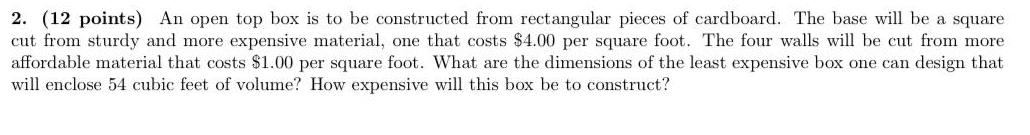
Math
Basic Math2. (12 points) An open top box is to be constructed from rectangular pieces of cardboard. The base will be a square
cut from sturdy and more expensive material, one that costs $4.00 per square foot. The four walls will be cut from more
affordable material that costs $1.00 per square foot. What are the dimensions of the least expensive box one can design that
will enclose 54 cubic feet of volume? How expensive will this box be to construct?

Math
Basic MathTruck #1 can haul 480 tons of steel in 24 trips. Truck #2 can haul the same amount of steel in 48 trips.
How many trips would it take if both trucks are used?
O 10 Trips
20 Trips
1 pt
O 36 Trips
O 16 Trips

Math
Basic MathA ball is thrown from a height of 164 feet with an initial downward velocity of 12 ft/s. The ball's height h (in feet) after seconds is given by the following.
h=164-12t - 16t²
How long after the ball is thrown does it hit the ground? Round your answer(s) to the nearest hundredth. (If there is more than one answer, use the "or" button.)

Math
Basic Math1190
1+28.75e-0.357 represents the population (in thousands)
13. The logistic growth model P =
of a bacterium in a culture tube after t hours.
a) What was the initial amount of bacteria in the population?
b) When will the population be equal to 500,000?
c) What is the carrying capacity of the population?

Math
Basic MathVideogame data: We collected data on the top 50 best-selling video games. These are the variable descriptions:
Metascore: Score out of 100, based on major critic reviews as provided by Metacritic.com
Total Worldwide Sales: Total number copied sold worldwide since December 31, 2015.
GameRankings: Score out of 100%, based on an average scores from websites and magazine reviews.
We used Metascore ratings as an explanatory variable and GameRankings ratings as the response variable in a
linear regression. The r2 value is 48%. With Total Worldwide Sales as the explanatory variable and
GameRankings ratings as the response variable in a linear regression, the r2 value is 62%. Using the ² value,
which is a better predictor of a videogames's GameRankings score: Metascore or Total Worldwide Sales?
Metascore
Total Worldwide Sales

Math
Basic MathMr. Young built a greenhouse 42 feet wide, 110 feet long, 5 feet to the eaves, and 14 feet to the ridge cap.
a. How many square feet of plastic does it take to enclose the greenhouse?
b. What is the slope of the roof?
c. What angle does the roof make with the horizontal?
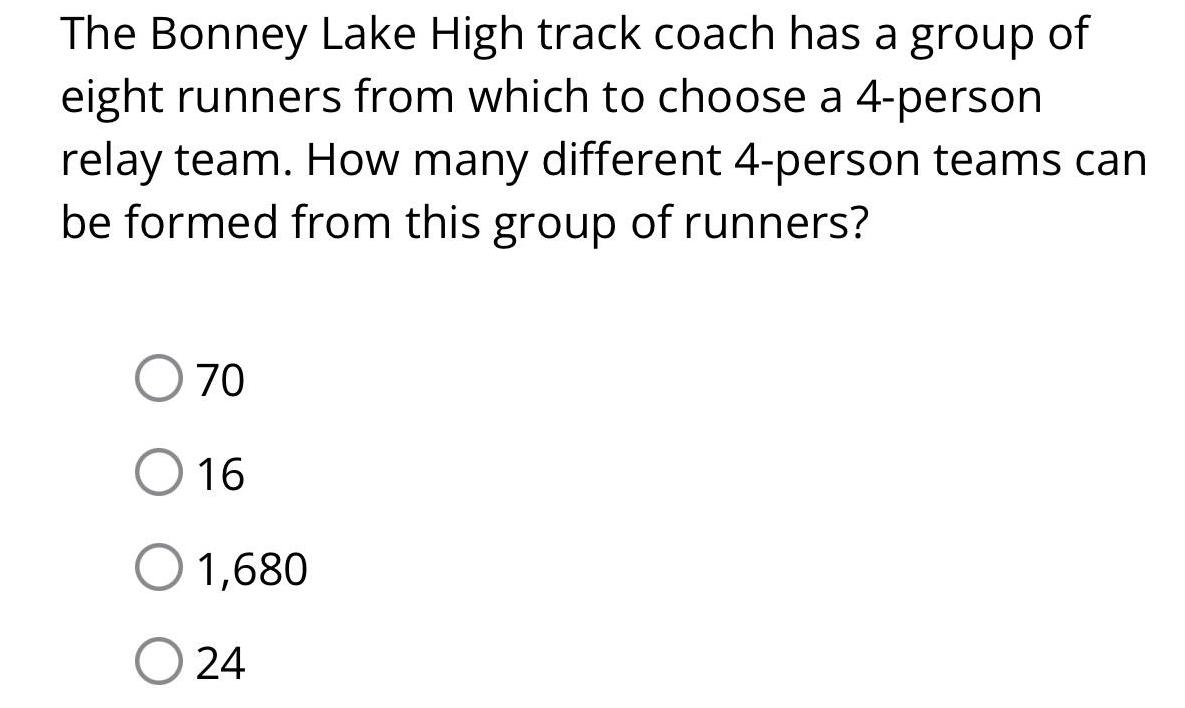
Math
Basic MathThe Bonney Lake High track coach has a group of eight runners from which to choose a 4-person
relay team. How many different 4-person teams can be formed from this group of runners?
70
16
1,680
24
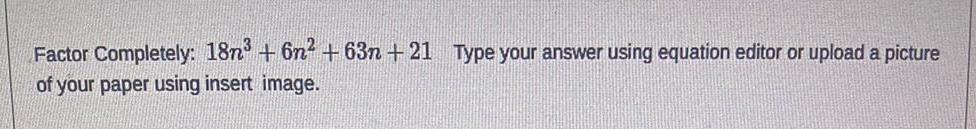
Math
Basic MathFactor Completely: 18n³ +6n² +63n+21 Type your answer using equation editor or upload a picture
of your paper using insert image.

Math
Basic MathA truck travels north from a warehouse at an average speed of 35 MPH. A second truck travels south from a store at an average speed of 40 MPH? If the store is 150 mile from the warehouse, how many miles from the warehouse will the trucks meet?
80 miles
75 miles
60 miles
70 miles

Math
Basic MathA stone is dropped from the upper observation deck of a tower, 700 m above the ground. (Assume g = 9.8 m/s².)
(a) Find the initial values of the velocity v (in m/s) and the distance s (in meters) of the stone above the ground.
v(0) =
s(0) =
Find the velocity (in m/s) of the stone at time t.
v(t) =
s(t): =
Find the distance (in meters) of the stone above ground at time t.
(b) How long (in seconds) does it take the stone to reach the ground? (Round your answer to two decimal places.)
(c) With what velocity (in m/s) does it strike the ground? (Round your answer to one decimal place.)
(d) If the stone is thrown downward with a velocity of 9 m/s, how long (in seconds) does it take to reach the ground? (Round your answer to two decimal places.)

Math
Basic MathEden bought 15 tickets to a soccer game. The group rate saved her $4.75 per ticket. She paid a total of $307.50 for the tickets What was the regular price of a ticket to the game?
Enter your answer in the box.
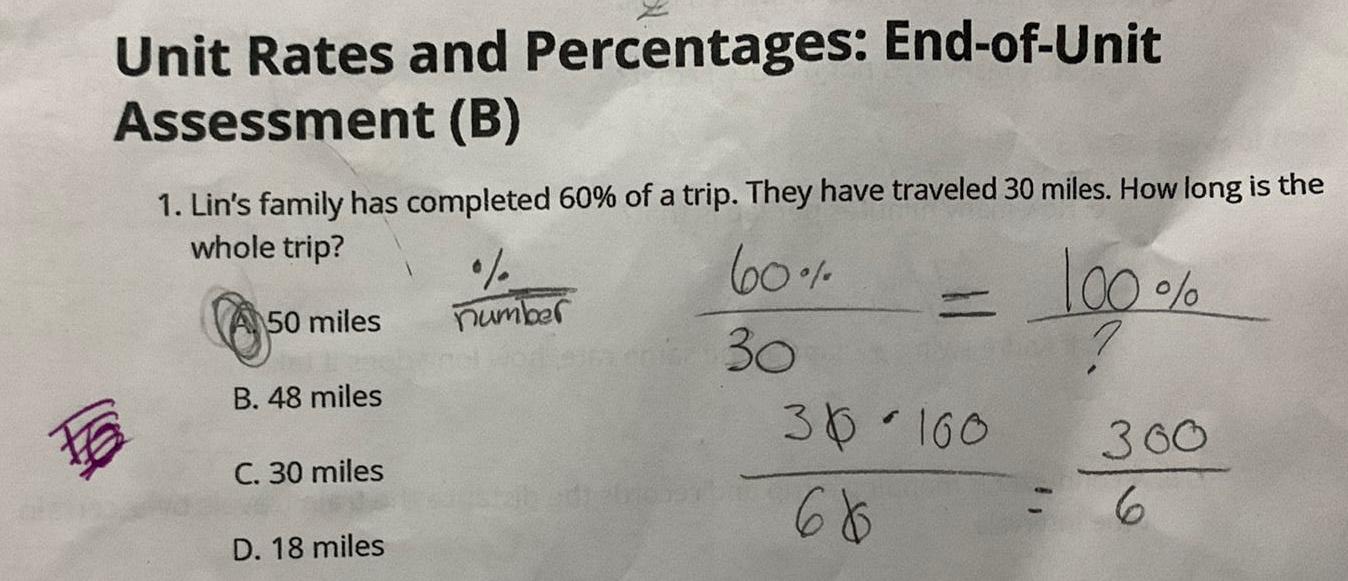
Math
Basic MathLin's family has completed 60% of a trip. They have traveled 30 miles. How long is the
whole trip?
A.50 miles
B. 48 miles
C. 30 miles
D. 18 miles
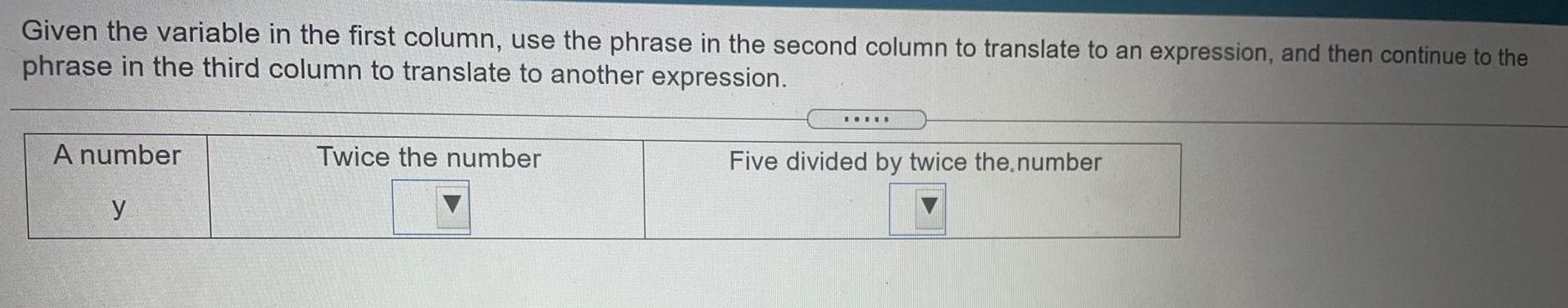
Math
Basic MathGiven the variable in the first column, use the phrase in the second column to translate to an expression, and then continue to the phrase in the third column to translate to another expression.
A number
Twice the number
Five divided by twice the number

Math
Basic MathYou are building a hexagonal patio with sides of 10 ft. (Note: For help, see Figure 12-9 on page 163.) You are flooring it with 4" of concrete.
a. What is the perimeter of the patio?
b. What is the measure of the interior angle?
c. How many equilateral triangles are formed have their vertices at the center of the hexagon?
d. What is the height of one of the equilateral triangles? (Note: For help, see section 2.2)
e. What is the area of the hexagon?
f. How many yard (cubic yards) of concrete are needed?

Math
Basic MathWhich of the following statements are true?
To solve 2-95= 55+4, you can subtract 5s from each side of the equation.
The equation-20-10d=5(-2d-4) has one solution, d = 2.
The solution of 6 (p+ 3) = 5(p-3) is all real numbers.
The equation -5(5x+9)=-45- 25x is an identity.
Linear equations do not always have one solution.

Math
Basic MathThe amount of sales tax on a new car varies directly to the purchase price of the car. On a $25,000 car, sales tax is $1,750. What's the purchase price of a new car with $3,500 in sales tax?

Math
Basic MathWhat does it mean for two shapes to be "similar?"
Corresponding angles and sides are proportional.
Corresponding angles are proportional and corresponding sides are congruent.
Corresponding angles and sides are congruent.
Corresponding angles are congruent and corresponding sides are proportional.

Math
Basic MathSolve the equation. Determine whether the equation has one solution, no solution, or infinitely many solutions.
4c-20=-20+4c
infinitely many solutions
c=0; one solution
c--4; one solution
no solution
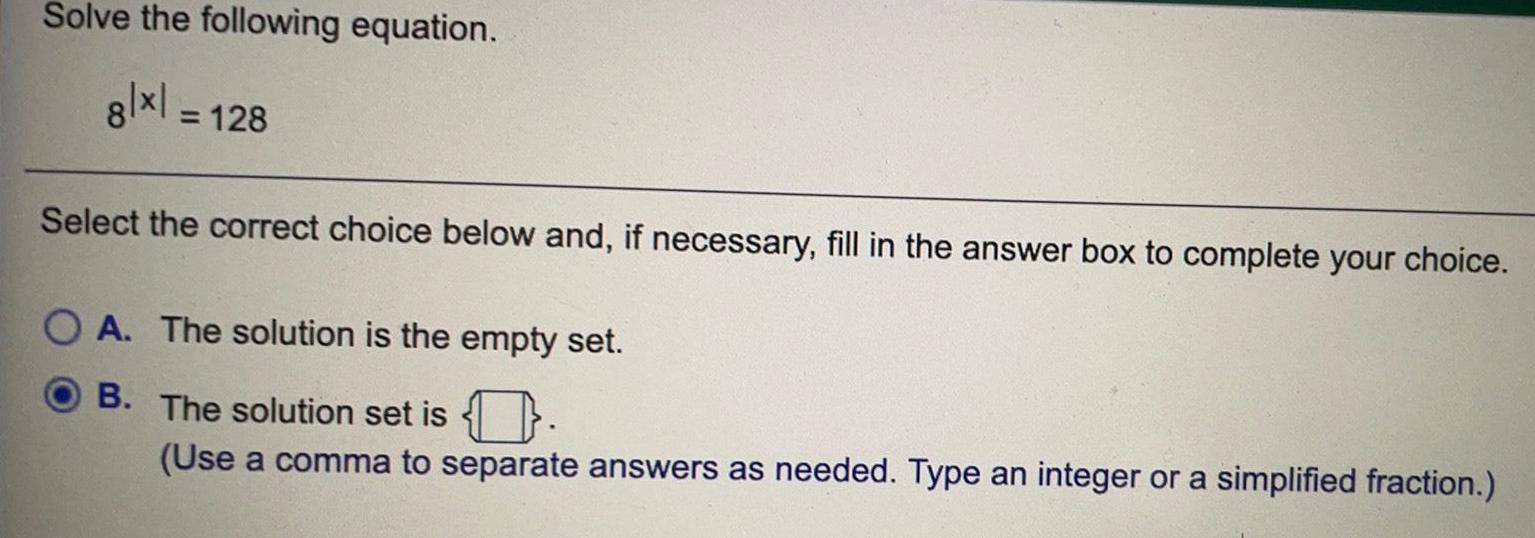
Math
Basic MathSolve the following equation.
8lx| = 128
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution is the empty set.
B.The solution set is
(Use a comma to separate answers as needed. Type an integer or a simplified fraction.)
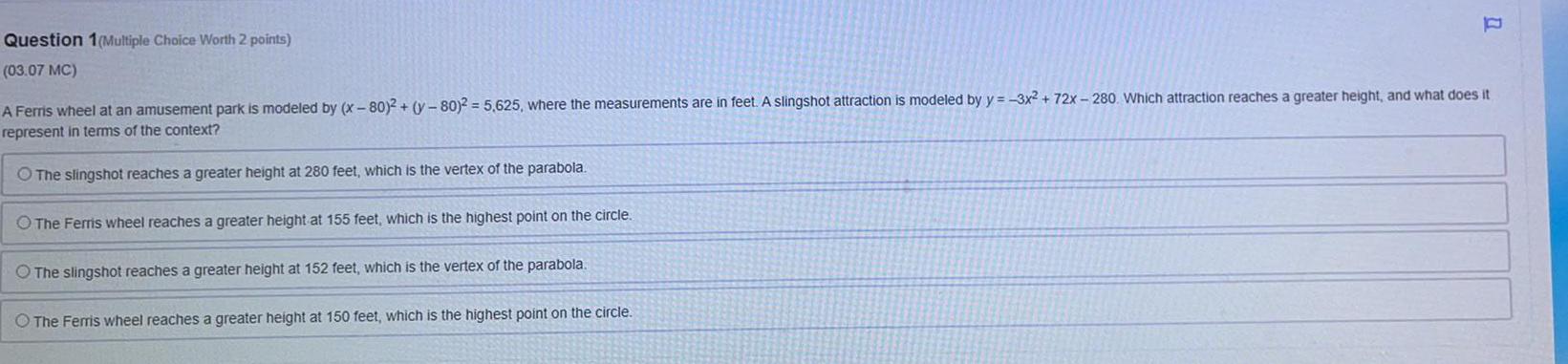
Math
Basic MathA Ferris wheel at an amusement park is modeled by (x-80)2 + (y-80)2 = 5,625, where the measurements are in feet. A slingshot attraction is modeled by y=-3x² + 72x-280. Which attraction reaches a greater height, and what does it represent in terms of the context?
The slingshot reaches a greater height at 280 feet, which is the vertex of the parabola.
The Ferris wheel reaches a greater height at 155 feet, which is the highest point on the circle.
The slingshot reaches a greater height at 152 feet, which is the vertex of the parabola.
The Ferris wheel reaches a greater height at 150 feet, which is the highest point on the circle.
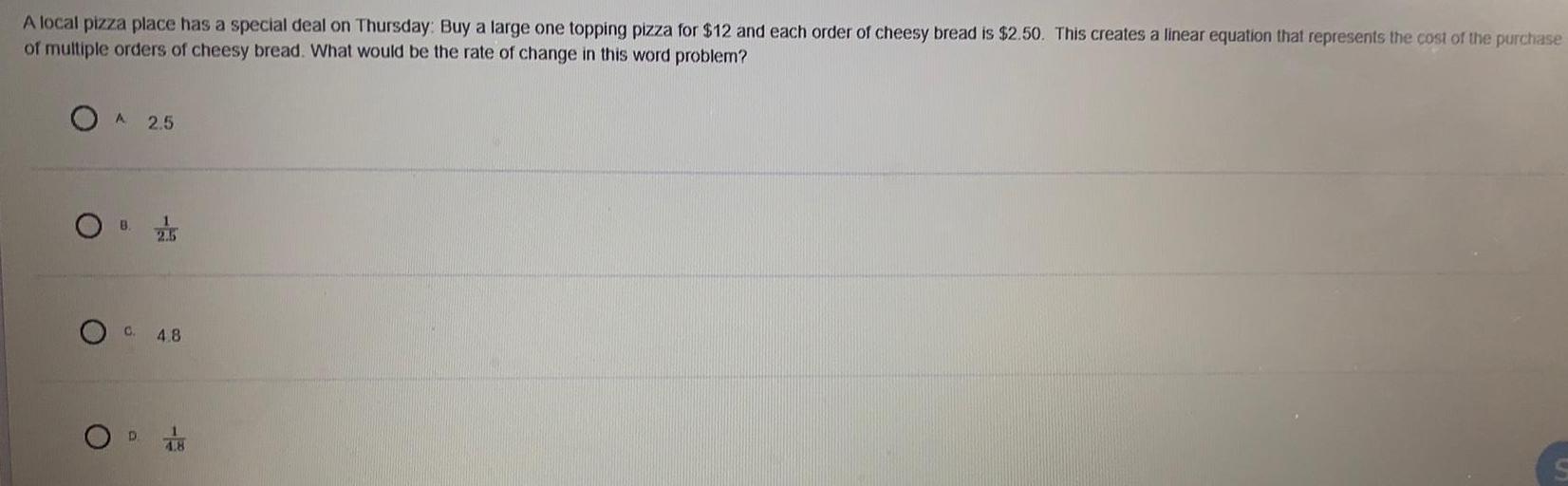
Math
Basic MathA local pizza place has a special deal on Thursday: Buy a large one topping pizza for $12 and each order of cheesy bread is $2.50. This creates a linear equation that represents the cost of the purchase
of multiple orders of cheesy bread. What would be the rate of change in this word problem?
O A 2.5
OB.
O
2.5
C. 4.8
O D
4.8
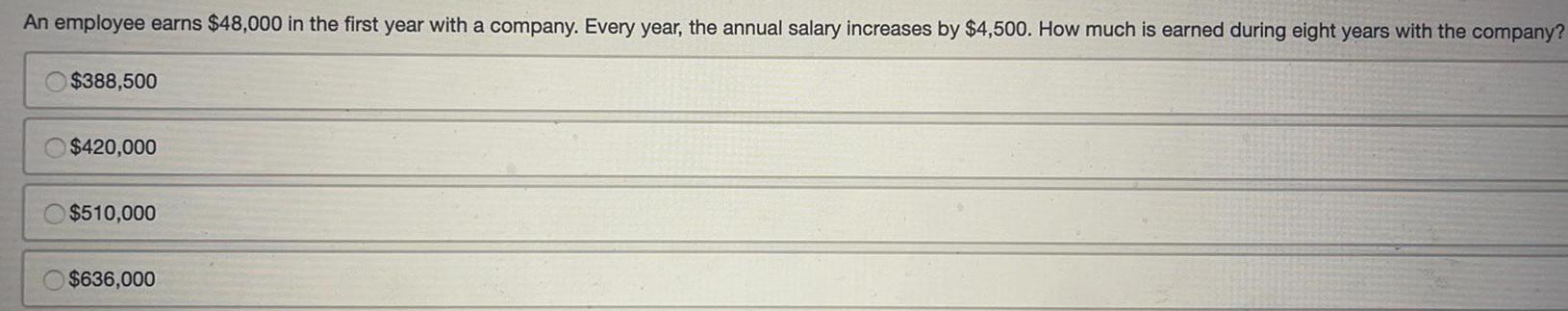
Math
Basic MathAn employee earns $48,000 in the first year with a company. Every year, the annual salary increases by $4,500. How much is earned during eight years with the company?
$388,500
$420,000
$510,000
$636,000
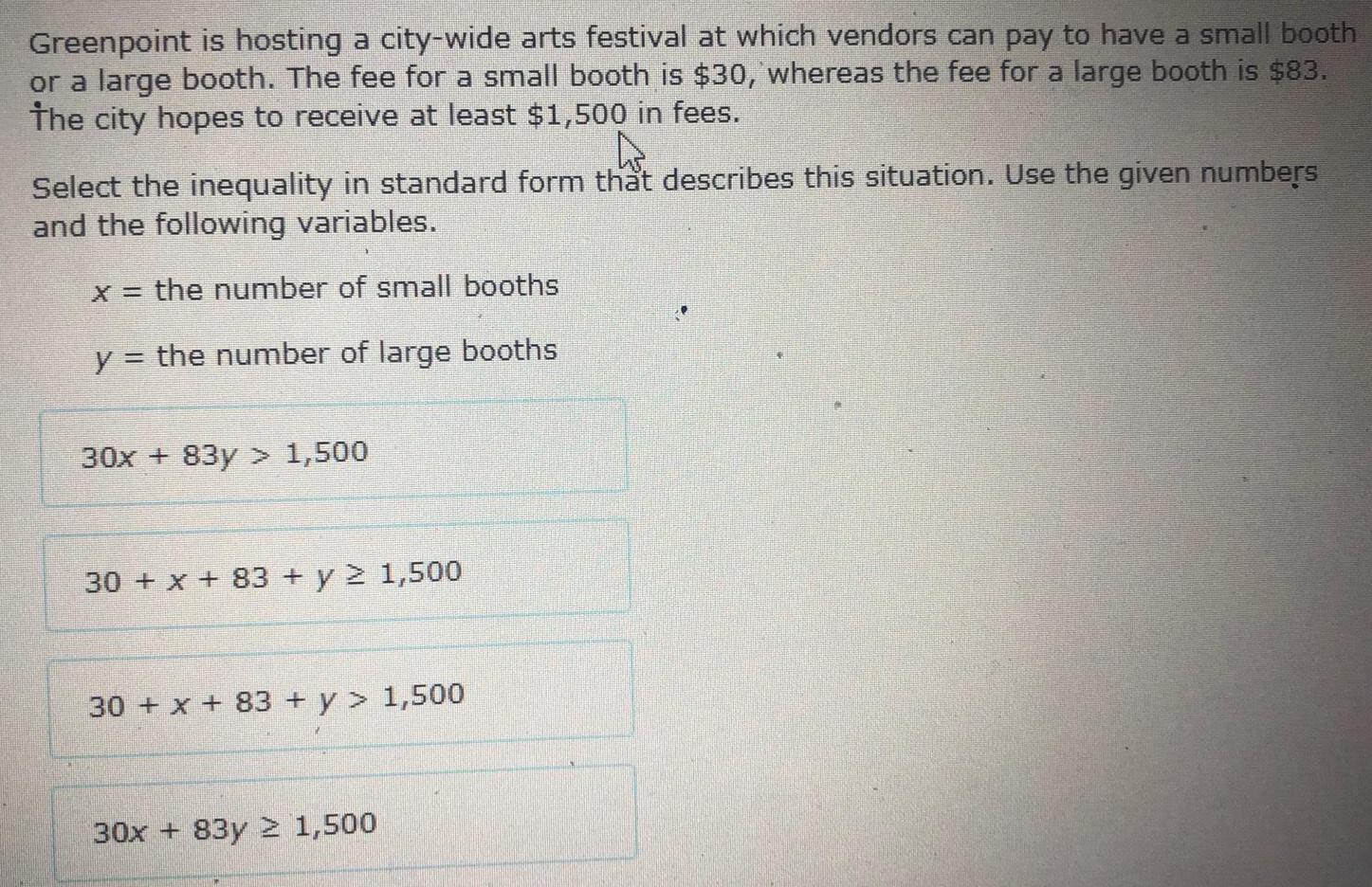
Math
Basic MathGreenpoint is hosting a city-wide arts festival at which vendors can pay to have a small booth
or a large booth. The fee for a small booth is $30, whereas the fee for a large booth is $83.
The city hopes to receive at least $1,500 in fees.
A
Select the inequality in standard form that describes this situation. Use the given numbers
and the following variables.
x = the number of small booths
y = the number of large booths
30x + 83y > 1,500
30 + x + 83 + y ≥ 1,500
30 + x + 83 + y > 1,500
30x + 83y2 1,500

Math
Basic MathSamantha donated 9 T-shirts to charity and has 16 left. Which equation could be used to find x, the number of T-shirts
Samantha had originally?
OA. x-16=9
OB. X+16=-9
OC. x+9=16
O D. X-9=-16
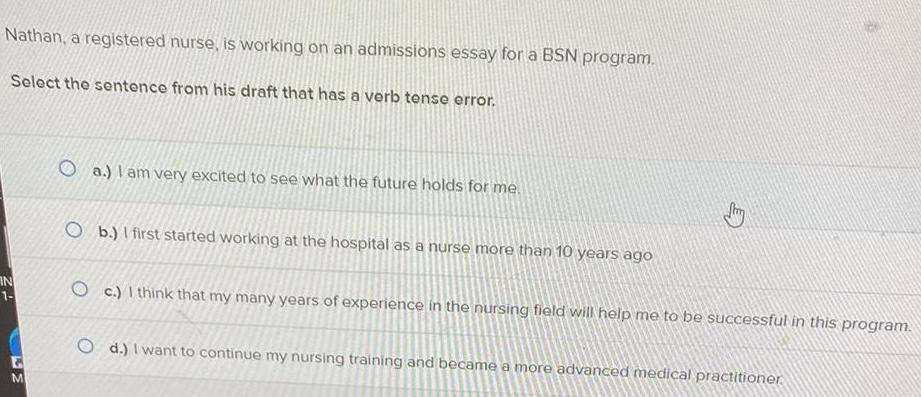
Math
Basic MathNathan, a registered nurse, is working on an admissions essay for a BSN program.
Select the sentence from his draft that has a verb tense error.
a.) I am very excited to see what the future holds for me.
b.) I first started working at the hospital as a nurse more than 10 years ago
c.) I think that my many years of experience in the nursing field will help me to be successful in this program.
d.) I want to continue my nursing training and became a more advanced medical practitioner.

Math
Basic Math3. Model with Math Clare had t seashells.
After she bought 8 more seashells, she had
24 seashells. Write and solve an equation to find
the number of seashells Clare started with.

Math
Basic MathLincoln, Nebraska lies directly north of Dallas, Texas. Lincoln is
at a latitude of 40.5° N and Dallas is at a latitude of 32.5° N.
Assuming the radius of the earth is 3,960 miles, how far apart are
these cities?

Math
Basic MathStacey purchased four concert tickets and paid more than $200. The amount she paid for each ticket was equal to the
face value plus a $15 service charge. Select the inequality where x represents the possible face value of each ticket and the
solution of that inequality.
OA. 4(x+15) > 200
The face value of a ticket is at least $46.25.
OB. 4x+15 > 200
O D.
The face value of a ticket is at least $46.25.
OC. 4(x+15) > 200
The face value of a ticket is at least $35.00.
4x+15 > 200
The face value of a ticket is at least $35.00.
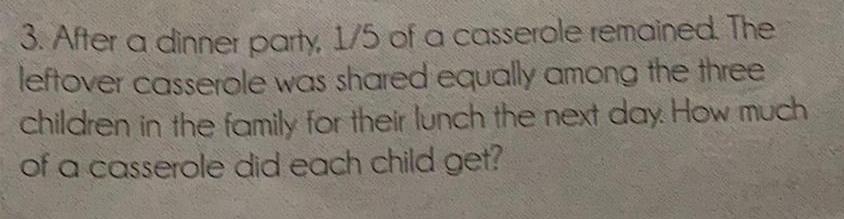
Math
Basic Math3. After a dinner party, 1/5 of a casserole remained. The
leftover casserole was shared equally among the three
children in the family for their lunch the next day. How much
of a casserole did each child get?

Math
Basic MathBrian paid a total of $46.44 for a jacket at a department store. The jacket was on sale for 20% off the regular price, and he
used a coupon for an additional $5.00 off of the discounted price. Brian had to pay 8% sales tax on the cost of the jacket
after any discounts and coupons.
Select the equation where p represents the original price of the jacket and the solution of that equation.
OA. 1.08(0.8(p-5)) = 46.44
The original price of the jacket was $58.75.
OB. 1.08(0.8p - 5) = 46.44
The original price of the jacket was $58.75.
OC. 1.08(0.8(p-5)) = 46.44
The original price of the jacket was $60.00.
OD. 1.08(0.8p-5) = 46.44
The original price of the jacket was $60.00.

Math
Basic MathWhich expression is not equal to 30?
(9 x 10) (4+1) + 12
÷
(2 x 60 ÷ 30) x (24 + 2)
(12020) x (2+3)
X
2+ (30-8 ÷ 4)

Math
Basic MathSuppose that $2500 is invested in an account that pays interest compounded continuously. Find the amount of time that it would take for the account to grow to $4000 at 4.25%. It would take approximately years. (Round to the nearest tenth.)

Math
Basic MathA pilot can travel 400 miles with the wind in the same amount of time as 352 miles against the wind. Find the speed of the wind if the
pilot's speed in still air is 235 miles per hour.
Math
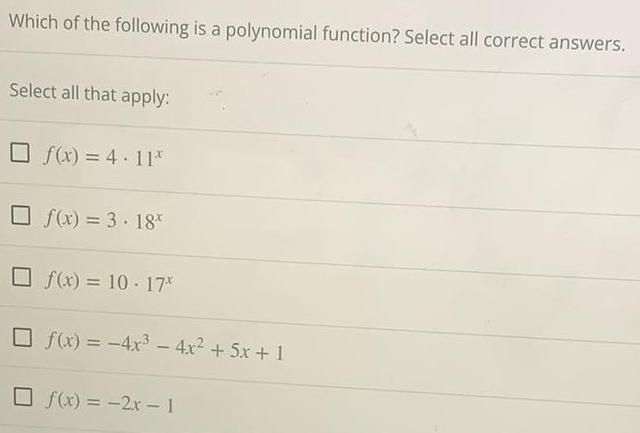
Basic MathWhich of the following is a polynomial function? Select all correct answers.
Select all that apply:
f(x) = 4.11x
f(x) = 3.18x
f(x) = 10.17x
f(x) = -4x³ - 4x² + 5x+1
f(x) = -2x - 1

Math
Basic MathWhich functions have graphs with a slant asymptote? Select all that apply.
Select all that apply:
f(x) = x+1
x²
f(x) = 3x²
5x
☐ f(x) = 2/1/2
2x²
☐ f(x) = 2x²+1
43
□ f(x) =
3x³
x+1

Math
Basic MathThe Lincoln Memorial has dimensions of 189.7 ft wide, 118.5 ft deep, and
99 ft tall. (The structure, not the statue of Lincoln.)
Sam is making a model of this to use in a presentation in his American
History class. If his model is 1 foot tall, what are the width and length
dimensions?
Image Source
Lincoln Memorial, public domain
Ow= 61.84 in, L = 158.48 in
Ow= 1.92 ft, L = 1.20 ft
Ow=18780.3 in, L= 11731.5 in
Ow= .52 ft, L = .84 ft

Math
Basic MathWhat interest will be earned if $7000 is invested for 4 years at 11% compounded monthly? (Round your answer to the nearest cent.)
$3864.39
X

Math
Basic MathConsider the graph of the function f(x) =x²-4/x²-12x+20
Find the x-value of the removable discontinuity of the function.
Provide your answer below:
The removable discontinuity occurs at x =

Math
Basic MathLet's "Texas Size" the "Rattlesnake" drink. The ratios are 32 parts Whiskey, 24 parts egg, 6 parts lemon, 2
parts sugar, and 1 part Absinthe. If I'm planning on making 65 gallons, how many gallons of
Absinthe will I need?
O2 gallons
32 gallons
1 gallon
O 3 gallons