Solution of triangles Questions and Answers

Math
Solution of trianglesAn art student wants to take a triangle with side lengths of 9, 9, and 15 and enlarge it so that the longest side of a new triangle is 22.5. What will be the length of the other two sides of the new triangle?
13.5
12
11.5
10
Math
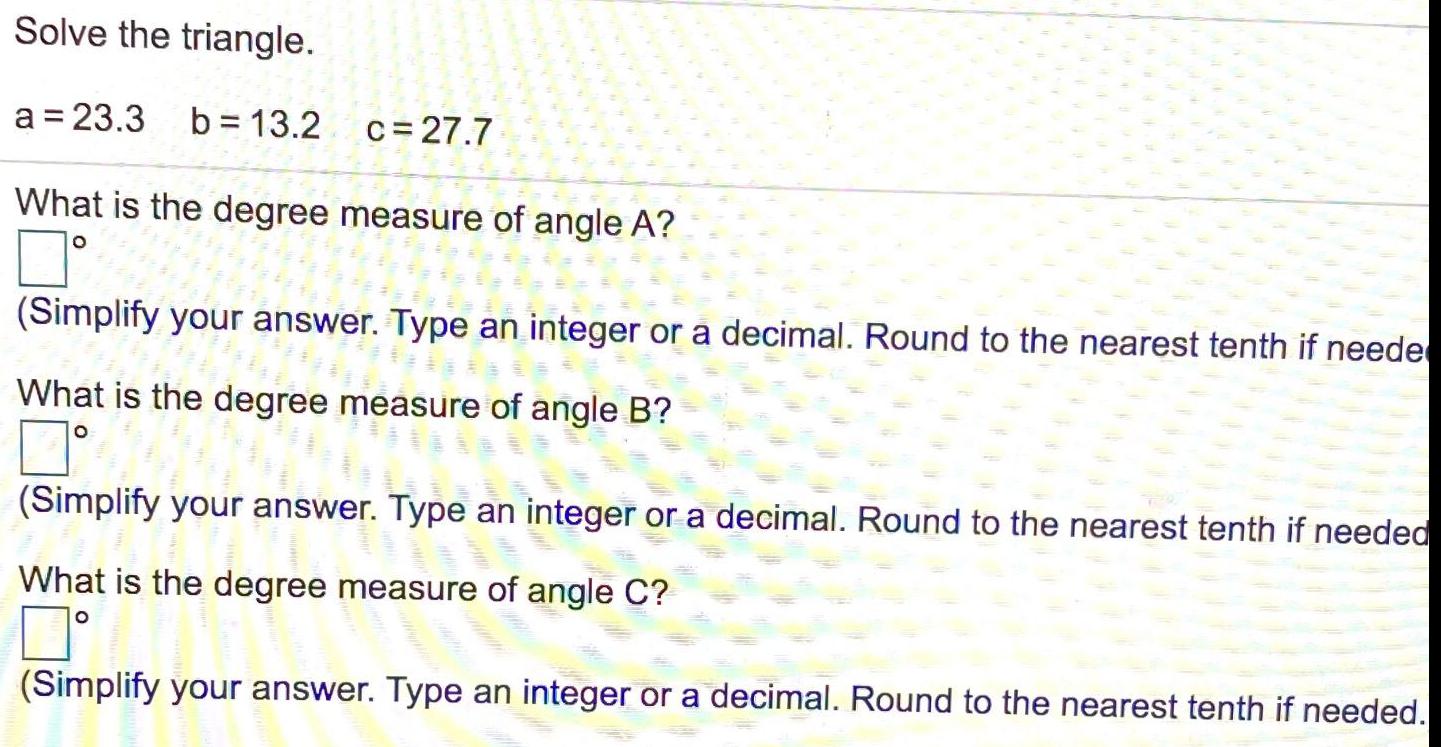
Solution of trianglesSolve the triangle.
a = 23.3 b = 13.2 c=27.7
What is the degree measure of angle A?
(Simplify your answer. Type an integer or a decimal. Round to the nearest tenth if needed.
What is the degree measure of angle B?
(Simplify your answer. Type an integer or a decimal. Round to the nearest tenth if needed
What is the degree measure of angle C?
(Simplify your answer. Type an integer or a decimal. Round to the nearest tenth if needed.

Math
Solution of trianglesA triangle in quadrant IV has been rotated 90° counterclockwise
around the origin. What do you know about the image and the pre-
Image?
They are congruent and their corresponding sides and angles are equal.
Their corresponding sides and angles are equal but they are not
congruent.
They are not congruent and their corresponding parts are not congruent.
They are congruent but their corresponding sides and angles are not
equal.

Math
Solution of trianglesA street light is at the top of a 26 ft pole. A 5 ft tall girl walks along a straight path away from the pole with a speed of 7 ft/sec.
At what rate is the tip of her shadow moving away from the light (ie away from the top of the pole) when the girl is 36 ft away from the pole?
How fast is her shadow lengthening?


Math
Solution of trianglesAccording to the Angle-Angle Similarity Postulate, if two triangles have two corresponding angles that are
similar, congruent
different, similar
congruent, congruent
congruent, similar
then the triangles

Math
Solution of trianglesWhat is the relationship between similar triangles and the altitude of a right triangle? Select all that apply.
An altitude drawn from the right angle in a right triangle divides the triangle into two sub-triangles.
Corresponding sides in right triangles are parallel.
Missing sides of right triangles cannot be calculated using the properties of altitude and similar triangles.
The sub-triangles are similar to the original triangle.

Math
Solution of triangles25. Tasha wants to measure the height of a tree that grows at an angle of 85°with respect to the ground. When she is 80 feet away from the base of the tree she looks up. The angle from the ground to the top of the tree is 25°. Approximately, how tall is the tree?
212.989 feet
35.979 feet
84.810 feet
177.880 feet

Math
Solution of trianglesWhich of the following triangles is an acute triangle?
a triangle with side lengths of 8, 15, 17
a triangle with side lengths of 4, 6, 7
a triangle with side lengths of 12, 14, 26
a triangle with side lengths of 8, 12, 15

Math
Solution of trianglesJillian has a triangular-shaped garden with side lengths of 11 feet, 12 feet, and 15 feet. Which of the following describes the garden and its shape? Select all that apply.
The area of the garden is about 65.2 feet squared.
The angle across from the side with a length of 15 feet is about 81.3".
The area of the garden is about 20.0 feet squared.
The angle across from the side with a length of 15 feet is about 52.3.

Math
Solution of trianglesIn Texas, the distance from Austin to Brownwood is 141 miles. It is 171 miles from Brownwood to Corsicana and 157 miles from Corsicana to Austin.
Determine the angle between the routes from Brownwood. Show your work.

Math
Solution of trianglesShaaron and her two sisters, Anna and Bonnie, have apps on their phones so that at any moment Shaaron can see the distance in kilometres between her and each of her two sisters. Shaaron looks at her phone and sees:
Anna - 15 km away from you
Bonnie - 20 km away from you
Which of these cannot be the distance between Anna and Bonnie?
A. 10 km
B. 20 km
C. 30 km
D .40 km

Math
Solution of trianglesPat needs to determine the height of a tree before cutting it down to be sure that it will not fall on a nearby fence. The angle of elevation of the tree from one position on a flat path from the tree is H=40°, and from a second position L = 50 feet farther along this path it is B = 30°. What is the height of the tree?
The height of the tree is approximately ft.
(Do not round until the final answer. Then round to the nearest tenth as needed.)
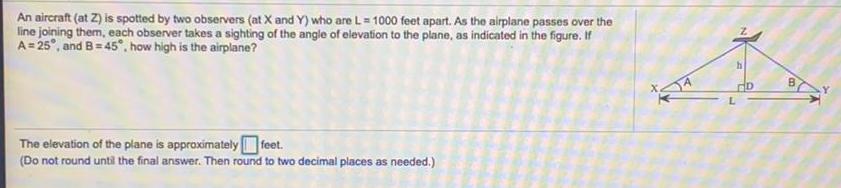
Math
Solution of trianglesAn aircraft (at Z) is spotted by two observers (at X and Y) who are L=1000 feet apart. As the airplane passes over the line joining them, each observer takes a sighting of the angle of elevation to the plane, as indicated in the figure. If A=25°, and B=45°, how high is the airplane?
The elevation of the plane is approximately feet.
(Do not round until the final answer. Then round to two decimal places as needed.)
Math
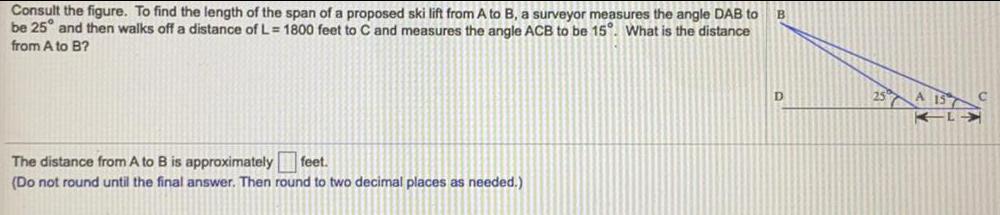
Solution of trianglesConsult the figure. To find the length of the span of a proposed ski lift from A to B, a surveyor measures the angle DAB to B be 25 and then walks off a distance of L= 1800 feet to C and measures the angle ACB to be 15°. What is the distance from A to B?
The distance from A to B is approximately feet.
(Do not round until the final answer. Then round to two decimal places as needed.) D

Math
Solution of trianglesUse the triangle shown on the right and the given information to solve the triangle.
a=9, A=25°; find b, c, and B
b= (Round to two decimal places as needed.)
c= (Round to two decimal places as needed.)

Math
Solution of trianglesSolve this triangle. Offer the exact solution for the missing angle, but round your answers for each missing side length to the nearest whole meter.
A = 39.70°, C = 30.35", b= 39.74m
A = 39.70°
B =
C = 30.35°
a=
b= 39.74m
C
Math
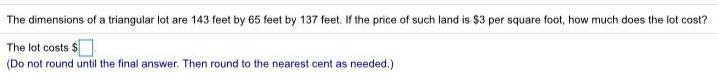
Solution of trianglesThe dimensions of a triangular lot are 143 feet by 65 feet by 137 feet. If the price of such land is $3 per square foot, how much does the lot cost? The lot costs $ (Do not round until the final answer. Then round to the nearest cent as needed.)

Math
Solution of trianglesRey says that this is an obtuse triangle. She is correct, which best describes the reason she justified her answer:
7,24,28
7 + 24 is greater than 28
28² = 7² +24²
28² >7² +24²
28²< 7² +24²

Math
Solution of trianglesDetermine whether the side lengths could form a triangle. Prove your answer with an inequality.
5 ft., 2 ft., 10 ft.
37 in., 18 in., 25 in.
Given the measures of two sides of a triangle, find the range of values for the third side.
3 km, 48 km
11 ft ,24 ft

Math
Solution of trianglesTriangle ABC has sides with lenths of 3, 6, and 8. Classify each of the transformations described as producing a triangle similar to triangle ABC or a triangle not similar to triangle ABC.
Match each transformation to either similar to triangle ABC or not similar to triangle ABC.
Column A Column B
1. ___ Multiply each side length by 3.5. a.Similar to triangle ABC.
2. ____ Add 12 to each side length. b. Not similar to triangle ABC.
3. _____Subtract 2 from each side length.
4. ___Divide each side length by 0.75.
Math
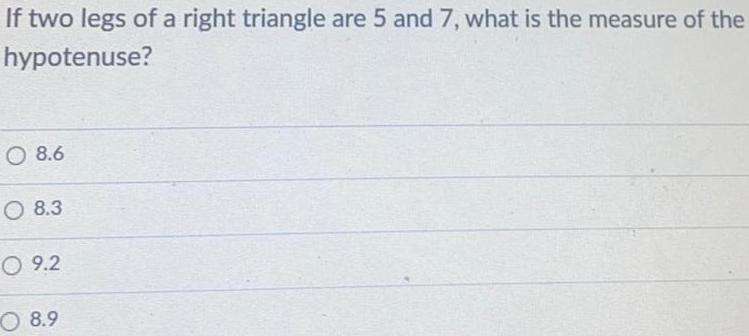
Solution of trianglesIf two legs of a right triangle are 5 and 7, what is the measure of the hypotenuse?
8.6
8.3
9.2
8.9
Math
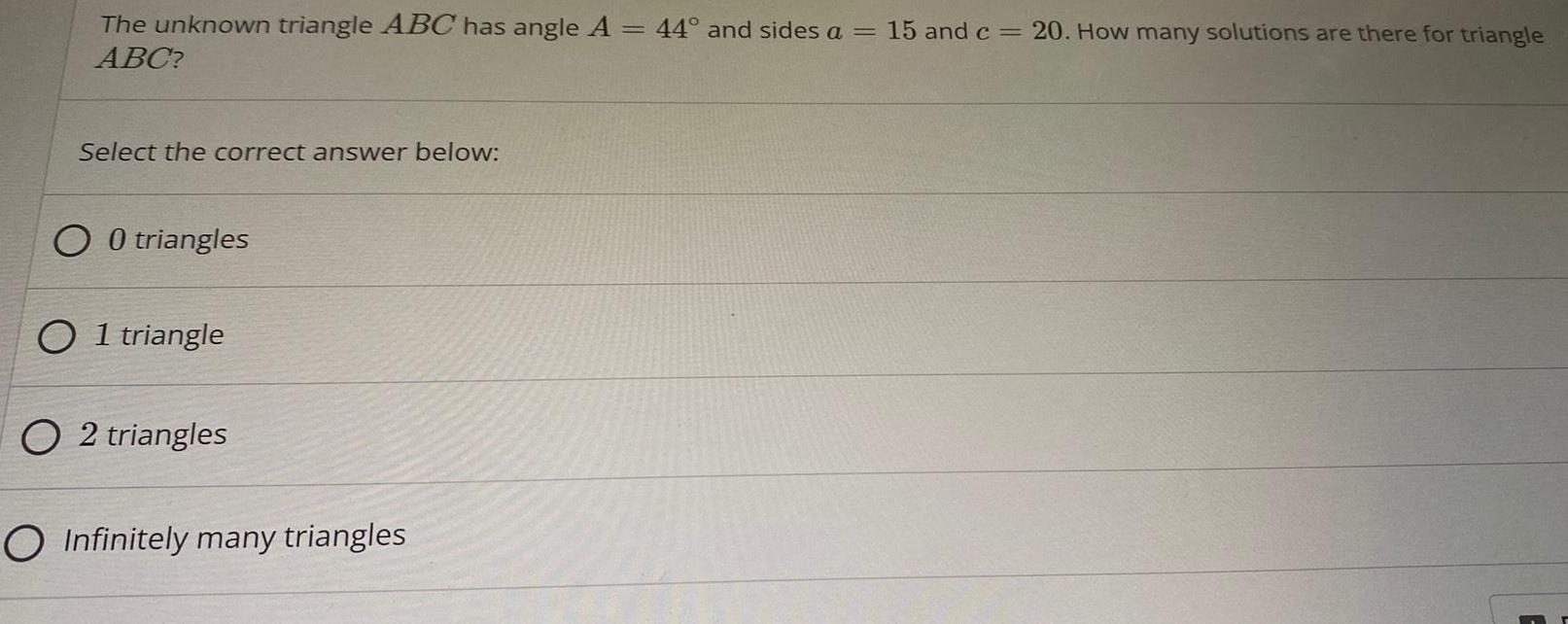
Solution of trianglesThe unknown triangle ABC has angle A = 44° and sides a = 15 and c = 20. How many solutions are there for triangle ABC?
Select the correct answer below:
0 triangles
1 triangle
2 triangles
Infinitely many triangles

Math
Solution of trianglesThe longer leg of a right triangle is 2 cm more than the shorter leg. The length of the hypotenuse is 4 cm more than the shorter leg. Find the length of the hypotenuse. Show your work and be sure to assign a variable.
The length of the hypotenuse is 6 cm.
The length of the hypotenuse is 8 cm.
The length of the hypotenuse is 10 cm.
The length of the hypotenuse is 2 cm.
Math
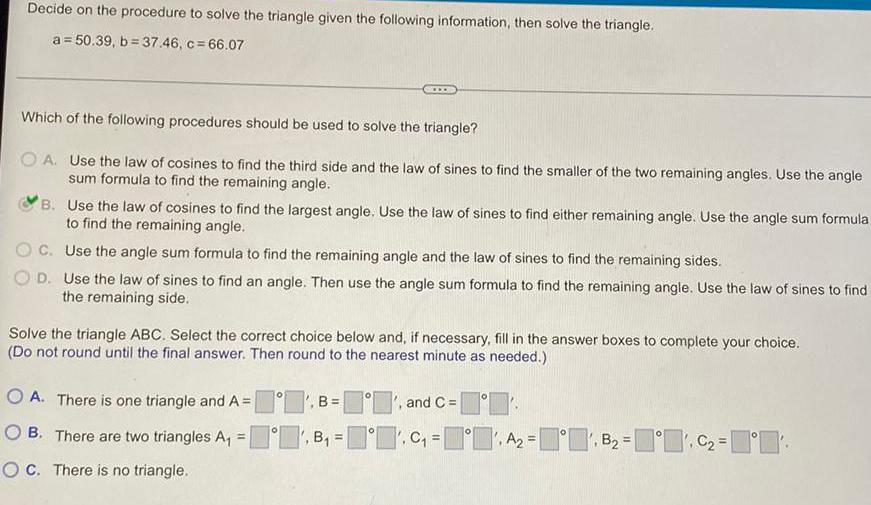
Solution of trianglesDecide on the procedure to solve the triangle given the following information, then solve the triangle.
a = 50.39, b = 37.46, c=66.07
Which of the following procedures should be used to solve the triangle?
A. Use the law of cosines to find the third side and the law of sines to find the smaller of the two remaining angles. Use the angle
sum formula to find the remaining angle.
B.Use the law of cosines to find the largest angle. Use the law of sines to find either remaining angle. Use the angle sum formula
to find the remaining angle.
C.Use the angle sum formula to find the remaining angle and the law of sines to find the remaining sides.
OD. Use the law of sines to find an angle. Then use the angle sum formula to find the remaining angle. Use the law of sines to find
the remaining side.
Solve the triangle ABC. Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice.
(Do not round until the final answer. Then round to the nearest minute as needed.)
OA. There is one triangle and A =
OB. There are two triangles A₁ =
OC. There is no triangle.
......
1
B=
B₁ =
and C=
1°₁ C₁ =
A₂ =
B₂=
}', C₂ = '.
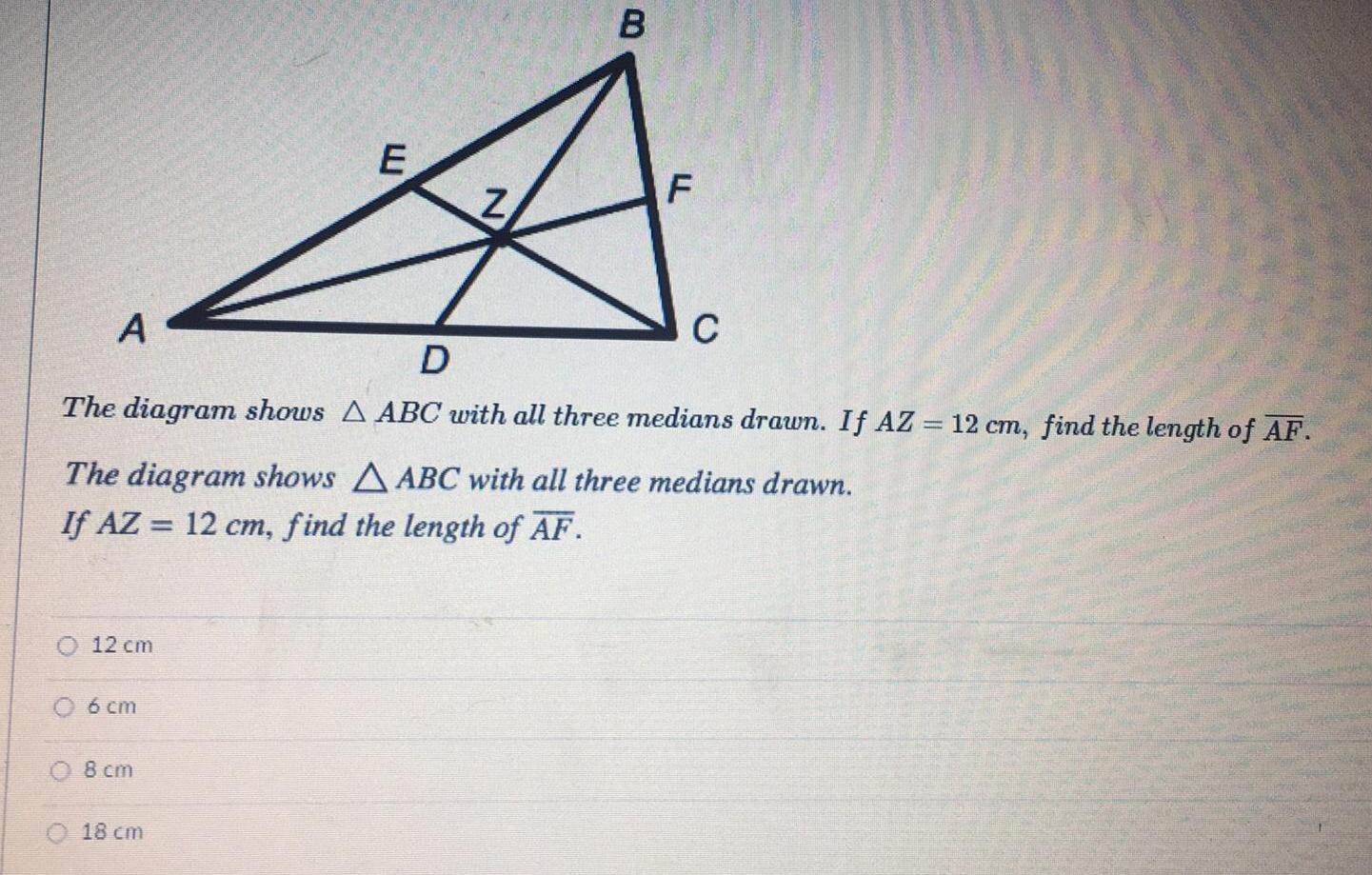
Math
Solution of trianglesThe diagram shows ΔABC with all three medians drawn.
If ΔZ = 12 cm, find the length of AF.
The diagram shows ΔABC with all three medians drawn. If AZ = 12 cm, find the length of AF.
a.12 cm
b. 6 cm
c. 8 cm
d. 18 cm
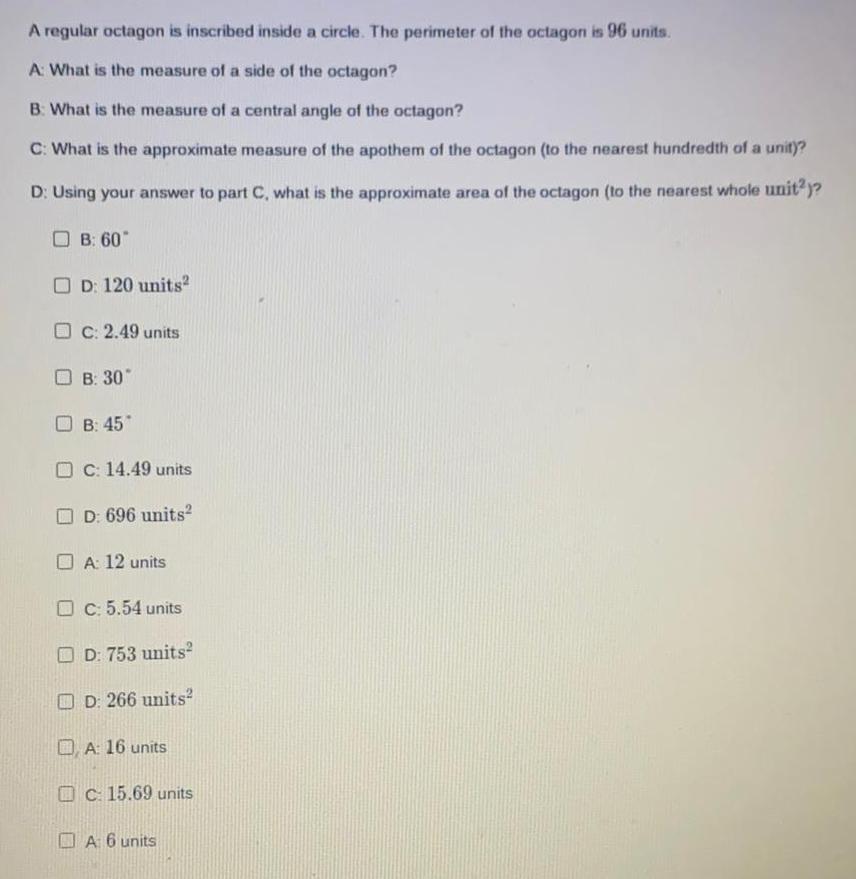
Math
Solution of trianglesA regular octagon is inscribed inside a circle. The perimeter of the octagon is 96 units.
A: What is the measure of a side of the octagon?
B: What is the measure of a central angle of the octagon?
C: What is the approximate measure of the apothem of the octagon (to the nearest hundredth of a unit)?
D: Using your answer to part C, what is the approximate area of the octagon (to the nearest whole unit²)?
B: 60*
D: 120 units²
C: 2.49 units
B: 30*
B: 45*
C: 14.49 units
D: 696 units²
A: 12 units
C: 5.54 units
D: 753 units²
D: 266 units²
A: 16 units
C: 15.69 units
A: 6 units
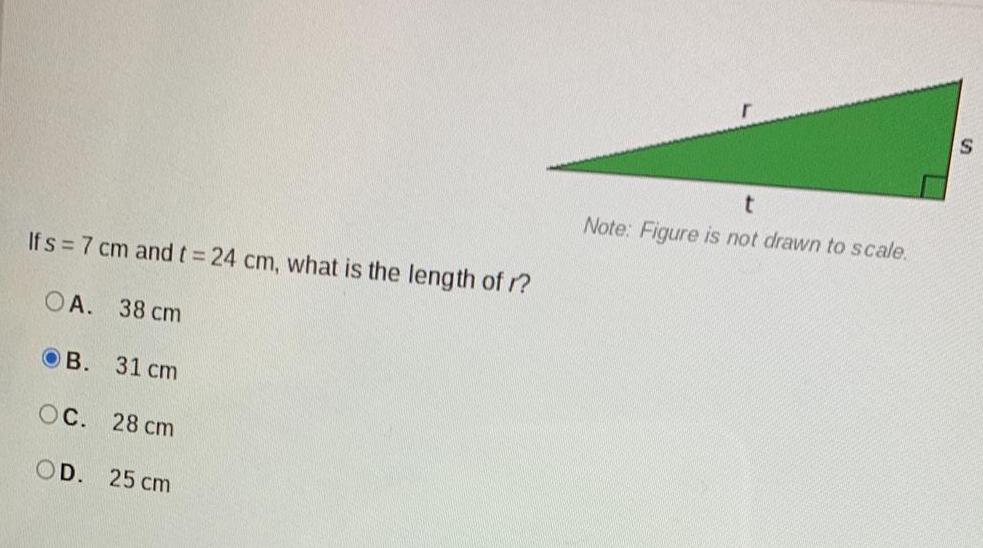
Math
Solution of trianglesIf s= 7 cm and t = 24 cm, what is the length of r?
A. 38 cm
B.31 cm
C. 28 cm
D. 25 cm
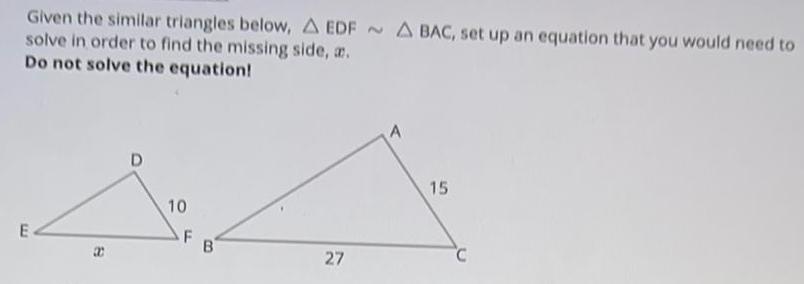
Math
Solution of trianglesGiven the similar triangles below, A EDF A BAC, set up an equation that you would need to solve in order to find the missing side, a. Do not solve the equation!
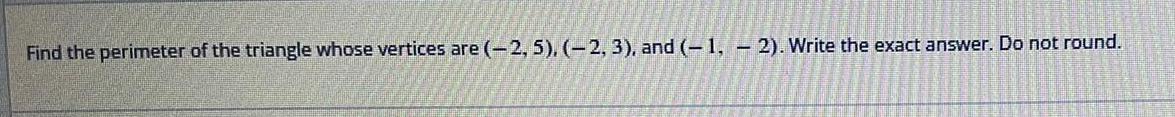
Math
Solution of trianglesFind the perimeter of the triangle whose vertices are (-2, 5), (-2, 3), and (-1, - 2). Write the exact answer. Do not round.

Math
Solution of trianglesOne angle in a triangle has a measure that is three times as large as the smallest angle. The measure of the third angle is 20 degrees more than that of the smallest angle. Find the measure of the LARGEST angle.
Answer: The LARGEST angle has a measure of degrees.
Math
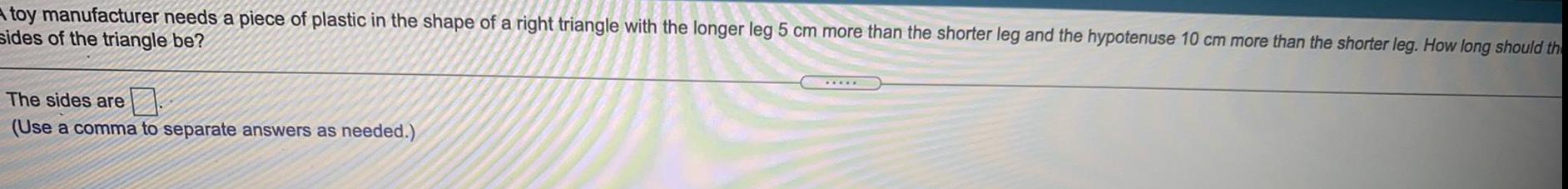
Solution of trianglestoy manufacturer needs a piece of plastic in the shape of a right triangle with the longer leg 5 cm more than the shorter leg and the hypotenuse 10 cm more than the shorter leg. How long should the sides of the triangle be?

Math
Solution of trianglesOne of the legs of a right triangle measures 3 cm and the other leg measures 5 cm Find the measure of the hypotenuse. If necessary, round to the nearest tenth.

Math
Solution of trianglesWhich point in a triangle is equidistant from the vertices of the triangle?
A. the point of intersection of the altitudes
B.the point of intersection of the medians
C.the point of intersection of the perpendicular bisectors
D.the point of intersection of the angle bisectors

Math
Solution of trianglesThe measure of the larger angle of an isosceles triangle is twenty more than two times the measure of each of the other two angles. Find the measure of the larger angle.

Math
Solution of trianglesFencing is used to enclose a rectangular parking lot. In addition, a fence is to be constructed in the middle of the lot, parallel to the shorter side,
dividing the parking lot into two sections. If 1,200 meters of fencing is available, what is the maximum area that can be enclosed?
square meters

Math
Solution of trianglesA farmer is building a triangular enclosure. He has already
built 2 sides. The first side is 500 yards and the second
one is 1200 yards, with a 36 degree angle between them.
What kind of a triangle is his enclosure?
Acute isosceles
Acute scalene
Right scalene
Obtuse scalene
Answer is not there

Math
Solution of trianglesFor the AABC, A(0,0), B(3, 0) and C(0, 4) then
distance between orthocentre and circumcentre of the A is 5
distance between orthocentre and circumcentre of the A is 5/2
distance between orthocentre and centroid of the A is 5/2
distance between orthocentre and centroid of the A is 5/3
Math
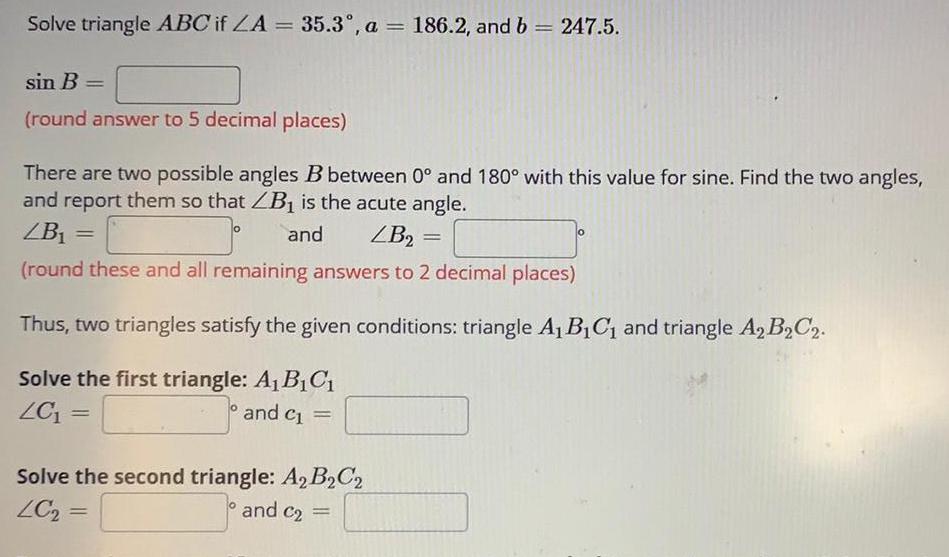
Solution of trianglesSolve triangle ABC if ZA = 35.3°, a = 186.2, and b = 247.5.
sin B =
(round answer to 5 decimal places)
There are two possible angles B between 0° and 180° with this value for sine. Find the two angles,
and report them so that ZB₁ is the acute angle.
ZB₁
and ZB₂
(round these and all remaining answers to 2 decimal places)
=
O
=
Thus, two triangles satisfy the given conditions: triangle A₁B₁C₁ and triangle A₂ B₂C₂.
Solve the first triangle: A₁B₁C₁
ZC₁ =
and C₁ =
Solve the second triangle: A₂ B2C2
ZC₂ =
and C₂ =

Math
Solution of trianglesThe Great Pyramid of Cheops has a square base with a length of 756 feet. Its height is 482 feet. If you
from the center of the north side to the top of the pyramid you have to climb an angle
degrees.
walk straight up
of
You decide to simplify your life and walk up along one of the ridges. Thus you have to climb only at an
angle of
degrees.
On your way up the ridge you walk a distance of
LO
feet.
Hint: For the first two parts draw right triangles and use an inverse trig function. For the third part just
use the Pythagorean Theorem.
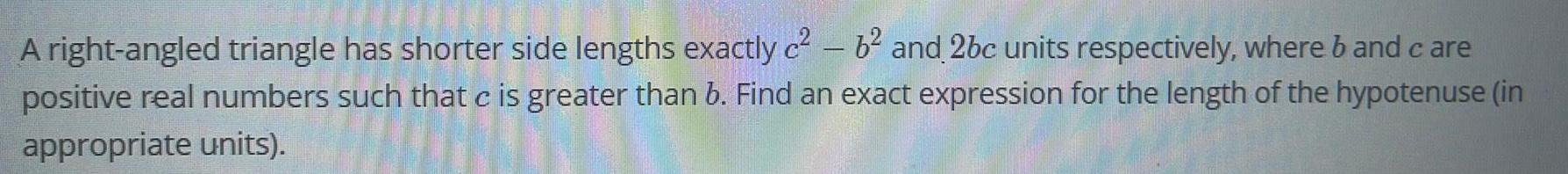
Math
Solution of trianglesA right-angled triangle has shorter side lengths exactly c² - 6² and 2bc units respectively, where b and c are positive real numbers such that c is greater than b. Find an exact expression for the length of the hypotenuse (in appropriate units).

Math
Solution of trianglesThe areas of similar triangles AABC and ADEF are equal. If the longest side of ADEF measures 25 units, what is the length of the longest side of ΔABCD
A 5 units
B. 25 units
.C. 50 units
D. 75 units

Math
Solution of trianglesThe Statue of Liberty is approximately 305 feet tall. If the angle of elevation of a ship to the top of the statue is 24.6°, how far, to the nearest foot, is the ship from the statue's base?
feet (Round to the nearest foot.)
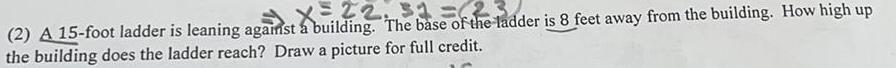
Math
Solution of trianglesA 15-foot ladder is leaning against a building. The base of the ladder is 8 feet away from the building. How high up the building does the ladder reach? Draw a picture for full credit.

Math
Solution of trianglesAirports A and B are 455 km apart, on an east-west line. Jim flies in a northeast direction from A to airport C. From C he flies 311 km on a bearing of 127°40' to B. How far is C from A? km. The distance between C and A is

Math
Solution of trianglesA Christmas tree is supported by a wire that is 1 foot longer than the height of the tree. The wire is anchored at a point whose distance from the base of the tree is 17 feet shorter than the height of the tree. What is the height of the tree?
Math
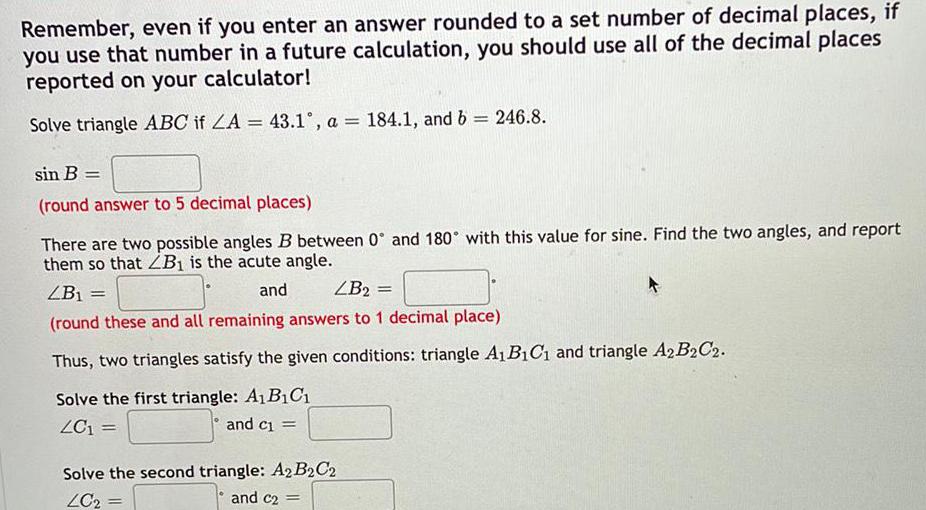
Solution of trianglesRemember, even if you enter an answer rounded to a set number of decimal places, if
you use that number in a future calculation, you should use all of the decimal places
reported on your calculator!
Solve triangle ABC if ZA = 43.1°, a = 184.1, and b = 246.8.
sin B
(round answer to 5 decimal places)
There are two possible angles B between 0 and 180° with this value for sine. Find the two angles, and report
them so that B1 is the acute angle.
LB₁ =
and
LB₂ =
(round these and all remaining answers to 1 decimal place)
Thus, two triangles satisfy the given conditions: triangle A₁B₁C₁ and triangle A2B2C2.
Solve the first triangle: A₁B1C₁
ZC1=
and c₁ =
=
=
Solve the second triangle: A2 B2C2
LC₂=
=
and c2
=
Math
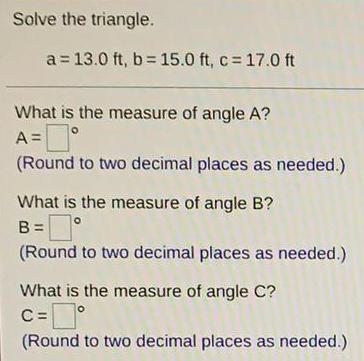
Solution of trianglesSolve the triangle.
a = 13.0 ft, b= 15.0 ft, c = 17.0 ft
What is the measure of angle A?
A =
(Round to two decimal places as needed.)
What is the measure of angle B?
B = °
(Round to two decimal places as needed.)
What is the measure of angle C?
C=
(Round to two decimal places as needed.)

Math
Solution of trianglesSolve the problem.
35) To find the distance AB across a river, a distance BC of 284 m is laid off on one side of the river. It is found that B =
113.7° and C= 13.1°. Find AB. Round to the nearest meter.