Quadratic equations Questions and Answers

For the following quadratic equation, find the discriminant. -2x² + 18x +42=-3x² - 2

Write the quadratic equation in standard form: -3x²+2x - 10 = -3x

An architect wants to draw a rectangle with a diagonal of 25 millimeters. The length of the rectangle is to be 3 millimeters more than triple the width. What dimensions should she make the rectangle?

What are the roots of the equation x² + 4x + 68 = 0 in simplest a + bi form?

Write the quadratic equation in standard form: 3x -4 = -x²

Fully simplify using only positive exponents. 125x6y3/25x²y6

Solve for all values of x by factoring. x² + 20x + 72 = 3x

Solve the following quadratic equation for all values of x in simplest form. 3(2x - 5)² 15 = 48

Hitting the ball into the parking lot As Juan continued his hitting practice showing off his abilities, one of the balls flew over the center field stands and into the parking lot. "Did you see that shot", he yelled at the girls. "The ball hung in the air for at least 10 seconds", he exclaimed. The formula for this hit is: h(x) = -16x² +83x+4 where h is the height of the ball and x is the number of seconds the ball is in the air. A. How can Juan provide proof that the ball actually hung in the air for 10 seconds? B. How long did the ball hang actually hang in the air?

A smart-phone is thrown upwards from the top of a 672-foot building with an initial velocity of 16 feet per second. The height h of the smart-phone after t seconds is given by the quadratic equation h=16t² + 16t+672. When will the smart-phone hit the ground? seconds

Solve by the quadratic formula: 3x² + 10x 25 = 0 If you have two rational solutions, list them separated by commas, for example: "2/3,5/2" If you have two irrationl solutions, type 5+2√3 5 as "(5+-2sqrt(3))/5" 25 3' 2 type

Consider the function f(x) = x³ + 6x² + 11x + 6. If f(x) = 0 for x = -3, for what other values of x is the function equal to 0? List the values separated by commas. Do not include the zero x = -3 in your answer.

If an object is projected from a height of 112 ft with an initial velocity of 96 ft per sec, its height after t seconds is given by the following equation. h=-16t² +961 + 112 Use this information to answer parts (a) and (b) below.

Consider the function. f(x) = 2x³ - 6x² + 48x Find all values of x that correspond to horizontal tangent lines. Select "None" if the function does not have any values of x that correspond to horizontal tangent lines.

If coefficients of biquadratic equation are all distinct and belong to the set {-9, - 5, 3, 4, 7}, then equation has (A) atleast two real roots (B) four real roots, two are conjugate surds and other two are also conjugate surds (C) four imaginary roots (D) None of these

A rocket is shot from an underground bunker. The height of the rocket after t seconds is given by y = - 161² + 1761-384 (measured in feet relative to the surface). Determine how long after the rocket is launched that it will emerge from the ground and determine how much longer it will be for the rocket to hit the ground. O It will take 3 seconds to emerge and another 8 seconds to hit the ground. It will take 5 seconds to emerge and another 3 seconds to hit the ground. It will take 3 seconds to emerge and another 5 seconds to hit the ground. It will take 5 seconds to emerge and another 8 seconds to hit the ground.

Find all complex (real and non-real) solutions of 2x³ 3x² + 32x + 17 = 0 Provide your answer below: - Give your answer as comma separated values, with the complex solutions in the form a +bi. Do not use signs, instead include them as separate values. Do not include x = in your answer. For example, if your solutions are 1 and 1 ± 2i, you would enter 1, 1+2i, 1 - 2i.

Consider the following quadratic equation: -4y² - 2y + 1 = 0 Step 2 of 2: Use the discriminant, b² 4ac, to determine the number of solutions of the given quadratic equation. Then solve the quadratic equation using th - b ± √√√b² - 4ac formula y = 2a Answer Select the number and type of solutions. Then, enter the solutions. Selecting an option will display any text boxes needed to complete your answer. OTwo different real solutions O One repeated real solution O Two non-real solutions Ke Keyboard She Previous Step Ar

The polynomial f(x) given below has 1 as a zero. Find all the other zeros of f(x). Provide your answer below: ƒ(x) = x³ − 3x² + 4x − 2

Which of the following are odd functions? Select all correct answers. Select all that apply: ƒ(x) = −9x ƒ(x) = x² − x² + 5 f(x) = 2x³ + x f(x) = 3x³ + 3x f(x) = -x² – 3x – 1

One factor of the function f(x) = x3 - 8x² + 17x - 10 is (x - 5). Describe how to find the x-intercepts and the y-intercept of the graph of f(x) without using technology. Show your work and include all intercepts in your answer.

Rewrite the following equation in standard form. f(x) = (2x + 5)(3x – 5) a f(x) = 6x²5x - 25 b f(x) = 6x² + 5x – 25 c f(x) = 6x² + 25x – 25 d f(x) = 5x² + 11x - 25

The graph of y = 5x6 - 3x4 +2x-9 has at most how many turning points? Choose the correct answer below.

Solve for w. 3w²2² +5=-8w If there is more than one solution, separate them with commas. If there is no solution, click on "No solution."

Use the long division method to find the result when 8x3³ +22x² + 29x + 21 is divided by 2x + 3.

Find all the zeros of the quadratic function. y=x²-2x-24 If there is more than one zero, separate them with commas. If there are no zeros, click on "None".

Solve for u. (u+6)² =2u² +9u+26 If there is more than one solution, separate them with commas.

The flight of a kicked football follows the quadratic function f(x) = -0.02x² +2.2x +2, where f(x) is the vertical distance in feet and is the horizontal distance the ball travels. How far, in feet, will the ball travel across the field by the time it hits the ground? Round your answer to one decimal place.

Watch the video that describes using the zero-product property with one solution. Click here to watch the video. Solve the following equation. For an equation with real solutions, support your answer graphically. 64s² +80s +25=0

Solve (x-5)2²-20=0, where x is a real number. Simplify your answer as much as possible. If there is more than one solution, separate them with commas. If there is no solution, click "No solution."

Solve the equation. For an equation with real solutions, support the answer graphically. 5x² - 6x=0 Rewrite the equation in factored form. _________=0 (Factor completely.)

For the quadratic function defined, (a) write the function in the form P(x)= a(x-h)2+k, (b) give the vertex of the parabola, and (c) graph the function. P(x)=x²-3x+3

Watch the video that describes completing the square. For the quadratic function defined, write the function in the form P(x)= a(x-h)² + k. P(x)=x² - 6x-4

For the following quadratic function, (a) use the vertex formula to find the coordinates of the vertex and (b) graph the function. P(x)=x² - 4x +3 The vertex is (Type an ordered pair.)

3. Graph the following equation by finding their intercepts. If an equation cannot be graphed using the intercepts, graph it by finding other points on the line. Use a third point on each graph to check your answers. a) x + 6y = 6 b) 5x + 2y = -10 c) X 100 8 + y = 1 5 X y d) 1/3-²/3 = 1 2 e) 7x - 7y=0 f) 7x - 7=0

Determine whether the function's verlex is a maximum point or a minimum point. (1/2)x²+x=y=5 The vertex is a maximum point. The vertex is a minimum point. Find the coordinates of this point. (x, y) = Find the zeros, if any exist. (Enter your answers as a comma-separated list. If an answer does not exist, enter DNE.) x = Find the y-Intercept. y =

Use Descartes' rule of signs to determine the total number of real zeros and the number of positive and negative real zeros. (Hint: First factor out x to its lowest power.) f(x) = 5x11+ 2x9 + 9x7 - 9x6 Select one: a. 7 real zeros; f(x) has 1 positive real zero, no negative real zeros and the number 0 is a zero of multiplicity 6. b. 2 real zeros; f(x) has 1 positive real zero, no negative real zeros and the number 0 is a zero of multiplicity 1. c. 11 real zeros; f(x) has 1 positive real zero, 4 negative real zeros and the number 0 is a zero of multiplicity 6. d. 6 real zeros; f(x) has 1 positive real zero, no negative real zeros and the number 0 is a zero of multiplicity 5.

Jason and his team were working on finding the points of intersection of f(x) = 2x² -5x + 6 and g(x) = 2x²-x+ 30. He suggested, "Maybe we could start by looking at the graphs of the functions." a. Use your graphing calculator to help you graph f(x) and g(x). b. Adjust the viewing window so that you can see all of the points of intersection. How accurately can you approximate the coordinates of these points by looking at the graph? Give it a try. c. Use the "Trace" feature to get a more accurate approximation of each of the points. d. With your team, explore the [CALC] feature of your TI-83/84+ graphing calculator. Can you find a way to make the graphing calculator calculate your points of intersection for you? How accurate are your results? Be prepared to share your method to the class.

Graph the equation y = -x² + 6x - 8 on the accompanying set of axes. You must plot 5 points including the roots and the vertex. Using the graph, determine the roots of the equation -x² + 6x - 8 = 0.

Put the equation y = x² + 14x + 40 into the form y.= (x - h)² + k: Answer: y =

Consider the parabola given by the equation: f(x) = - 2x² + 4x + 15 Answer the following for this parabola: A) The graph of this function opens: Select an answer B) Give the domain of the function using interval notation: C) Give the range of the function using interval notation:

Solve the equation 2 = - 4s2 - 9s State your answers as integers or reduced fractions, separated by commas.

If the discriminant of a quadratic equation is -2, then the equation has If the discriminant of a quadratic equation is 2, then the equation has If the discriminant of a quadratic equation is 4, then the equation has If the discriminant of a quadratic equation is 0, then the equation has

Which statements are true about exponential functions and quadratic functions? Check all that apply. Quadratic functions both decrease and increase for different values of X. Equations of both quadratic and exponential functions have exponents containing the variable. Graphs of exponential function are curves. Equations of exponential functions have a variable in the exponent. OQuadratic functions are always decreasing, or always increasing, as increases.

While warming up for a game, two basketball players shoot balls in parabolic paths. The path of the first player's ball can be represented by the function g(x) = -2x² + 9x + 5, where x represents the distance from the coach. The path of the second player's ball can be represented by the function h(x) = -x² + 3x + 10. Part A: Find the distances in which the two basketball paths will cross. Show all necessary calculations. (4 points) Part B: Let f(x)=g(x)/h(x) Solve for f(x) in simplest form and determine all discontinuities. (6 points)

Plot the given parabola on the axes. Plot the roots, the vertex and two other points. -10 y = 2x² + 246x + 6804

Consider the quadratic equation 4x² - 11x - 3= 0. Which of the following shows this equation rewritten and ready to solve using factoring by grouping? A) 4x² - 9x-2x - 3 = 0 B) 4x² - 12x + x - 3=0 C) 4x² + 12x-x-3=0 D) 4x² - 6x-5x-3 = 0

Write the equation of the quadratic function whose graph is a parabola containing the points (10,84), (0, -1), and (-5,31.5). y=(Use integers or decimals for any numbers in the expression.)

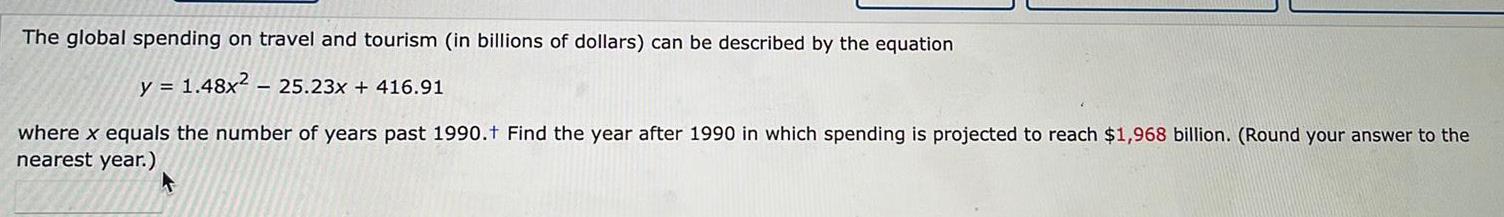
The global spending on travel and tourism (in billions of dollars) can be described by the equation y = 1.48x²- 25.23x + 416.91 where x equals the number of years past 1990.+ Find the year after 1990 in which spending is projected to reach $1,968 billion. (Round your answer to the nearest year.)

Using data from 2002 and with projections to 2024, total annual expenditures for national health care (in billions of dollars) can be described by E = 4.61x² + 43.4x + 1620 where x is the number of years past 2000.+ If the pattern indicated by the model remains valid, in what year does the model predict these expenditures will reach $7,071 billion?